I have this function
$$ f(x)=\frac{1-25 x^2}{\left| 25 x^2-1\right| }\;\frac{2500 x}{\sqrt{\left(25 x^2-1\right)^2 \sinh (\pi x)+9}}+\cosh ^{-1}\left(\frac{1000 x^2+\left(25 x^2-1\right)^2}{\left(25 x^2+1\right)^2}\right) $$
f[x_] := ArcCosh[(1000 x^2 + (-1 + 25 x^2)^2)/(1 + 25 x^2)^2] + (
1 - 25 x^2)/Abs[-1 + 25 x^2] (2500 x )/ Sqrt[
9 + (-1 + 25 x^2)^2 Sinh[π x]];
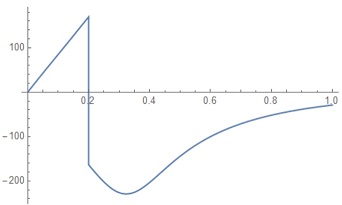
for $0<x<5$. I plot this function and I get
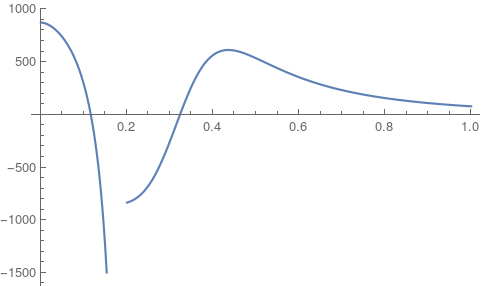
As can be seen, for $0.2<x<1$, function is decreasing first and then increasing. Therefore $f'(x)$ must be negative and positive. Also, $f'(x)$ must be zero at one point. But when I use RegionPlot to see where $f'(x)$ is negative, it says nowhere. Also, I try to Plot $f'(x)$, but I get no answer. Does someone know where I am making mistake?
RegionPlot[a (f'[x]) < 0, {x, 0.2, 1}, {a, 0, 1}]
Plot[f'[x], {x, 0.2, 1}]
ContourPlot[a (f'[x]) == 0, {x, 0, 1}, {a, 0, 1}]