Here the answer for @Luiz Roberto Meier, which was closed although it was no duplicate, but needed some deeper reflections.
First, ContourPlot doesn't show the complete solution.
This plot is complex-valued, therefore you may use Re and Im
So, the extraction of the plotpoints in ContourPlot won't be very useful, but as already suggested it might be done of course using Cases:
points = ContourPlot[{y^4 + 6 x^3*y + x^8 == 0},
{x, -5, 5}, {y, -5, 5}] // Normal // Cases[#, Line[$_] -> $, Infinity] &
ListPlot[points, Joined -> True, AspectRatio -> 1, PlotRange -> 5]
Basically the two functions can be solved by
Clear@"`*"
f[x_] := x^3
g[x_] := Block[{y}, y /. Solve[{y^4 + 6 x^3*y + x^8 == 0 }, y]]
Plot[{f[x], g[x]}, {x, -5, 5}, PlotRange -> 5, AspectRatio -> 1]
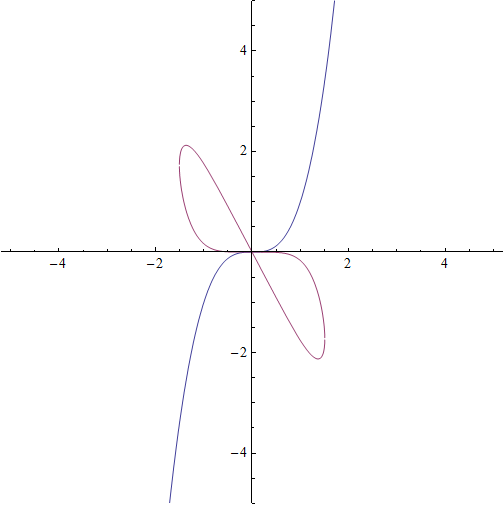
f is easy, so we concentrate on g from now on.
Inspecting g we find that it has 4 complex solutions in y, (order 4)
Plotting g using Plot having a look at Re and Im gives:
Clear@"`*"
g[x_] := Block[{y}, y /. Solve[{y^4 + 6 x^3*y + x^8 == 0 }, y]]
Plot[{g[x][[1]], g[x][[2]], g[x][[3]], g[x][[4]]} // Re //
Evaluate, {x, -5, 5}, PlotRange -> 5, AspectRatio -> 1]
Plot[{g[x][[1]], g[x][[2]], g[x][[3]], g[x][[4]]} // Im //
Evaluate, {x, -5, 5}, PlotRange -> 5, AspectRatio -> 1]
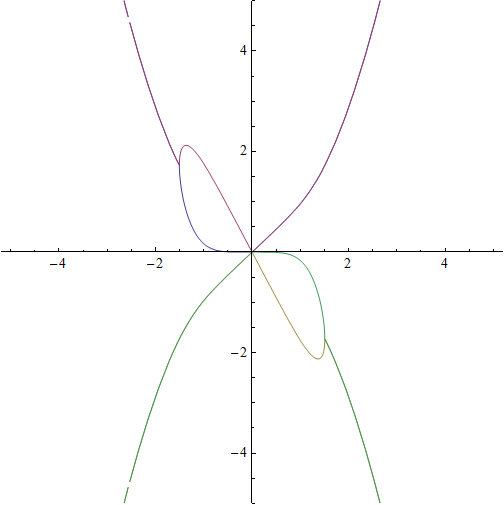
Let's say, we are interested in the real part.
In the plot we see that we are missing some points for x near -2.5
As we are interested in a complete point list, we try increasing option values like MaxRecursions or PlotPoints (without success).
Changing strategy using ListPlot, where we can increment ourself the x-Range provides
a high quality solution and a complete set of {x,y} point coordinates by analytical means:
Clear@"`*"
g[x_] := Block[{y}, y /. Solve[{y^4 + 6 x^3*y + x^8 == 0 }, y]]
sol1 = Re@{#, g[#][[1]]} & /@ Range[-5, 5, .005];
sol2 = Re@{#, g[#][[2]]} & /@ Range[-5, 5, .005];
sol3 = Re@{#, g[#][[3]]} & /@ Range[-5, 5, .005];
sol4 = Re@{#, g[#][[4]]} & /@ Range[-5, 5, .005];
ListPlot[#, Joined -> True, PlotRange -> 5,
AspectRatio -> 1] & /@ {sol1, sol2, sol3, sol4}
ListPlot[#, Joined -> True, PlotRange -> 5, AspectRatio -> 1] &@{sol1,
sol2, sol3, sol4}
Of course it would be possible to map over the 4 solutions at once but for the sake of explanation I didn't.
the first image shows the solutions separated,
the next image combines them.
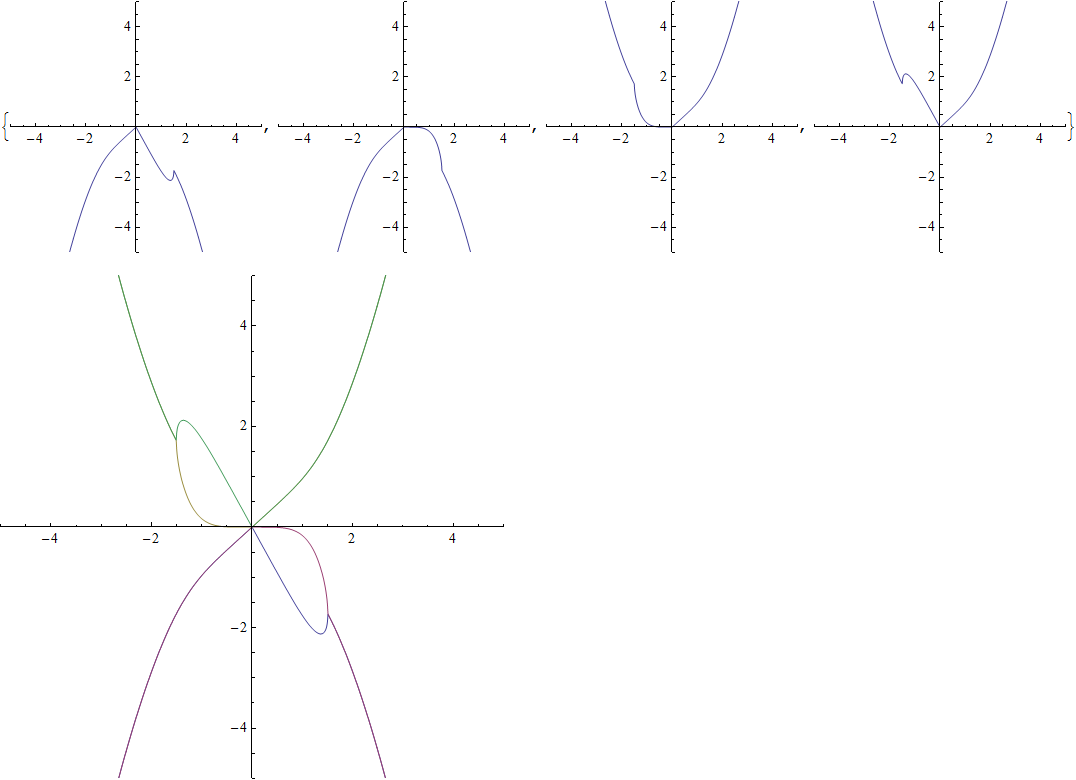
all points for the complete real solution you were looking for g[x], are now in:
{ sol1, sol2, sol3, sol4 }

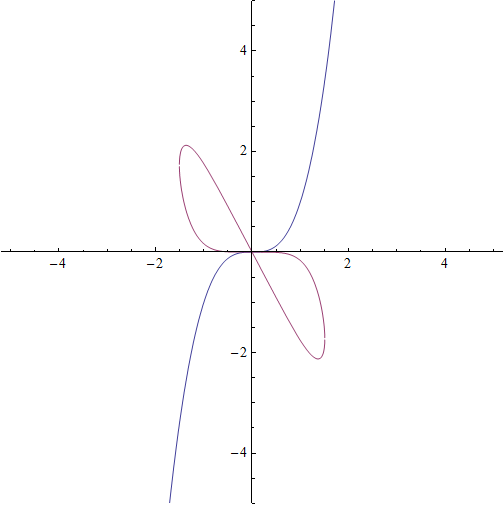
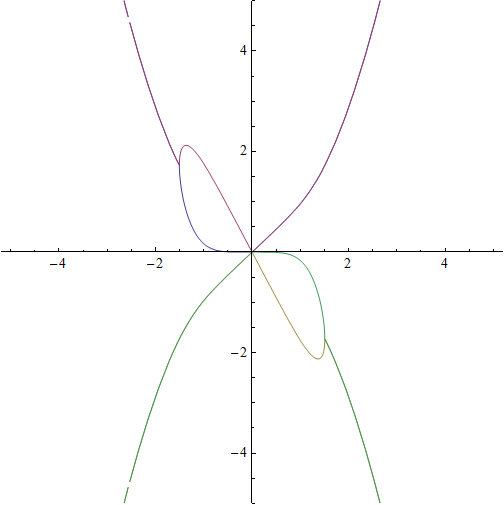

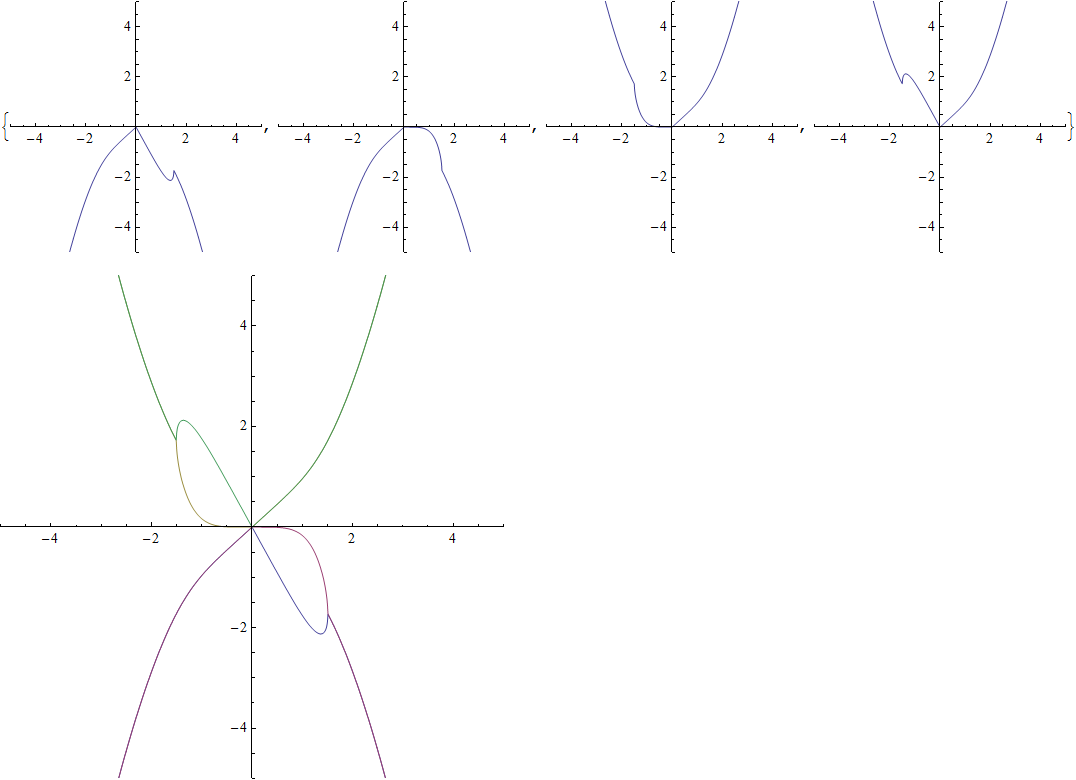
RegionPlotoutput. $\endgroup$