ListPlot3D. Only plots about half of the data in a 3D matrix

Question

Here is the data and the exact command I'm trying to run.

I've had success with other datasets generated and written out to a file by the exact same program in the exact same manner but for some reason this one won't plot correctly. The only thing I can think of is that it has something to do with the large ranges of the numbers. I can't find any missing commas or otherwise incorrect syntax, however I am very new to mathematica so its likely something very simple I'm overlooking. Played around with PlotRange to no avail. Any Ideas?

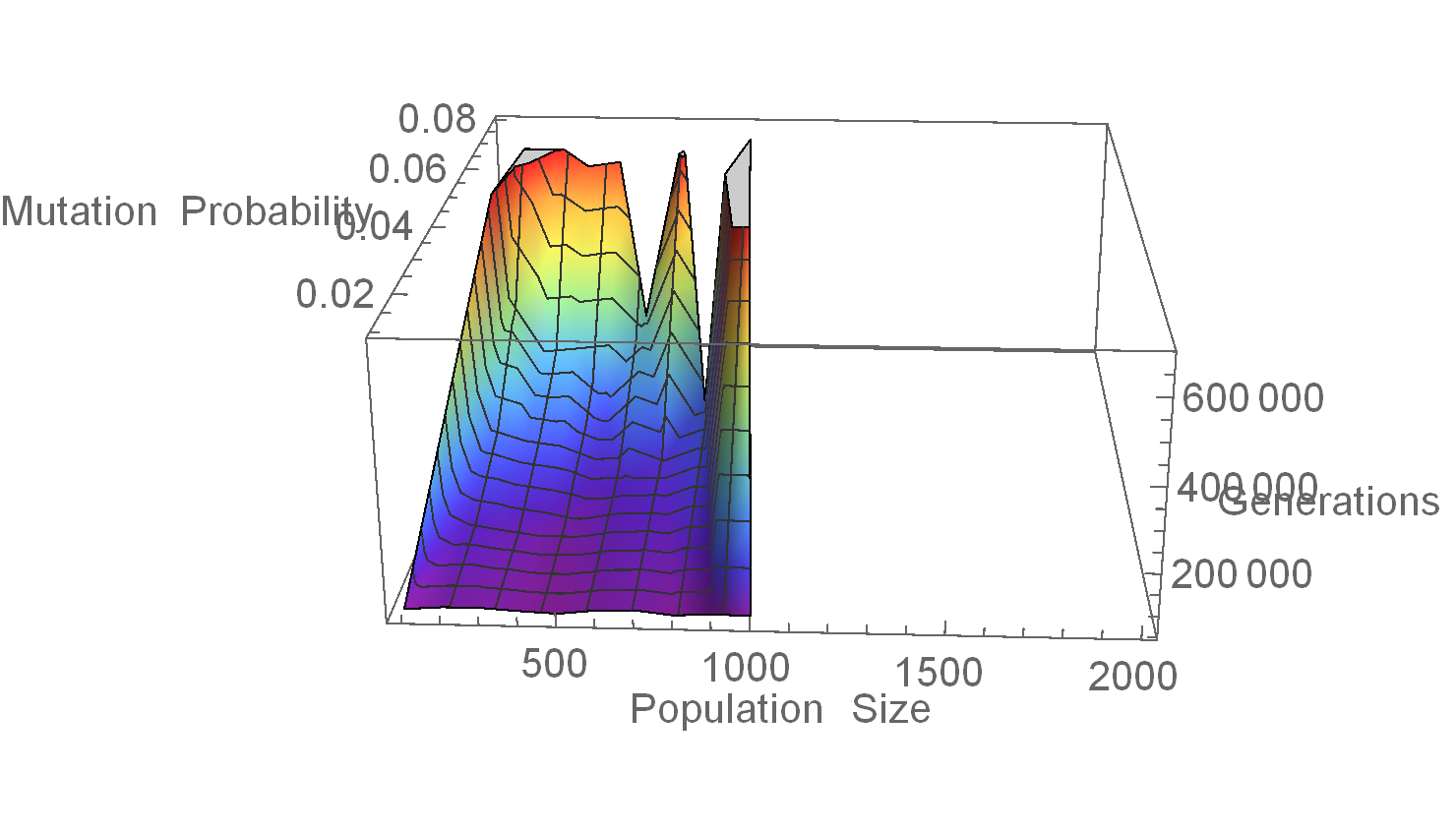
enter image description here

ListPlot3D[{{100, 0.01, 60510.1}, {100, 0.015, 70971.5}, {100, 0.02, 
   90358.1}, {100, 0.025, 107710}, {100, 0.03, 155191}, {100, 0.035, 
   176332}, {100, 0.04, 268286}, {100, 0.045, 387221}, {100, 0.05, 
   446528}, {100, 0.055, 616056}, {100, 0.06, 840413}, {100, 0.065, 
   1.37393*10^6}, {100, 0.07, 1.36754*10^6}, {100, 0.075, 
   2.77176*10^6}, {100, 0.08, 2.426*10^6}, {200, 0.01, 66869.9}, {200,
    0.015, 71399.5}, {200, 0.02, 74329.3}, {200, 0.025, 
   99582.5}, {200, 0.03, 121926}, {200, 0.035, 166560}, {200, 0.04, 
   186512}, {200, 0.045, 207654}, {200, 0.05, 256898}, {200, 0.055, 
   398627}, {200, 0.06, 466594}, {200, 0.065, 662348}, {200, 0.07, 
   933421}, {200, 0.075, 1.11269*10^6}, {200, 0.08, 
   1.36385*10^6}, {300, 0.01, 67070.1}, {300, 0.015, 65805.3}, {300, 
   0.02, 80248.1}, {300, 0.025, 85741.4}, {300, 0.03, 96002.1}, {300, 
   0.035, 120096}, {300, 0.04, 159605}, {300, 0.045, 170339}, {300, 
   0.05, 228897}, {300, 0.055, 256947}, {300, 0.06, 345085}, {300, 
   0.065, 450238}, {300, 0.07, 700978}, {300, 0.075, 832152}, {300, 
   0.08, 1.32654*10^6}, {400, 0.01, 61246.9}, {400, 0.015, 
   66118}, {400, 0.02, 68661.2}, {400, 0.025, 73570.8}, {400, 0.03, 
   92025.9}, {400, 0.035, 109598}, {400, 0.04, 147937}, {400, 0.045, 
   161330}, {400, 0.05, 224650}, {400, 0.055, 266217}, {400, 0.06, 
   310519}, {400, 0.065, 447822}, {400, 0.07, 641469}, {400, 0.075, 
   806530}, {400, 0.08, 1.37741*10^6}, {500, 0.01, 56989.3}, {500, 
   0.015, 67928.9}, {500, 0.02, 69761.9}, {500, 0.025, 86710.9}, {500,
    0.03, 79849}, {500, 0.035, 97954.5}, {500, 0.04, 127872}, {500, 
   0.045, 139649}, {500, 0.05, 150587}, {500, 0.055, 275188}, {500, 
   0.06, 305953}, {500, 0.065, 426897}, {500, 0.07, 654867}, {500, 
   0.075, 966621}, {500, 0.08, 1.36171*10^6}, {600, 0.01, 
   66711.9}, {600, 0.015, 65131.8}, {600, 0.02, 73614.3}, {600, 0.025,
    78866}, {600, 0.03, 81276.7}, {600, 0.035, 102292}, {600, 0.04, 
   107857}, {600, 0.045, 139988}, {600, 0.05, 189010}, {600, 0.055, 
   260216}, {600, 0.06, 315841}, {600, 0.065, 440656}, {600, 0.07, 
   662428}, {600, 0.075, 985202}, {600, 0.08, 1.51782*10^6}, {700, 
   0.01, 69192.1}, {700, 0.015, 66052.4}, {700, 0.02, 72424.6}, {700, 
   0.025, 82281.8}, {700, 0.03, 86213}, {700, 0.035, 94029.4}, {700, 
   0.04, 114066}, {700, 0.045, 140307}, {700, 0.05, 182638}, {700, 
   0.055, 235287}, {700, 0.06, 330950}, {700, 0.065, 474889}, {700, 
   0.07, 735568}, {700, 0.075, 1.08146*10^6}, {700, 0.08, 
   1.66039*10^6}, {800, 0.01, 58843.3}, {800, 0.015, 64994.5}, {800, 
   0.02, 67298.5}, {800, 0.025, 74371.7}, {800, 0.03, 80555.1}, {800, 
   0.035, 99640.4}, {800, 0.04, 104818}, {800, 0.045, 143354}, {800, 
   0.05, 177583}, {800, 0.055, 245006}, {800, 0.06, 338566}, {800, 
   0.065, 499601}, {800, 0.07, 732425}, {800, 0.075, 
   1.17749*10^6}, {800, 0.08, 1.88361*10^6}, {900, 0.01, 
   63246.3}, {900, 0.015, 64967.4}, {900, 0.02, 67008.1}, {900, 0.025,
    79926.8}, {900, 0.03, 77706.3}, {900, 0.035, 96751.9}, {900, 0.04,
    123840}, {900, 0.045, 133210}, {900, 0.05, 178143}, {900, 0.055, 
   254075}, {900, 0.06, 355435}, {900, 0.065, 504921}, {900, 0.07, 
   756158}, {900, 0.075, 1.29332*10^6}, {900, 0.08, 
   2.01113*10^6}, {1000, 0.01, 61433.1}, {1000, 0.015, 
   63099.3}, {1000, 0.02, 66182.8}, {1000, 0.025, 72844.6}, {1000, 
   0.03, 85995.2}, {1000, 0.035, 103902}, {1000, 0.04, 109085}, {1000,
    0.045, 138993}, {1000, 0.05, 189084}, {1000, 0.055, 
   257315}, {1000, 0.06, 396645}, {1000, 0.065, 539882}, {1000, 0.07, 
   778581}, {1000, 0.075, 1.40995*10^6}, {1000, 0.08, 
   2.23897*10^6}, {1100, 0.01, 60104.5}, {1100, 0.015, 
   64439.1}, {1100, 0.02, 72467.6}, {1100, 0.025, 76612.4}, {1100, 
   0.03, 84767.1}, {1100, 0.035, 106206}, {1100, 0.04, 122682}, {1100,
    0.045, 152169}, {1100, 0.05, 198745}, {1100, 0.055, 
   259857}, {1100, 0.06, 388296}, {1100, 0.065, 564835}, {1100, 0.07, 
   899551}, {1100, 0.075, 1.46177*10^6}, {1100, 0.08, 
   2.31448*10^6}, {1200, 0.01, 58711.3}, {1200, 0.015, 
   62742.4}, {1200, 0.02, 64179.7}, {1200, 0.025, 73288.1}, {1200, 
   0.03, 85146.1}, {1200, 0.035, 96879.7}, {1200, 0.04, 
   127671}, {1200, 0.045, 154304}, {1200, 0.05, 201372}, {1200, 0.055,
    278887}, {1200, 0.06, 416279}, {1200, 0.065, 622232}, {1200, 0.07,
    962591}, {1200, 0.075, 1.54832*10^6}, {1200, 0.08, 
   2.50081*10^6}, {1300, 0.01, 58959.4}, {1300, 0.015, 
   59761.6}, {1300, 0.02, 69075.9}, {1300, 0.025, 70665.1}, {1300, 
   0.03, 87007.1}, {1300, 0.035, 105193}, {1300, 0.04, 124706}, {1300,
    0.045, 163178}, {1300, 0.05, 213193}, {1300, 0.055, 
   300242}, {1300, 0.06, 432419}, {1300, 0.065, 651916}, {1300, 0.07, 
   982562}, {1300, 0.075, 1.67169*10^6}, {1300, 0.08, 
   2.57347*10^6}, {1400, 0.01, 57704.8}, {1400, 0.015, 
   58191.2}, {1400, 0.02, 66720.6}, {1400, 0.025, 79106.7}, {1400, 
   0.03, 88716.8}, {1400, 0.035, 102867}, {1400, 0.04, 126105}, {1400,
    0.045, 165217}, {1400, 0.05, 222163}, {1400, 0.055, 
   316881}, {1400, 0.06, 460439}, {1400, 0.065, 660282}, {1400, 0.07, 
   1.14617*10^6}, {1400, 0.075, 1.69754*10^6}, {1400, 0.08, 
   2.6663*10^6}, {1500, 0.01, 59764.7}, {1500, 0.015, 62215.2}, {1500,
    0.02, 65577.2}, {1500, 0.025, 76682.2}, {1500, 0.03, 
   86029.3}, {1500, 0.035, 112143}, {1500, 0.04, 137974}, {1500, 
   0.045, 172846}, {1500, 0.05, 239260}, {1500, 0.055, 341533}, {1500,
    0.06, 485092}, {1500, 0.065, 753040}, {1500, 0.07, 
   1.16581*10^6}, {1500, 0.075, 1.86748*10^6}, {1500, 0.08, 
   3.00517*10^6}, {1600, 0.01, 62543.2}, {1600, 0.015, 
   64851.5}, {1600, 0.02, 65456.3}, {1600, 0.025, 78520.7}, {1600, 
   0.03, 87927.6}, {1600, 0.035, 109371}, {1600, 0.04, 137308}, {1600,
    0.045, 181338}, {1600, 0.05, 247086}, {1600, 0.055, 
   362190}, {1600, 0.06, 500233}, {1600, 0.065, 756103}, {1600, 0.07, 
   1.17895*10^6}, {1600, 0.075, 1.94942*10^6}, {1600, 0.08, 
   2.93227*10^6}, {1700, 0.01, 60449.8}, {1700, 0.015, 
   63595.8}, {1700, 0.02, 65236.4}, {1700, 0.025, 77964}, {1700, 0.03,
    92225.1}, {1700, 0.035, 112889}, {1700, 0.04, 142803}, {1700, 
   0.045, 184930}, {1700, 0.05, 269727}, {1700, 0.055, 362958}, {1700,
    0.06, 540865}, {1700, 0.065, 780493}, {1700, 0.07, 
   1.26828*10^6}, {1700, 0.075, 2.0631*10^6}, {1700, 0.08, 
   3.2993*10^6}, {1800, 0.01, 58425.4}, {1800, 0.015, 62231.2}, {1800,
    0.02, 69519.5}, {1800, 0.025, 80873.6}, {1800, 0.03, 
   98030.4}, {1800, 0.035, 115355}, {1800, 0.04, 147563}, {1800, 
   0.045, 203516}, {1800, 0.05, 268480}, {1800, 0.055, 390830}, {1800,
    0.06, 567131}, {1800, 0.065, 885360}, {1800, 0.07, 
   1.34411*10^6}, {1800, 0.075, 2.13143*10^6}, {1800, 0.08, 
   3.22582*10^6}, {1900, 0.01, 57286.1}, {1900, 0.015, 
   63730.3}, {1900, 0.02, 72297.1}, {1900, 0.025, 81728.8}, {1900, 
   0.03, 99080.2}, {1900, 0.035, 123071}, {1900, 0.04, 152708}, {1900,
    0.045, 205180}, {1900, 0.05, 273948}, {1900, 0.055, 
   403890}, {1900, 0.06, 591449}, {1900, 0.065, 917536}, {1900, 0.07, 
   1.3526*10^6}, {1900, 0.075, 2.21305*10^6}, {1900, 0.08, 
   3.38519*10^6}, {2000, 0.01, 59740.3}, {2000, 0.015, 
   65435.7}, {2000, 0.02, 73929.5}, {2000, 0.025, 90775.2}, {2000, 
   0.03, 100335}, {2000, 0.035, 124159}, {2000, 0.04, 159627}, {2000, 
   0.045, 214404}, {2000, 0.05, 300085}, {2000, 0.055, 422780}, {2000,
    0.06, 602504}, {2000, 0.065, 924957}, {2000, 0.07, 
   1.44754*10^6}, {2000, 0.075, 2.29632*10^6}, {2000, 0.08, 
   3.55013*10^6}}, 
 AxesLabel -> {Population Size, Mutation Probability, Generations}, 
 ColorFunction -> "Rainbow"]

works fine for me in V9 but get the same problem in V10. On my way out so cannot investigate — Mike Honeychurch, Commented Jan 22, 2015 at 6:24
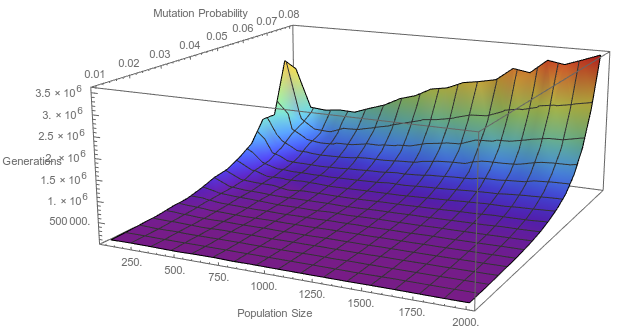
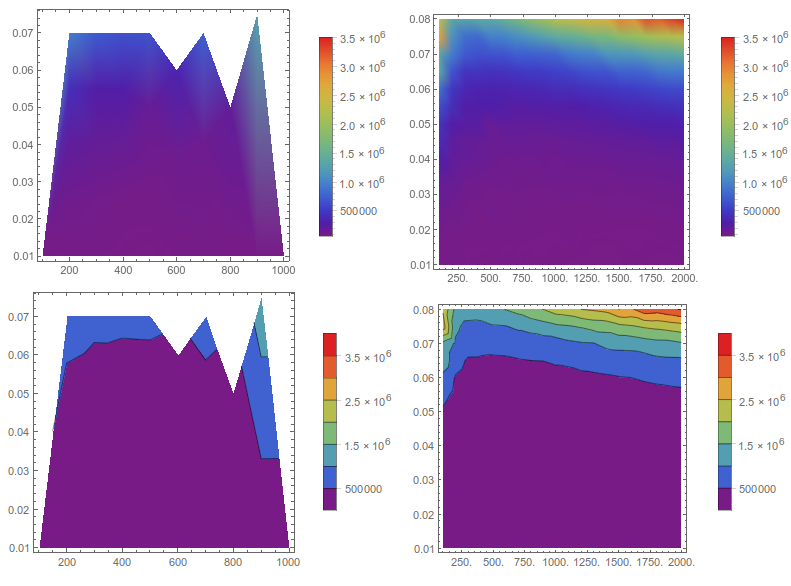
That's really odd. I can't find the problem, but here's a workaround for the time being: ListPlot3D[Transpose@Partition[Last /@ data, 15], DataRange -> {{100, 2000}, {0.01, 0.08}}, PlotRange -> All, ColorFunction -> "Rainbow"] i.sstatic.net/mwQmc.png — user484, Commented Jan 22, 2015 at 6:52
ListPointPlot3D applied to the original data works properly, although it does not have the desired appearance. Interestingly, setting InterpolationOrder -> 0 in ListPlot3D also plots the entire range, but the output is wrong! Evidently, ListPlot3D cannot handle data with such an enormous range of variation. — bbgodfrey, Commented Jan 22, 2015 at 12:46
I think this is the familiar long-standing problem with the triangulation algorithm. This is related though the "DelaunayDomainScaling" option in my answer there doesn't work in version 10. A simple workaround would be to plot the probability as a percentage (data[[All,2]] *= 100), or the population in thousands. — Simon Woods, Commented Jan 22, 2015 at 13:57
Can you try with DataRange->{{xmin,xmax},{ymin,ymax},...}, or with DataRange -> All, respectivly? — user9660, Commented Jan 22, 2015 at 15:01

Community · Accepted Answer · 2017-04-13 12:55:57Z

As stated in the comments, this problem has to do with the fact that the different axes have wildly different scales. See the questions here and here for a discussion on this.

@Rahul gave a workaround that is specific to the case where the data is on a rectangular grid,

ListPlot3D[Transpose@Partition[Last /@ data, 15], 
 DataRange -> {{100, 2000}, {0.01, 0.08}}, PlotRange -> All, 
 ColorFunction -> "Rainbow"]

But we can make another workaround for the case where the data is even on an unstructured grid. The idea is to rescale the data before plotting, and then rescale the tick marks so as to match the original data ranges using the CustomTicks package.

Needs["CustomTicks`"];
rescaleListPlot3D[data_, plotopts : OptionsPattern[ListPlot3D]] := 
 Module[{rescaleddata},
  rescaleddata = 
   Transpose[Rescale[#, MinMax@#] & /@ Transpose[data]];
  ListPlot3D[rescaleddata, 
   Ticks -> 
    Function[mm, 
      LinTicks[Sequence @@ mm, 
       TickPostTransformation -> (Rescale[#, mm, {0, 1}] &),
       TickLabelFunction -> (N@# &)]] /@ (MinMax /@ Transpose[data]), 
   plotopts]
  ]

rescaleListPlot3D[data, PlotRange -> All, 
 AxesLabel -> {Population Size, Mutation Probability, Generations}, 
 ColorFunction -> "Rainbow"]

And we can make a contour and density plot version, since these also suffer from the same interpolation problems,

rescaleListDensityPlot[data_, 
   plotopts : OptionsPattern[ListDensityPlot]] := 
  Module[{rescaleddata},
   rescaleddata = data;
   rescaleddata[[All, ;; 2]] = 
    Transpose[
     Rescale[#, MinMax@#] & /@ Transpose[data[[All, ;; 2]]]];
   ListDensityPlot[rescaleddata, plotopts,
    FrameTicks ->
     (Function[mm,
        {LinTicks[Sequence @@ mm, 
          TickPostTransformation -> (Rescale[#, mm, {0, 1}] &),
          TickLabelFunction -> (N@# &)],
         StripTickLabels[
          LinTicks[Sequence @@ mm, 
           TickPostTransformation -> (Rescale[#, mm, {0, 1}] &),
           TickLabelFunction -> (N@# &)]]}] /@ (MinMax /@ 
         Transpose[data[[All, {2, 1}]]]))]
   ];
rescaleListContourPlot[data_, 
  plotopts : OptionsPattern[ListContourPlot]] := Module[{rescaleddata},
  rescaleddata = data;
  rescaleddata[[All, ;; 2]] = 
   Transpose[Rescale[#, MinMax@#] & /@ Transpose[data[[All, ;; 2]]]];
  ListContourPlot[rescaleddata, plotopts,
   FrameTicks ->
    (Function[mm,
       {LinTicks[Sequence @@ mm, 
         TickPostTransformation -> (Rescale[#, mm, {0, 1}] &),
         TickLabelFunction -> (N@# &)],
        StripTickLabels[
         LinTicks[Sequence @@ mm, 
          TickPostTransformation -> (Rescale[#, mm, {0, 1}] &),
          TickLabelFunction -> (N@# &)]]}] /@ (MinMax /@ 
        Transpose[data[[All, {2, 1}]]]))]
  ];

and compare the results of plotting with and without the rescaling.

Grid[{
  {ListDensityPlot[data, ColorFunction -> "Rainbow", PlotRange -> All,
     PlotLegends -> Automatic],
   rescaleListDensityPlot[data, ColorFunction -> "Rainbow", 
    PlotRange -> All, PlotLegends -> Automatic]},
  {ListContourPlot[data, ColorFunction -> "Rainbow", PlotRange -> All,
     PlotLegends -> Automatic],
   rescaleListContourPlot[data, ColorFunction -> "Rainbow", 
    PlotRange -> All, PlotLegends -> Automatic]
   }}]

Stack Exchange Network

ListPlot3D. Only plots about half of the data in a 3D matrix

1 Answer 1

Your Answer

Not the answer you're looking for? Browse other questions tagged
plotting
or ask your own question.

Linked

Hot Network Questions

ListPlot3D. Only plots about half of the data in a 3D matrix

1 Answer 1

Your Answer

Sign up or log in

Post as a guest

Not the answer you're looking for? Browse other questions tagged plotting or ask your own question.

Linked

Related

Hot Network Questions

Not the answer you're looking for? Browse other questions tagged
plotting
or ask your own question.