In the definition of s you're summing from k==0. Since the summand has a term 1/k this gives a divide-by-zero error when calculating the partial sums. The sum should in fact start from k==1 (the zeroth coefficient is taken care of by the constant term in front of the sum). The first few approximations then look like
s[n_, x_] :=
8/4 + 3/(9 π) Sum[(6 (-1)^k)/(k π) Cos[(k π x)/
2] + (16 (-1)^k + 13)/(π k) Sin[(k π x)/2], {k, 1, n}]
partialsums = Table[s[n, x], {n, 1, 5}];
Plot[Evaluate[partialsums], {x, -4, 4}]
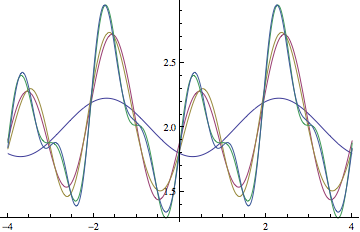
To compare this with f we plot the s[5,x] and f in the same plot:
Plot[{s[5, x], f[x]}, {x, -2, 2}]
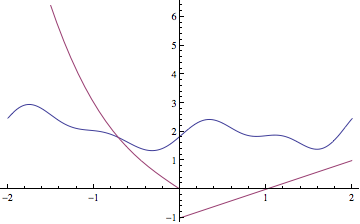
which doesn't seem right to me, so I suspect you made a mistake somewhere in calculating the coefficients.
Calculating coefficients by hand
We could use FourierSeries to calculate the partial sums, but this is very slow, and doesn't give produce the general equation for the coefficients. Therefore it's better to calculate the coefficients by hand. The $n$-th coefficient can be calculated according to
coeff[0] = 1/4 Integrate[f[x], {x, -2, 2}];
coeff[n_] = 1/4 Integrate[f[x] Exp[I Pi n x/2], {x, -2, 2}]
(1/(2 n^4 π^4))E^(-I n π) (-48 + 48 E^(I n π) -
48 I n π + 28 n^2 π^2 - 6 E^(I n π) n^2 π^2 +
2 E^(2 I n π) n^2 π^2 + 12 I n^3 π^3 -
I E^(I n π) n^3 π^3 - I E^(2 I n π) n^3 π^3)
Then the partial sums are given by
series[m_, x_] := Sum[Exp[-I Pi n x/2] coeff[n], {n, -m, m}]
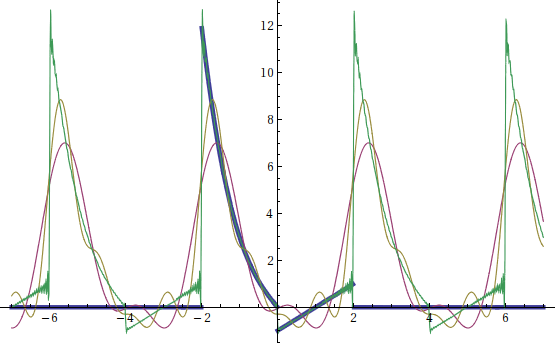
Plotting the first few approximations:
Plot[Evaluate[Table[series[j, x], {j, 0, 5}]], {x, -6, 6}]
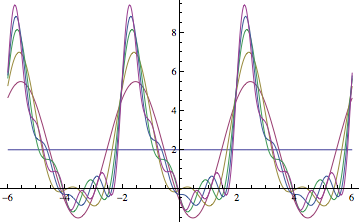
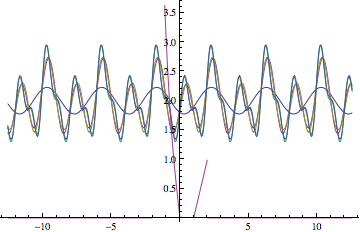
To see how this compares with the original function f:
Plot[Evaluate[{series[5, x], f[Mod[x, 4, -2]]}], {x, -4, 4}]
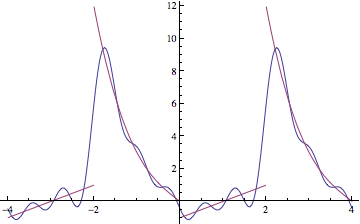
which looks a lot better than the before.
Edit: Real coefficients
Here, coeff[n] are the coefficients for the Fourier series in exponential form, but these can be easily converted to the coefficients for the $\cos$ and $\sin$ series, a_n and b_n, by doing something like
a[0] = coeff[0];
a[n_] = Simplify[ComplexExpand[coeff[n] + coeff[-n]]];
b[n_] = Simplify[ComplexExpand[I (coeff[n] - coeff[-n])]];


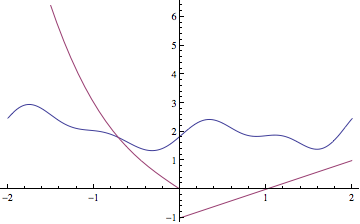


{k,1,n}. I actually only want to plot a certain amount of partial sums. Does this number go where thenis located? $\endgroup$