Correctly specifying Filling option for ListPointPlot3D

Question

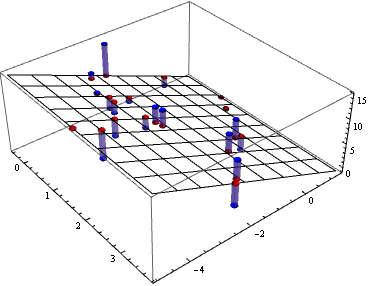
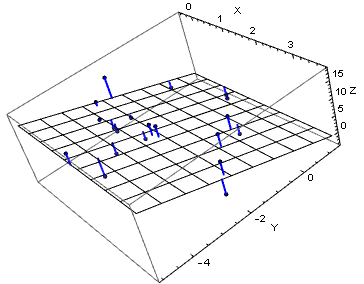
I'm fitting a linear model to some three dimensional data (x,y = explanatory variable, z = predicted variable). I am trying to visualize the data and model fit using a combination of 3D plots. Specifically, I want to plot the data in 3D plot and then have a 'fill' line down to a plane in 3D space that represents the model prediction. The problem I am having is figuring out the correct options for "Filling" in ListPointPlot3D to get the desired effect. I have tried 1->2, 1->{2}, {1->2}, and other variants but none of htem have the desired effect.

Here's an attempt to illustrate the problem.

xyzData = {{-0.31`, 0.222`, -0.025`}, {3.754`, -2.502`, 
    4.4`}, {0.658`, 1.301`, -3.481`}, {-0.377`, -1.869`, 
    13.746`}, {2.335`, -0.487`, 7.506`}, {0.618`, -2.151`, 
    7.045`}, {1.150`, 0.8250`, 0.148`}, {2.783`, -1.37`, 
    9.404`}, {0.774`, -2.823`, 7.264`}, {1.435`, -3.508`, 
    7.888`}, {3.64`, -2.308`, 11.922`}, {0.579`, -2.767`, 
    7.018`}, {1.754`, -2.317`, 11.3860`}, {1.791`, -4.276`, 
    7.58`}, {0.43`, -3.012`, 10.156`}, {1.406`, -2.485`, 
    6.463`}, {1.693`, -4.999`, 18.205`}, {1.618`, -2.394`, 
    11.532`}, {2.289`, -0.19`, 0.067`}, {-0.232`, -2.521`, 9.826`}};

(*get information on range of data values*)
{xData, yData, zData} = 
  Transpose[xyzData];
{xMin, xMax} = {Min[xData], Max[xData]};
{yMin, yMax} = {Min[yData], Max[yData]};
{zMin, zMax} = {Min[zData], Max[zData]};

(*fit linear model to the data*)

model =  LinearModelFit[xyzData, {x1, x2}, {x1, x2}];

(*create expected values based on linear fit *)

predictZ[x_, y_] = {x, y, model[x, y]};
predictedZ = Map[predictZ[#[[1]], #[[2]] ] &, xyData];

plot3d = Show[
   (*plot observed values and predicted values based on model
   Goal is to have a line drawn between the observed and predicted \
  values to aid in visualization*)

   ListPointPlot3D[ {xyzData, predictedZ},
    PlotRange -> All,
    PlotStyle -> {Directive[Black, PointSize[Medium]], 
      Directive[Red, PointSize[Small]]},
    Filling -> {1 -> {{2}, {Red, Blue}}}(*this doesn't work, 
    nor does "Filling -> 1\[Rule]{2}" or "Filling -> {1\[Rule]2}" *)
   ],

   (*create a plane in 3D space of the expected values based on the model.
   The predicted values should lie on this plane
    *)

    Plot3D[model[x, y] , {x, xMin, xMax}, {y, yMin, yMax}, 
        PlotStyle -> Directive[Opacity[0.1], Specularity[], Glow[White]],
        Mesh -> 8,
        ViewVertical -> {0, 0, 1}], 

       PlotRange -> All, 
       AxesLabel -> {"X", "Y", "Z"}, 
       LabelStyle -> Directive[FontFamily -> "Helvetica"]];

showPlot3D = Show[plot3d]

Answer 1

Update: Another work-around: Use DiscretePlot3D

You can use the datasets xyzData and predictedZ to define two functions, say foo1 and foo2

ClearAll[foo1, foo2];
(foo1[#, #2] = #3) & @@@ xyzData;
(foo2[#, #2] = #3) & @@@ predictedZ;

dp3d = DiscretePlot3D[{foo1[x, y], foo2[x, y]}, 
    {x, xyzData[[All, 1]]}, {y, xyzData[[All, 2]]}, ImageSize -> 400, 
   BoxRatios -> 1, ExtentSize -> 1/10, Filling -> {1 -> {2}}, 
   PlotStyle -> {Blue, Red}, PlotMarkers -> {"Sphere", Small}];

p3d = Plot3D[model[x, y], {x, xMin, xMax}, {y, yMin, yMax}, 
   PlotStyle -> Directive[Opacity[0.1], Specularity[], Glow[White]], 
   Mesh -> 8, ViewVertical -> {0, 0, 1}];

Show[p3d, dp3d]

---

Original post:

A work-around: add the vertical lines using Graphics3D:

Show[ListPointPlot3D[{xyzData, predictedZ}, PlotRange -> All, 
  PlotStyle -> {Directive[Black, PointSize[Medium]], 
    Directive[Red, PointSize[Small]]}], 
 Plot3D[model[x, y], {x, xMin, xMax}, {y, yMin, yMax}, 
  PlotStyle -> Directive[Opacity[0.1], Specularity[], Glow[White]], 
  Mesh -> 8, ViewVertical -> {0, 0, 1}], 
 Graphics3D[{Blue, Thick, Line /@ Transpose[{xyzData, predictedZ}]}], 
 PlotRange -> All, AxesLabel -> {"X", "Y", "Z"}, 
 LabelStyle -> Directive[FontFamily -> "Helvetica"]]