I'm using Mathematica to prepare some teaching materials based on the discussion of the damped, driven pendulum in Chapter 12 of Taylor's Classical Mechanics. This involves using NDSolveValue to solve the non-linear ODE
$$
\ddot{\phi} + 2 \beta \dot{\phi} + \omega_0^2 \sin \phi = \gamma \omega_0^2 \cos \omega t.
$$
We then want to create a phase plot, which is a parametric curve of the form $(\phi(t), \phi'(t))$ as a function of $t$.
This is easy enough to implement:
DDPeq = D[ϕ[t], {t, 2}] + 2 β D[ϕ[t], t] + Subscript[ω, 0]^2 Sin[ϕ[t]] ==
γ Subscript[ω, 0]^2 Cos[ω t]
paramvals = {ω -> 2 π, Subscript[ω, 0] -> 3 π, β -> 3 π/8, γ -> 1.5}
tmin = 0;
tmax = 200;
ϕsol1[t_] = NDSolveValue[{DDPeq /. paramvals, ϕ[0] == -π/2, ϕ'[0] == 0},
ϕ[t], {t, tmin, tmax}];
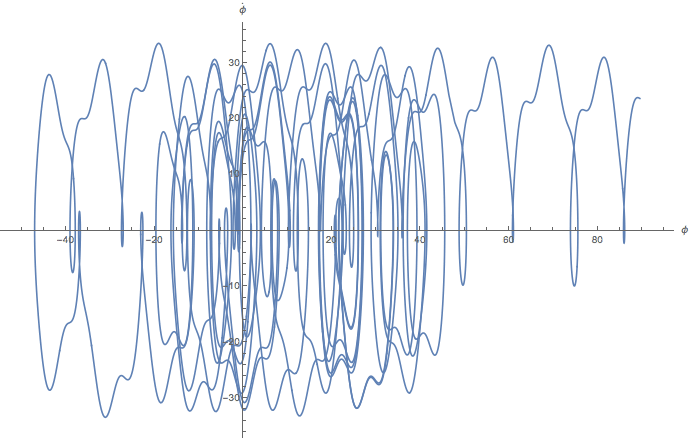
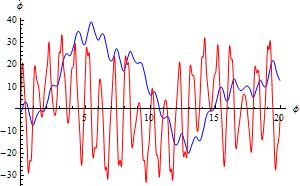
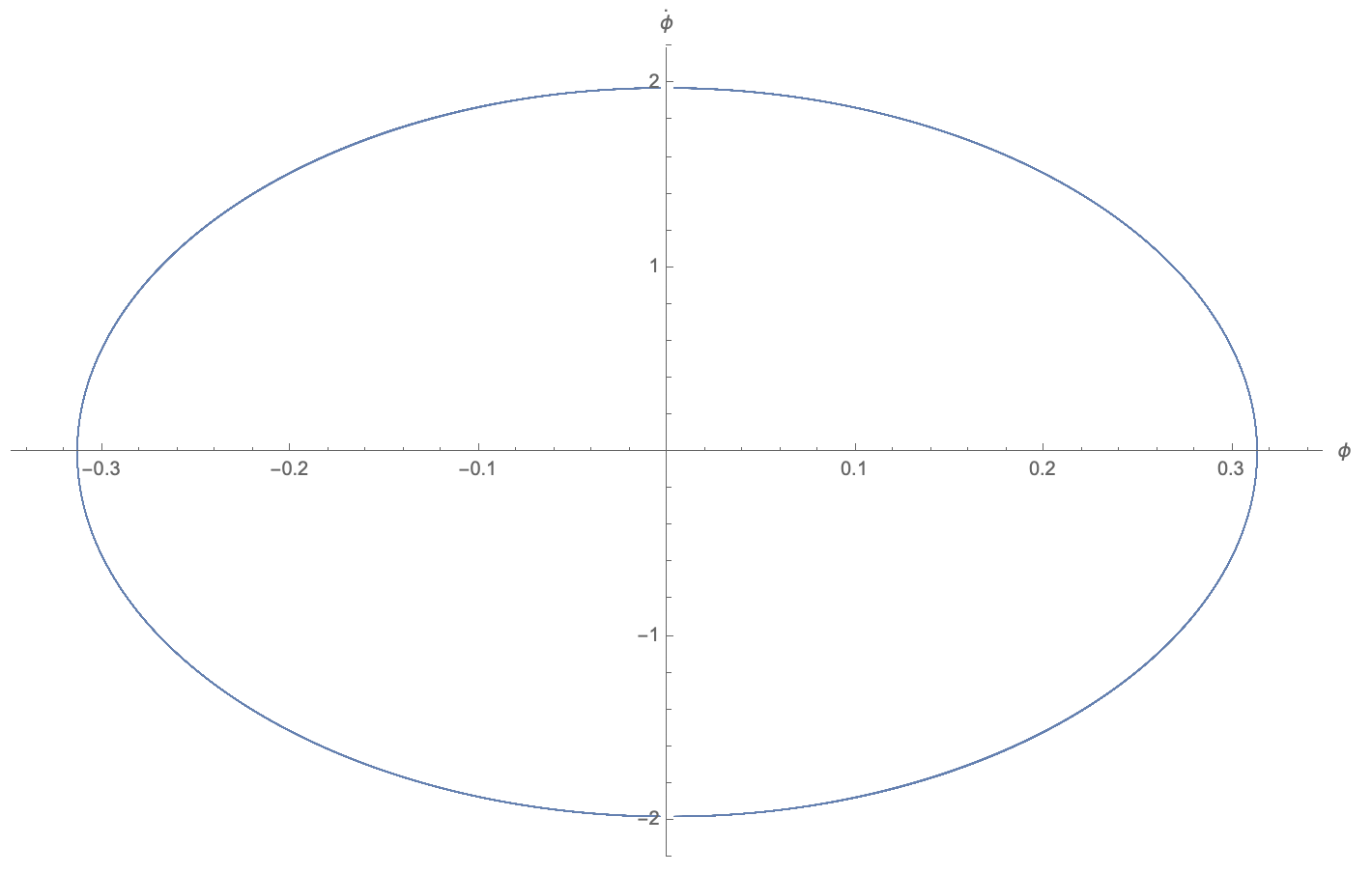
ParametricPlot[{ϕsol1[t], ϕsol1'[t]}, {t, 0, tmax/4},
AspectRatio -> 1/GoldenRatio, AxesLabel -> {ϕ, OverDot[ϕ]},
ImageSize -> Full, PlotPoints -> 100]
However, the angle of the pendulum is really only defined modulo $2 \pi$; ideally, what we'd like to do is have Mathematica plot with the horizontal axis "wrapped around". We can use Mod to calculate this, and we can use Exclusions to ensure that the opposite edges of the graph aren't connected.
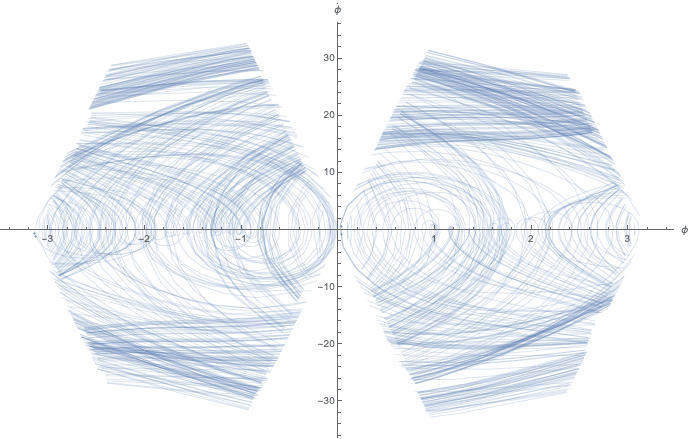
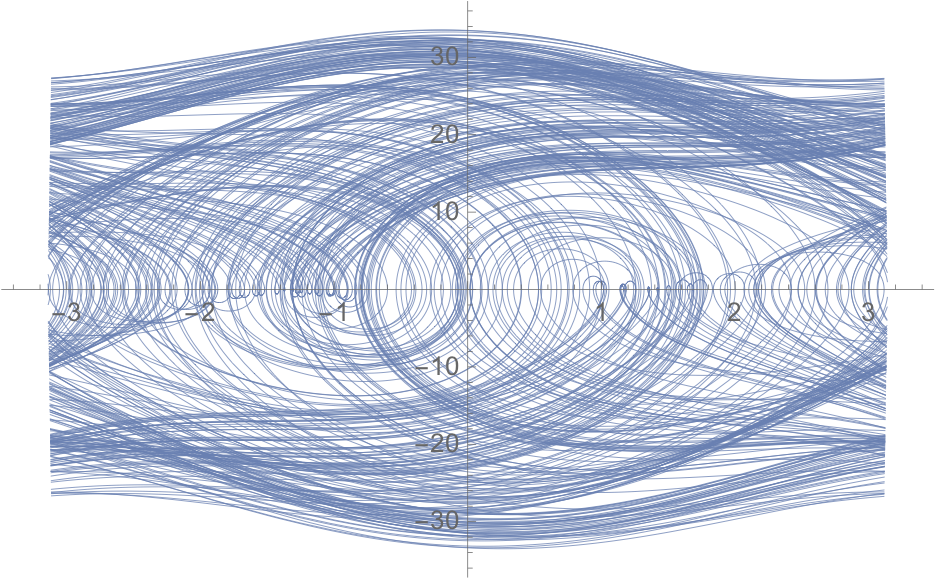
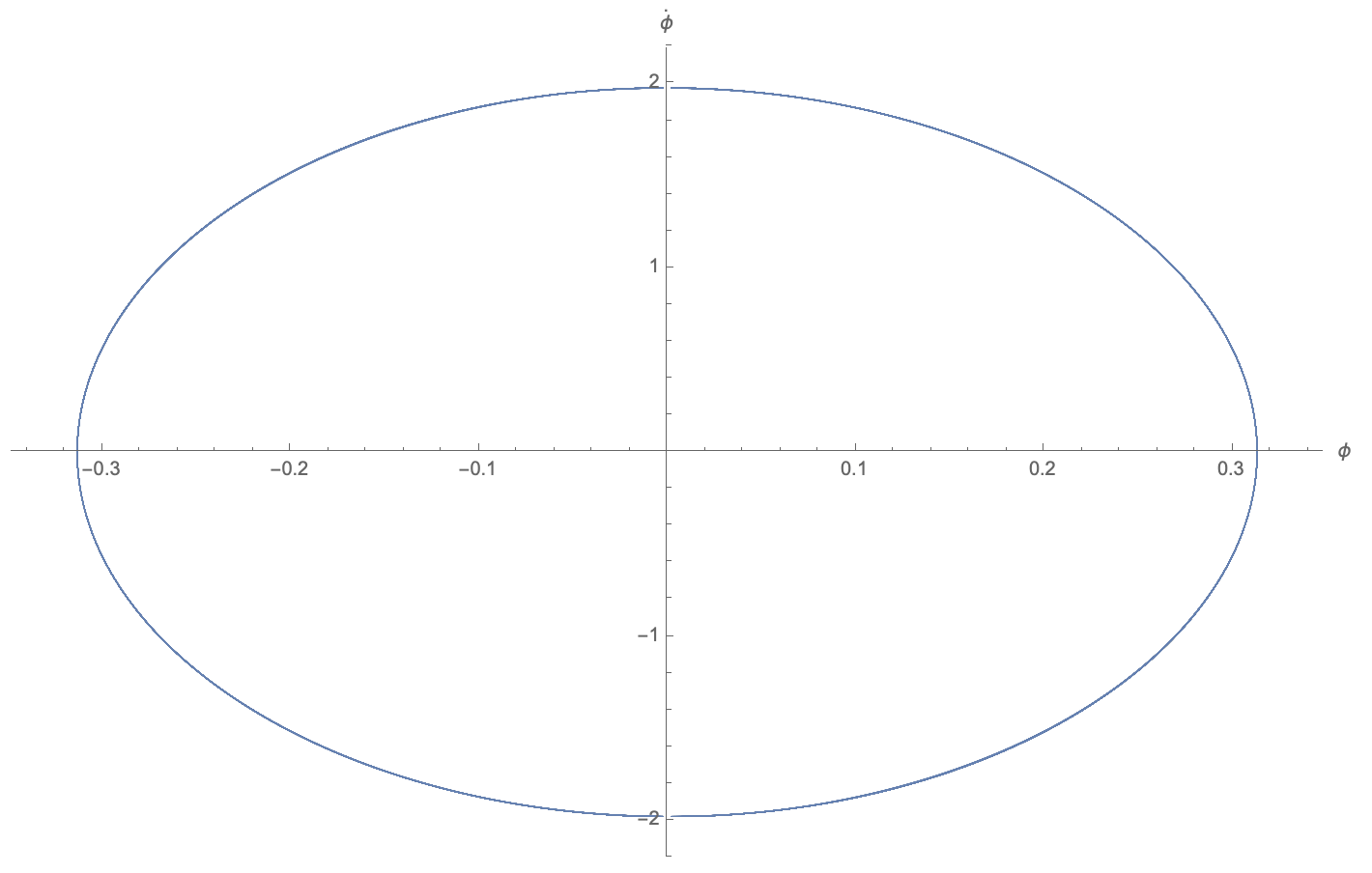
ParametricPlot[{Mod[ϕsol1[t], 2 π, -π], ϕsol1'[t]}, {t, tmax/10, tmax},
AspectRatio -> 1/GoldenRatio, AxesLabel -> {ϕ, OverDot[ϕ]}, ImageSize -> Full,
PlotPoints -> 100, Exclusions -> Mod[ϕsol1[t], 2 π] == π, PlotStyle -> Thin]
The problem: Mathematica also seems to be "breaking" the plots when $\phi = 0$ (modulo $2 \pi$); you can see that the lines are broken whenever the curve crosses the vertical axis. Increasing PlotPoints makes this problem less noticeable, by narrowing the span of the curve that is omitted (at the edges as well as at the vertical axis); however, the gaps are still present.
Why is Mathematica excluding points that I didn't want it to exclude? Also, is there a better way to create this plot? (I'm still using Mathematica 10.4.1, though my college now has a site license for 11, so if there's a nifty feature in 11 that fixes this problem, I'm all ears.)
EDIT: Two years later, I'm teaching the course again, and the problem is still bugging me. What's more, the default behavior in Mathematica 12.0 is even worse.
The missing "wedges" get smaller when I increase PlotPoints, but I have to crank that value up to 2000 or so to get a result I find acceptable.


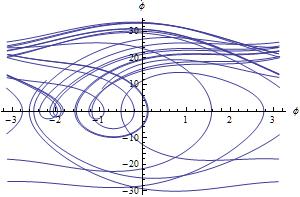
PhysicalSystemData[ Entity["PhysicalSystem", "DampedDrivenHarmonicOscillator1D"], "EquationsOfMotion"]. The output will allow comparing to the exact solution and plenty of properties [reference.wolfram.com/language/ref/entity/PhysicalSystem.html]. The built-inPhysicalSystemData[ Entity["PhysicalSystem", "DampedDrivenHarmonicOscillator1D"], "Classes"]contains the classification "exactly solvable systems". So what NDSolveValue calculates in your first graph is error accumulation. $\endgroup$PlotPoints -> 2000, MaxRecursion -> 6, Exclusions -> "Discontinuities". You would need to balanceMaxRecursionandPlotPointsfor the best performance. Having one too high and the other too low will be slower than ideal. $\endgroup$