I'm getting the same answer "1" for Exp[x] with x less than "1.1*10^-16", and I really need to calculate much smaller numbers (in order of 10^-40).
I tried using SetPrecision[Exp[x],1000].
Obviously I'm doing something wrong. #_-
I've searched about increasing the precision but I couldn't find helpful answers. Would you please kindly guide me?
Edit:
Maybe this screenshot could make it easier to understand what I'm talking about.
You're setting the precision too late.
Exp[1.`200*^-40]
and
Exp[SetPrecision[1*^-40, 200]]
yield
On the other hand,
SetPrecision[Exp[1.*^-40], 200]
yields
because Exp[1.*^-40] evaluates to 1. (exactly, in machine precision), before it is passed to SetPrecision.
[1.1^-40, 200]] , Exp[(1.1`200)^-40] and Exp[(SetPrecision[1.1, 200])^-40] Hold different answers, aren't these supposed to be identical?
$\endgroup$
1.1^40 is first computed in 16-digit machine precision and will have significant differences when compared to the other 200-digit precision results. For the difference in the other two, examine 1.1`200 // FullForm and SetPrecision[1.1, 200] // FullForm. Here the difference arises because 1.1`200 is parsed to have 200-digit precision, whereas with SetPrecision, the 1.1 is first evaluated at 16-digit machine precision, then converted to 200-digit precision.
$\endgroup$
Commented
Dec 2, 2016 at 23:19
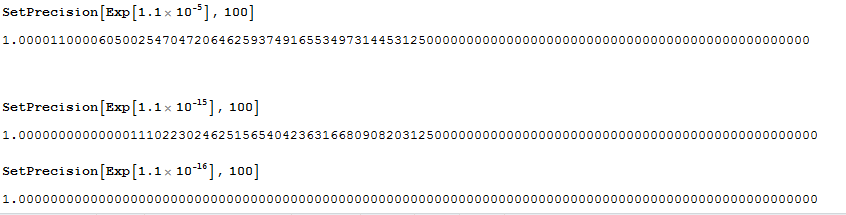
N[Exp[11/10000000000000000000000], 4000]. In other words, use rational numbers and not numbers with decimal points when you need lots of precision. $\endgroup$expm1function for this purpose, which returns $\exp(x)-1$ to high precision even when $\exp(x)$ would return exactly $1$. Mathematica unfortunately doesn't, but if that's your goal, you could implement something similar along the lines oflog1p. $\endgroup$