I'm trying to illustrate the solutions numerically and graphically for an equation such as Tan[x] == x. I think I did everything ok except I wanted to mark each intersection between Tan[x] and x.
Does anyone know how such a thing can be done?
$\begingroup$
$\endgroup$
2
2 Answers
$\begingroup$
$\endgroup$
13
Edited to make it a function.
For the strange Exclusions specification I use below, see my answer here. Thanks to @Oleksandr and @JM for their great comments.
plInters[{f1_, f2_}, {min_, max_}] :=
Module[{sol, x},
sol = x /. NSolve[f1[x] == f2[x] && min < x < max, x];
Framed@Show[
ListPlot[{#, f1[#]} & /@ sol, PlotStyle -> PointSize[Large]],
Plot[{f1[x], f2[x]}, {x, min, max}, Exclusions -> {True, f2[x] == 10, f1[x] == 10}]
]
]
GraphicsRow[plInters[#, {-10, 10}] & /@ {{# &, Tan}, {Tan, Coth}, {Sin, 1/# &}}]
answered Sep 12, 2012 at 0:59
-
1
-
1$\begingroup$ @kale That is one of the good things in this site. You can always find other ways. $\endgroup$ Commented Sep 12, 2012 at 1:01
-
1
-
1$\begingroup$ I don't really know why
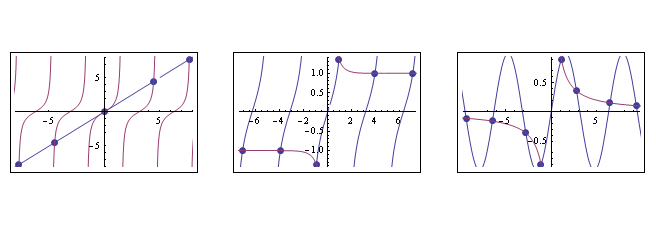
Plotcan sometimes determine exclusions properly and sometimes not. Still, this'll look a bit better if you setExclusions -> Pi/2 Range[-5, 5, 2]forPlot(otherwise, it may be harder for students to see that these aren't really solutions). $\endgroup$ Commented Sep 12, 2012 at 1:45 -
2$\begingroup$ @Alexey I tried to explain why here mathematica.stackexchange.com/a/10502/193. It gets the plot refinement routine to work to your advantage. $\endgroup$ Commented Sep 13, 2012 at 1:52
$\begingroup$
$\endgroup$
4
Update 3: Using Graphics`Mesh`FindIntersections to get the intersection points (see also):
showIntersections =
Block[{Annotation = # &},
Show[#, Graphics@{Red, PointSize[Large],
Point@Graphics`Mesh`FindIntersections@#}]] &;
Using the two examples in the original answer:
Row[showIntersections /@ {Plot[{Cos[x], x Sin[x]}, {x, -3 Pi, 3 Pi},
ImageSize -> 400],
Plot[{Tan[x], x Sin[x]}, {x, -3 Pi, 3 Pi}, ImageSize -> 400,
Exclusions -> Range[-5 Pi/2, 5 Pi/2, Pi]]}]
Original answer:
You can also use MeshFunctions:
Plot[{Cos[x], x Sin[x]}, {x, -3 Pi, 3 Pi},
MeshFunctions -> {(Cos[#] - # Sin[#]) &}, Mesh -> {{0}},
MeshStyle -> Directive[Red, PointSize[Large]]]
![plot of Cos[x] and x Sin[x]](https://i.sstatic.net/W1OJS.png)
Update: Dealing with Tan[x] using Exclusions
Plot[{Tan[x], x Sin[x]}, {x, -3 Pi, 3 Pi},
MeshFunctions -> {(Tan[#] - # Sin[#]) &}, Mesh -> {{0}},
MeshStyle -> Directive[Red, PointSize[Large]],
Exclusions -> Range[-5 Pi/2, 5 Pi/2, Pi]]
(* or Exclusions -> (Cos[x] == 0) *)
![plot of Tan[x] and x Sin[x]](https://i.sstatic.net/8TB1X.png)
Update 2: Using just Mesh and MeshStyle:
points = NSolve[Tan[x] == x Sin[x] && -3 Pi < x < 3 Pi, x][[All, 1, 2]];
Plot[{Tan[x], x Sin[x]}, {x, -3 Pi, 3 Pi},
Mesh -> {points},
MeshStyle -> {Directive[Red, PointSize[Large]]},
Exclusions -> Range[-5 Pi/2, 5 Pi/2, Pi]]
(* same picture as above *)
-
-
4$\begingroup$ This has some problems with the exclusions :( $\endgroup$ Commented Sep 12, 2012 at 5:03
-
2$\begingroup$ @R.M thank you. Belisarius, right... without
excludingthe vertical segments of theTanfunction (usingExclusionsorRegionFunction)MeshFunctionsdo not work. $\endgroup$– kglrCommented Sep 12, 2012 at 5:43 -
$\begingroup$ But that is not always easy > mathematica.stackexchange.com/q/10501/193 $\endgroup$ Commented Sep 12, 2012 at 13:28
Comment by the OP (migrated from the question) ->** I'm sorry if I'm not 'commenting' properly. I'll figure it out when I get more time here. I wanted to say THANKS to EVERYONE that posted. This is exactly what I was looking for. I will use all the input and make sure I learn from what was given. What a goldmine this site is for learning something like this. Thanks again, it's greatly appreciated!! $\endgroup$