Fitting data to peaks visually with manipulate - how to compactly implement for many peaks

Question

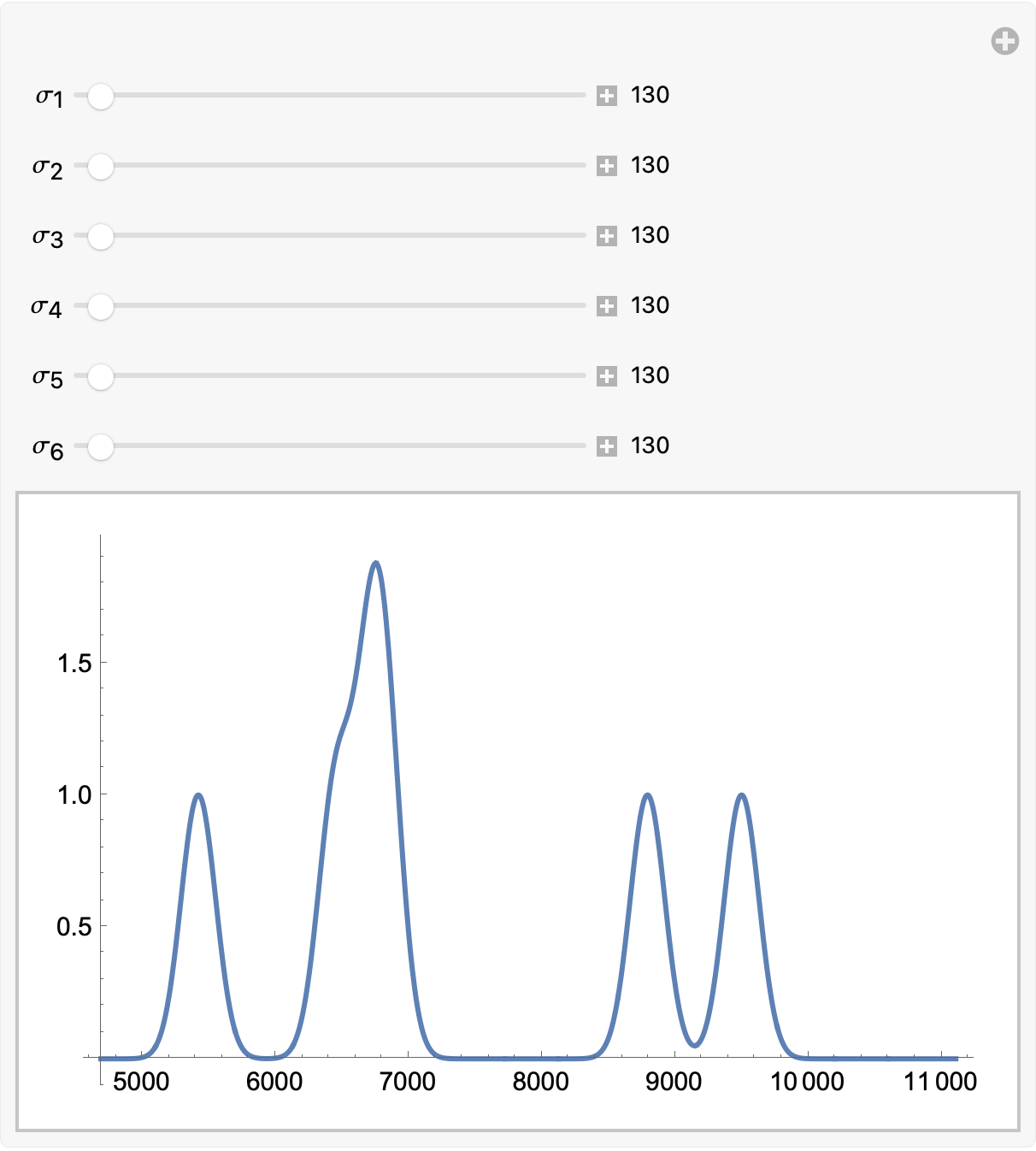
I am trying to create a visual aid for peak fitting. There should be a background profile and on top, I want to place peaks. So far, I am stuck with the following issue. For simplicity, I am using a gaussian and two peaks. I have:

μd = {5422.6501499999995`, 6450.7998`, 6711.8498500000005`, 
   6824.2998`, 8795.4004`, 9502.0996`};
{t0, t1} = {4690.5`, 11110.5`};

The following code works as intended (I manipulate the sigmas as I wish):

ClearAll[model0]
model0 = Exp[-((t - μd[[1]])^2/(2 σ1^2))] + Exp[-((t - μd[[2]])^2/(2 σ2^2))];

With[
 {localmodel = model0 /. {σ1 -> σv1, σ2 -> σv2}},
 Manipulate[
  Quiet@Plot[localmodel, {t, t0, t1}, PlotRange -> Full],
  {{σv1, 100}, 0.01, t1 - t0}, 
  {{σv2, 100}, 0.01, t1 - t0}
 ]
]

Since I am planning to have more peaks in the model, the above will become soon cumbersome and I was hoping to simplify things by defining the model compactly but the approach below is not working (I have never understood how Mathematica interprets subscripts):

model1 = Sum[Exp[-((t - μd[[i]])^2/(2 Subscript[σ, i]^2))], {i, 1, 2}]

With[
 {localmodel = model1 /. {σ -> σv}},
 Manipulate[
  Show[
   Plot[model1[t, σ], {t, t0, t1}, PlotRange -> Full],
  ],
  {{Subscript[σv, 1], 1000}, 0.01, t1 - t0}, 
  {{Subscript[σv, 2], 1000}, 0.01, t1 - t0}
 ]
]

How should I go about this so that I can define things compactly, ideally also in the Manipulate variables?

Answer 1

$Version

(* "13.3.0 for Mac OS X ARM (64-bit) (June 3, 2023)" *)

Clear["Global`*"]

μd = {5422.6501499999995`, 6450.7998`, 6711.8498500000005`, 
   6824.2998`, 8795.4004`, 9502.0996`};

{t0, t1} = {4690.5`, 11110.5`};

Format[σ[i_]] := Subscript[σ, i]

n = 6;

model1 = Sum[Exp[-((t - μd[[i]])^2/(2 σ[i]^2))], {i, 1, n}];

With[{localmodel = model1 /.
    ((σ[#] -> σv[#]) & /@ Range[n])},
 Manipulate[
  Plot[localmodel, {t, t0, t1},
   PlotRange -> Full,
   PlotPoints -> 50,
   MaxRecursion -> 5],
  Evaluate[
   Sequence @@
    ({{σv[#], 130, Subscript[σ, #]}, 0.01, t1 - t0,
        Appearance -> "Labeled"} & /@
      Range[n])]]]