How to add constraints of derivative type to PDE using NDSolve

Question

The PDE looks like

      x1_dot+h1_dot=J11*(x1+h1)+J12*(x2+h2)
      x2_dot+h2_dot=J21*(x1+h1)+J22*(x2+h2)
      s.t. Gradient(h1[x1_0,x2_0])=0 and Gradient(h2[x1_0,x2_0])=0 at fixed point of [x1_0,x2_0]

where J11, J12, J21, J22 are coefficients; h1 and h2 are functions of x1 and x2; x1_dot and x2_dot are the derivatives with respect to time t. h1_dot can be converted to partial_h1/dx1x1_dot + partial_h1/dx2x2_dot.

The ODE of d[x1,x2]/dt is as follows:

        x1_dot = w0*x2
        x2_dot = (Pm-Pe*Sin[x1]-Damp*x2)/TJ

So the fixed point can be computed as x1_0=asin(Pm/Pe)=0.822, x2_0=0

I have two questions.

1. How to add the constraints of Gradient(h1)=0 and Gradient(h2)=0.
2. I'm aware that I need more boundary conditions to make h1 and h2 unique, but above is everything I have. How can I give or estimate the right boundary conditions?

Below are my code and output from Mathematica

w0 = 314.1593
Pm = 1.2
Pe = 1.6382
Damp = 5
TJ = 6

NDSolve[{
   w0*x2 + D[h1[x1, x2], x1]*(w0*x2) + D[h1[x1, x2], x2]*(Pm - Pe*Sin[x1]
     - Damp*x2)/TJ == w0*(x2 + h2[x1, x2]), 
  (Pm - Pe*Sin[x1] - Damp*x2)/TJ + D[h2[x1, x2], x1]*(w0*x2) + 
    D[h2[x1, x2], x2]*(Pm - Pe*Sin[x1] - Damp*x2)/TJ ==
      -(Pe^2 - Pm^2)^0.5/TJ*(x1 + h1[x1, x2]) - 
        Damp/TJ*(x2 + h2[x1, x2])}, 
  {h1[x1, x2], h2[x1, x2]}, {x1, -1, 4}, {x2, -0.04, 0.04}
]

Plot3D[h1[x1, x2], {x1, -1, 4}, {x2, -0.04, 0.04}]

Answer 1

The direct problem to determine points {x1,x2} where restrictions $\nabla h1=\nabla h2=0$ are true has solution as follows:

w0 = 314.1593;
Pm = 1.2;
Pe = 1.6382;
Damp = 5;
TJ = 6;
eq = {w0*x2 + D[h1[x1, x2], x1]*(w0*x2) + 
     D[h1[x1, x2], x2]*(Pm - Pe*Sin[x1] - Damp*x2)/TJ == 
    w0*(x2 + h2[x1, x2]), (Pm - Pe*Sin[x1] - Damp*x2)/TJ + 
     D[h2[x1, x2], x1]*(w0*x2) + 
     D[h2[x1, x2], x2]*(Pm - Pe*Sin[x1] - Damp*x2)/
       TJ == -(Pe^2 - Pm^2)^0.5/TJ*(x1 + h1[x1, x2]) - 
     Damp/TJ*(x2 + h2[x1, x2])};
bc = DirichletCondition[{h1[x1, x2] == 0, h2[x1, x2] == 0}, x1 == -1];

s = NDSolve[{eq, bc}, {h1[x1, x2], h2[x1, x2]}, {x1, -1, 
    4}, {x2, -0.04, 0.04}];

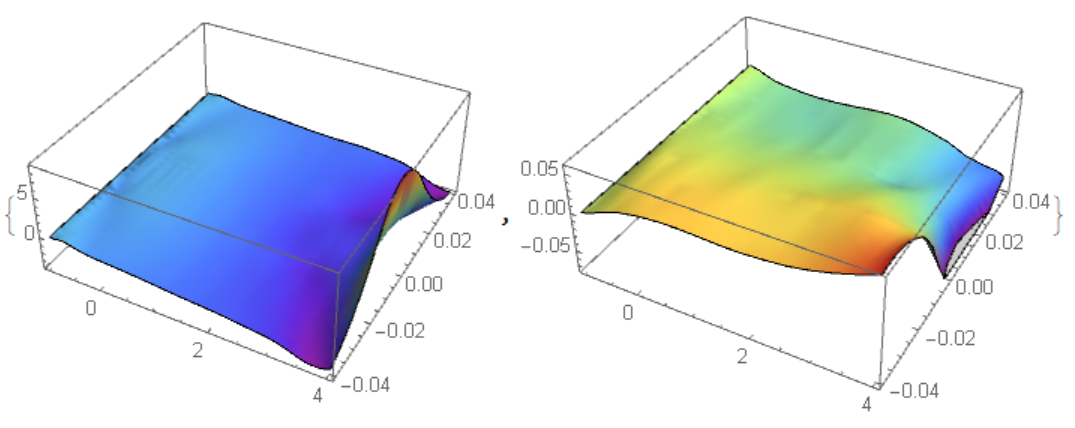
Visualization of numerical solution

{Plot3D[h1[x1, x2] /. s[[1]], {x1, -1, 4}, {x2, -0.04, 0.04}, 
  PlotRange -> All, Mesh -> None, ColorFunction -> "Rainbow"], 
 Plot3D[h2[x1, x2] /. s[[1]], {x1, -1, 4}, {x2, -0.04, 0.04}, 
  ColorFunction -> "Rainbow", Mesh -> None]}

Now we are looking points where restrictions are true, for this we use

eq1 = 
 eq /. {D[h1[x1, x2], x1] -> 0, D[h2[x1, x2], x2] -> 0, 
   D[h1[x1, x2], x2] -> 0, D[h2[x1, x2], x1] -> 0}

Out[]= {0. + 314.159 x2 == 314.159 (x2 + h2[x1, x2]), 
 0. + 1/6 (1.2 - 5 x2 - 1.6382 Sin[x1]) == -0.185869 (x1 + 
      h1[x1, x2]) - 5/6 (x2 + h2[x1, x2])}

Then we solve eq1 using

Solve[eq1, {h1[x1, x2], h2[x1, x2]}]

Out[]= {{h1[x1, x2] -> -1.07603 - 1. x1 - 5.97316*10^-16 x2 + 
    1.46896 Sin[x1], h2[x1, x2] -> 0.}}

This general solution can be plot with our numerical solution as

Show[ContourPlot[
  Evaluate[h2[x1, x2] == 0. /. s], {x1, -1, 4}, {x2, -0.04, 0.04}], 
 ContourPlot[
  Evaluate[h1[x1, x2] == -1.076027972872623` - 1.` x1 - 
      5.973155153119597`*^-16 x2 + 1.4689575209666093` Sin[x1] /. 
    s], {x1, -1, 4}, {x2, -0.04, 0.04}, ContourStyle -> Red]] 

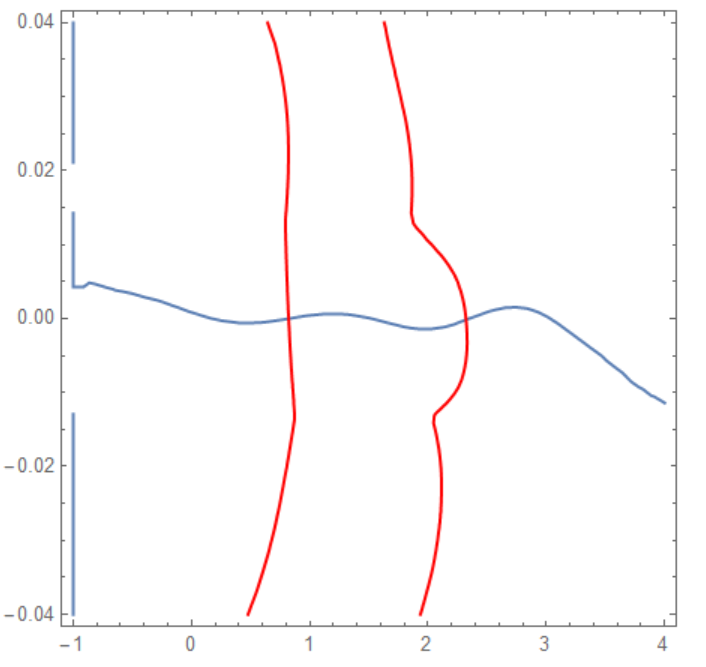
Points where gray and red lines crossing we can calculate with

FindRoot[{Evaluate[h2[x1, x2] == 0. /. s], 
  h1[x1, x2] == -1.076027972872623` - 1.` x1 - 
     5.973155153119597`*^-16 x2 + 1.4689575209666093` Sin[x1] /. 
   s}, {{x1, 2.5}, {x2, 0}}]

Out[]= {x1 -> 2.32257, x2 -> -0.000184708}

 FindRoot[{Evaluate[h2[x1, x2] == 0. /. s], 
  h1[x1, x2] == -1.076027972872623` - 1.` x1 - 
     5.973155153119597`*^-16 x2 + 1.4689575209666093` Sin[x1] /. 
   s}, {{x1, .8}, {x2, 0}}]

Out[]= {x1 -> 0.820887, x2 -> -7.45875*10^-7}

Therefore in this case of special type of boundary condition we got two point. Now let consider invers problem: for a given points we need to define boundary conditions. Obviously this problem has no unique solution. But it can be solved with this code:

w0 = 314.1593;
Pm = 1.2;
Pe = 1.6382;
Damp = 5;
TJ = 6;
eq = {w0*x2 + D[h1[x1, x2], x1]*(w0*x2) + 
     D[h1[x1, x2], x2]*(Pm - Pe*Sin[x1] - Damp*x2)/TJ == 
    w0*(x2 + h2[x1, x2]), (Pm - Pe*Sin[x1] - Damp*x2)/TJ + 
     D[h2[x1, x2], x1]*(w0*x2) + 
     D[h2[x1, x2], x2]*(Pm - Pe*Sin[x1] - Damp*x2)/
       TJ == -(Pe^2 - Pm^2)^0.5/TJ*(x1 + h1[x1, x2]) - 
     Damp/TJ*(x2 + h2[x1, x2])};
bc = DirichletCondition[{h1[x1, x2] == a1, h2[x1, x2] == a2}, 
   x1 == -1];
 eq1 = eq /. {D[h1[x1, x2], x1] -> 0, D[h2[x1, x2], x2] -> 0, 
   D[h1[x1, x2], x2] -> 0, D[h2[x1, x2], x1] -> 0};
sol1=Solve[eq1, {h1[x1, x2], h2[x1, x2]}];   
x10 = 0.822; x20 = 0; c = 
 Last[First[First[sol1]]] /. {x1 -> x10, x2 -> x20}
sol0 = ParametricNDSolveValue[{eq, bc}, {h1[x10, x20], 
   h2[x10, x20]}, {x1, -1, 4}, {x2, -0.04, 0.04}, {b1, b2}];
s = FindRoot[{sol0[a1, a2][[2]] == 0, 
   sol[a1, a2][[1]] == c}, {{a1, 0.}, {a2, 0.}}]
(*{a1 -> -0.822004, a2 -> 0.}*)

Using parameters a1,a2 we can evaluate and plot solution

sol = ParametricNDSolveValue[{eq, bc}, {h1, h2}, {x1, -1, 
   4}, {x2, -0.04, 0.04}, {a1, a2}]


{Plot3D[sol[-.822004, 0][[1]][x1, x2], {x1, -1, 4}, {x2, -0.04, 0.04}, 
  PlotRange -> All, Mesh -> None, ColorFunction -> "Rainbow"], 
 Plot3D[sol[-.822004, 0][[2]][x1, x2], {x1, -1, 4}, {x2, -0.04, 0.04}, 
  PlotRange -> All, Mesh -> None, ColorFunction -> "Rainbow"]}

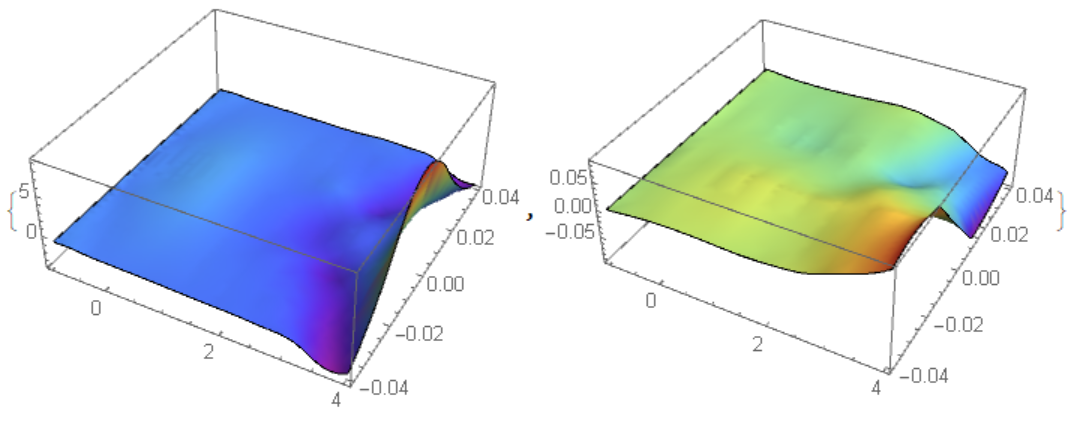
It is not unique solution, since we can apply some boundary condition for any border as well.