15
$\begingroup$

I have a polynomial curve that I got through interpolation.

pts1 = {{0, 0}, {12, 27}, {31, 52}, {58, 73}, {81, 85}};
y1 = pts1[[#, 1]] & /@ Range[Length[pts1]];
eq1 = Fit[pts1, {1, x, x^2, x^3, x^4}, x]//Chop;

$-2.74861*10^{-6}x⁴+0.00059554 x³-0.0516843 x²+2.7892 x$

eq1 /. x -> y1;

${0,27,52,73,85}$

pl1 = Plot[eq1, {x, Min[y1], Max[y1]}, Epilog -> {Blue, PointSize[0.02], Point[pts1]}, PlotRange -> {{-10, 100}, {-10, 100}}, AspectRatio -> 1, PlotStyle -> {Orange, Thick}]

I want to make another offset curve in 10 units. But I do not know how to proceed within Mathematica.

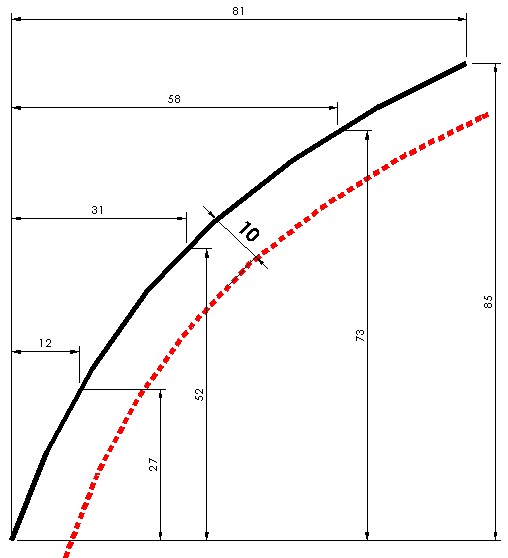
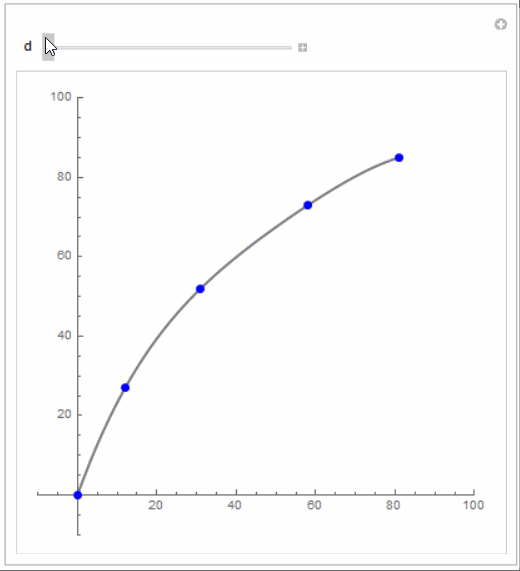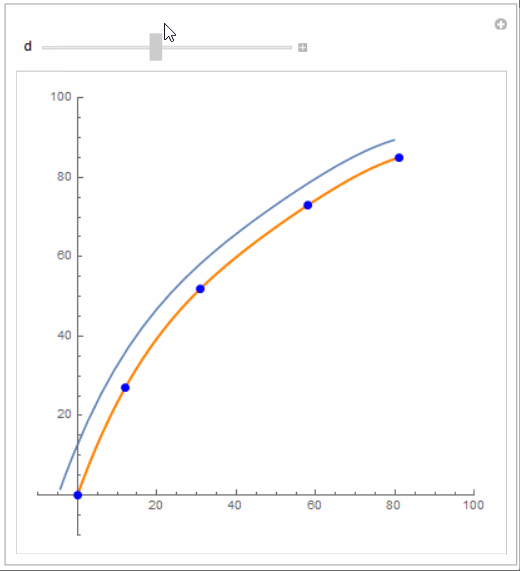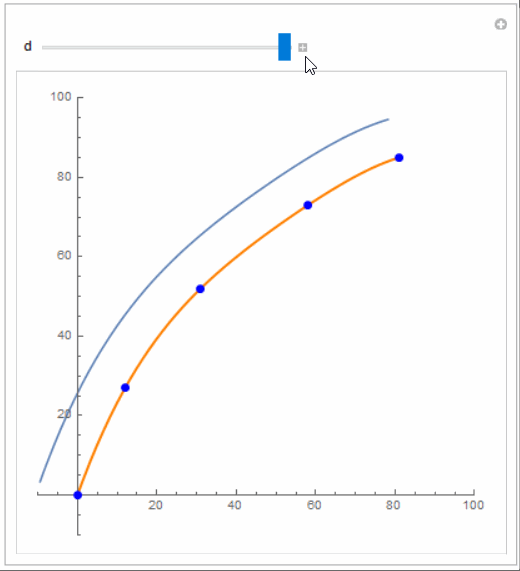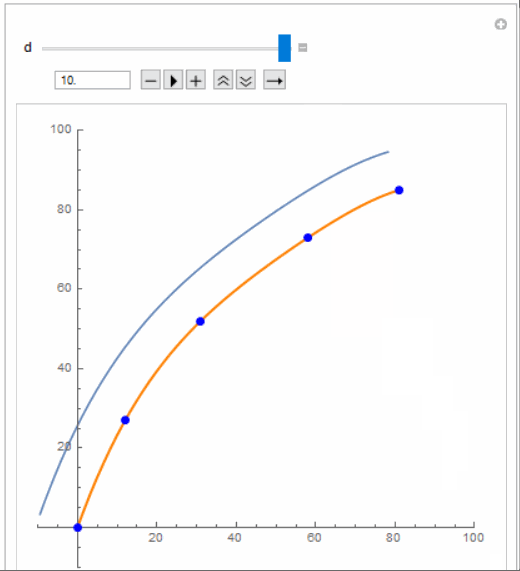
enter image description here

I made an offset through another software using multiple circles with a 10-unit radius to get the points I need.

enter image description here

Anyway, what would be the appropriate command to get these points?

Improve this question
$\endgroup$
2

3 Answers 3

Reset to default
11
$\begingroup$

This involves an algebraic curve so it can be done in closed form (one approach already shown does this, in the parametric form). We'll do the interpolation below at high precision in order to make some later computations more reliable.

pts1 = {{0, 0}, {12, 27}, {31, 52}, {58, 73}, {81, 85}};
f[x_] = Fit[N[pts1, 200], {1, x, x^2, x^3, x^4}, x];
N[f[x]]

(* Out[1252]= 0. + 2.78920381052 x - 0.0516843269209 x^2 + 
 0.000595539716825 x^3 - 2.74861498447*10^-6 x^4 *)

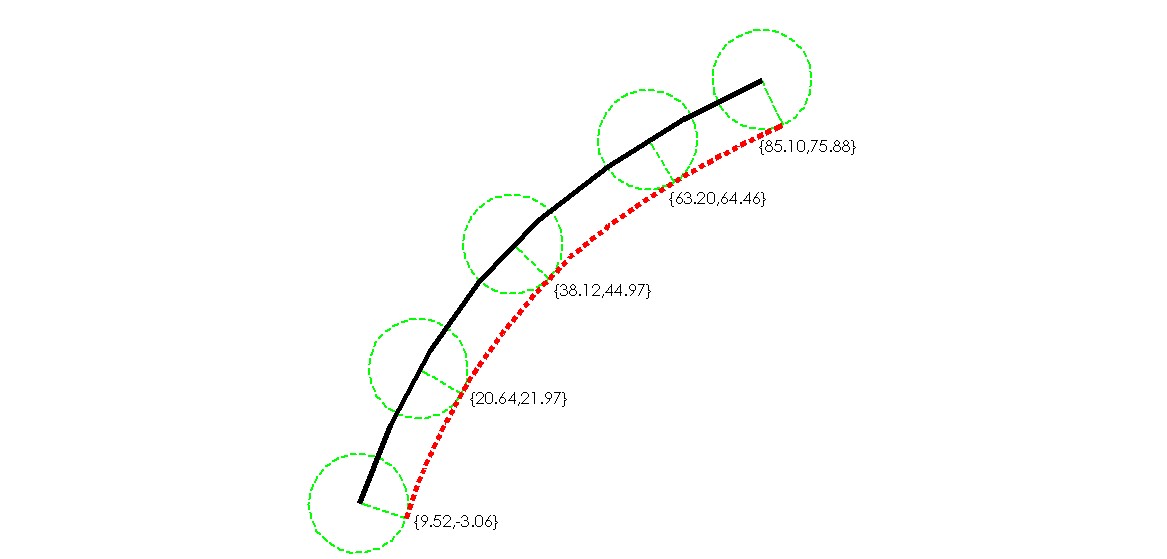
Here we find the parametric form of the (lower) offset curve).

offset[x_] = 
  With[{deriv = D[{x, f[x]}, x]},
    {grad = {-deriv[[2]], deriv[[1]]}},
    {x, f[x]} - grad/Sqrt[grad.grad]*10];

We check the plot.

ParametricPlot[{{t, f[t]}, offset[t]}, {t, 0, 80}]

plot

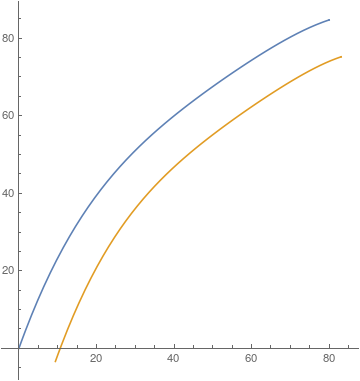
We can also implicitize. This part required the high precision interpolation. We could use Rationalize but that can get into round-off and cancellation error problems in plotting, since coefficients appear at very different scales.

imp = First[
   GroebnerBasis[Together[{x, y} - offset[t]], {x, y}, t, 
    MonomialOrder -> EliminationOrder]];
imp // N

(* Out[1260]= -6.73373194281*10^25 + 5.56558925951*10^24 x + 
 3.6738758353*10^23 x^2 - 4.34415318895*10^22 x^3 + 
 1.93344441642*10^21 x^4 - 5.36588154643*10^19 x^5 + 
 1.06609597248*10^18 x^6 - 1.60542472424*10^16 x^7 + 
 1.88490826277*10^14 x^8 - 1.74235671779*10^12 x^9 + 
 1.26230731848*10^10 x^10 - 7.01480518001*10^7 x^11 + 
 284576.519771 x^12 - 758.341572269 x^13 + 1. x^14 + 
 3.72787534059*10^24 y - 6.8504825964*10^23 x y + 
 9.94066556163*10^21 x^2 y + 7.41868236137*10^20 x^3 y - 
 4.34299189813*10^19 x^4 y + 1.19168781004*10^18 x^5 y - 
 2.1498342949*10^16 x^6 y + 2.77290008879*10^14 x^7 y - 
 2.63253515622*10^12 x^8 y + 1.82904347319*10^10 x^9 y - 
 8.95087000739*10^7 x^10 y + 280923.407204 x^11 y - 
 426.500272743 x^12 y - 3.17385078171*10^22 y^2 + 
 2.18287264042*10^22 x y^2 - 3.93259394774*10^20 x^2 y^2 - 
 1.00071010929*10^19 x^3 y^2 + 6.86935186234*10^17 x^4 y^2 - 
 1.75325222649*10^16 x^5 y^2 + 2.83739730618*10^14 x^6 y^2 - 
 3.21684582573*10^12 x^7 y^2 + 2.65009867828*10^10 x^8 y^2 - 
 1.59027982886*10^8 x^9 y^2 + 677296.69588 x^10 y^2 - 
 1950.02118583 x^11 y^2 + 3. x^12 y^2 - 1.48945282252*10^21 y^3 - 
 4.62703214764*10^20 x y^3 + 8.5373151706*10^18 x^2 y^3 + 
 6.50159149141*10^16 x^3 y^3 - 6.85046668404*10^15 x^4 y^3 + 
 1.58285949288*10^14 x^5 y^3 - 2.17546347848*10^12 x^6 y^3 + 
 1.98184395157*10^10 x^7 y^3 - 1.20720722952*10^8 x^8 y^3 + 
 465742.200007 x^9 y^3 - 853.000545485 x^10 y^3 + 
 6.2893710958*10^19 y^4 + 6.33039722844*10^18 x y^4 - 
 1.1302847025*10^17 x^2 y^4 + 4.83188192567*10^13 x^3 y^4 + 
 4.60017785742*10^13 x^4 y^4 - 9.9826564209*10^11 x^5 y^4 + 
 1.20048427437*10^10 x^6 y^4 - 9.37112169389*10^7 x^7 y^4 + 
 476873.182752 x^8 y^4 - 1625.01765486 x^9 y^4 + 3. x^10 y^4 - 
 1.09303426085*10^18 y^5 - 5.50342304678*10^16 x y^5 + 
 9.17636707233*10^14 x^2 y^5 - 4.3681823605*10^12 x^3 y^5 - 
 1.76328081245*10^11 x^4 y^5 + 3.57598551957*10^9 x^5 y^5 - 
 3.37083626949*10^7 x^6 y^5 + 184818.792803 x^7 y^5 - 
 426.500272743 x^8 y^5 + 1.03689348135*10^16 y^6 + 
 2.95870424611*10^14 x y^6 - 4.66457552293*10^12 x^2 y^6 + 
 3.02885768057*10^10 x^3 y^6 + 4.65196245499*10^8 x^4 y^6 - 
 1.00479219318*10^7 x^5 y^6 + 84153.0066438 x^6 y^6 - 
 433.338041296 x^7 y^6 + 1. x^8 y^6 - 5.50780682701*10^13 y^7 - 
 7.85681314181*10^11 x y^7 + 1.41189443972*10^10 x^2 y^7 - 
 1.57656872186*10^8 x^3 y^7 + 727639.19694 x^4 y^7 + 
 1.32364700231*10^11 y^8 *)

We can check the zero contour.

ContourPlot[imp == 0, {x, 0, 60}, {y, 0, 80}]

plot

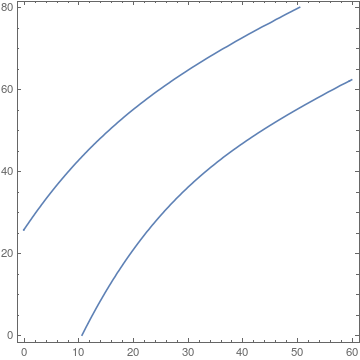
One will notice we got both upper and lower offsets. This is due to the fact that GroebnerBasis internals will make polynomial relations out of radicals, in effect losing information about sign on square roots.

Improve this answer
$\endgroup$
19
$\begingroup$ 
f[u_] := eq1 /. x -> u
n[u_] := {-D[eq1, x], 1} /. x -> u
plot[u_, d_] := {u, f[u]} + d Normalize[n[u]]
Manipulate[
 Show[pl1, ParametricPlot[plot[u, d], {u, 0, 81}]], {d, 0, 10}]

enter image description here

Improve this answer
$\endgroup$
2
  • 3
    $\begingroup$ +1. Wouldn't it be more efficient to use Set (=) instead of SetDelayed (:=), though? That way, you'd only calculate the derivative once, when the function is defined, instead of once for every plotted point $\endgroup$
    – Niki Estner
    Commented May 16, 2017 at 10:43
  • 2
    $\begingroup$ If anyone is curious, the Wikipedia article on parallel curves discusses the mathematics that are being used here. $\endgroup$
    – Michael Seifert
    Commented May 16, 2017 at 12:43
5
$\begingroup$

Using Interpolation

pts1 = {{0, 0}, {12, 27}, {31, 52}, {58, 73}, {81, 85}};

f = Interpolation[pts1];

{xmin, xmax} = MinMax[pts1[[All, 1]]];

pl1 = Plot[f[x], {x, xmin, xmax}, 
   Epilog -> {Blue, PointSize[0.02], Point[pts1]}, 
   PlotRange -> {{-10, 100}, {-10, 100}}, AspectRatio -> 1, 
   PlotStyle -> {Orange, Thick}];

Manipulate[
 Show[pl1,
  ParametricPlot[{
    x + a f'[x]/Sqrt[1 + f'[x]^2],
    f[x] - a/Sqrt[1 + f'[x]^2]},
   {x, xmin, xmax}]],
 {{a, 0}, -10, 10, 0.1, Appearance -> "Labeled"}]
Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.