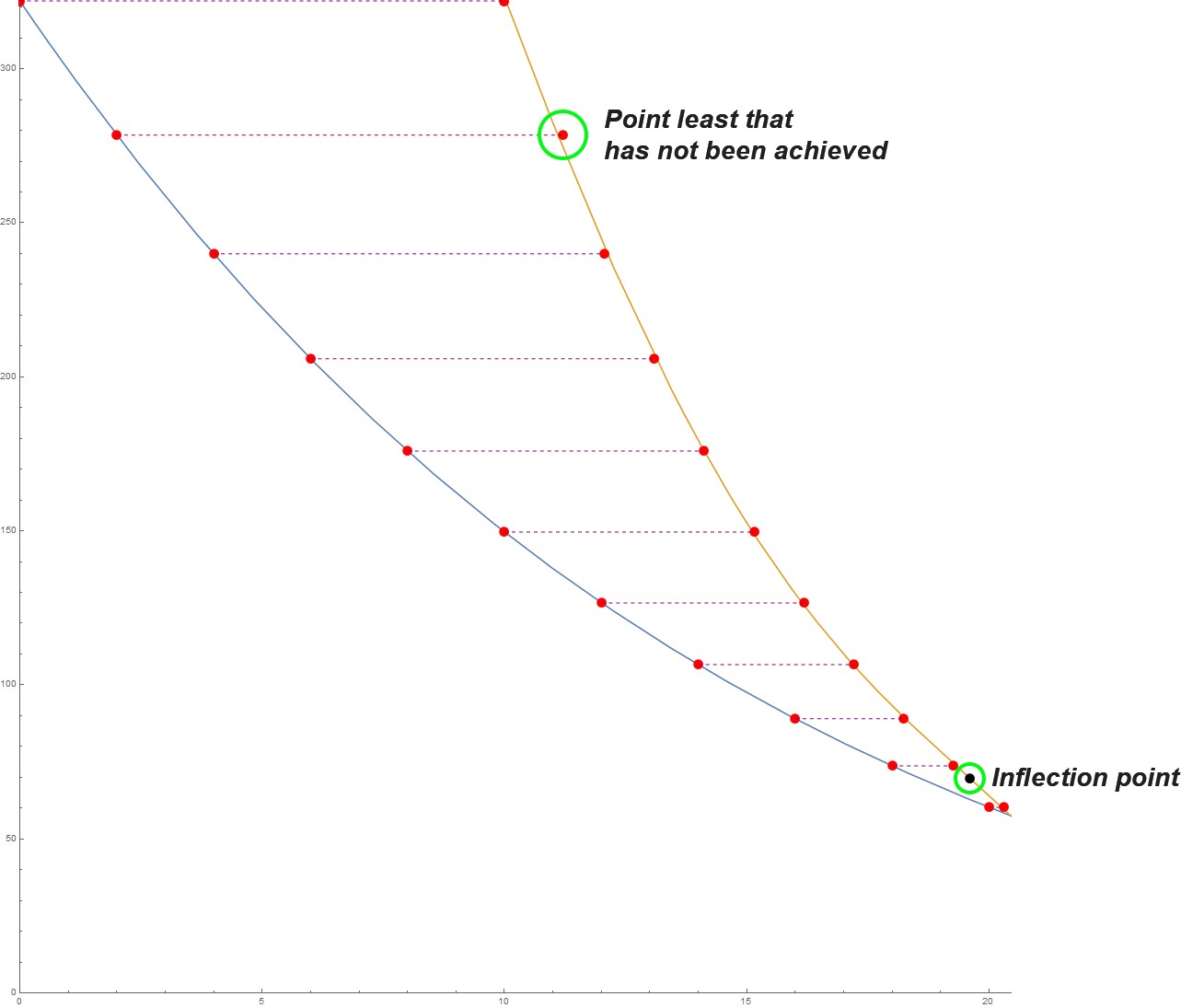
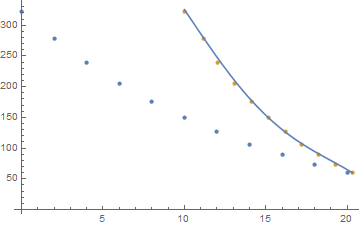
I have a function ($a(x)=0.00005x^4-0.01x^3+0.68x^2-23.087x+322$) which defines a profile desired.
I need to get a new function that is shifted relative to the first function using a list (offset=${10,9.2,8.06,7.09,6.12,5.15,4.18,3.21,2.24,1.27,0.3}$) that determines points least for this new function.
This means that my new function should go in addition to these points and may not be below these values. Also should not allow the existence of any inflection point.
Through my code is possible to have na idea of what I need, but how can see, I couldn't avoid the inflection points and my function is not well as all least points.
Another issue: I this code inters = Solve[a[x] == b, x], how so I use the value rational and positive? The code inters = x /. inters[[4]], the value $4$ insert manually.
Clear["Global`*"];
a[x_]:=0.00005x^4-0.01x^3+0.68x^2-23.087x+322;
ent=Range[0,20,2];
xinit=a[#]&/@ent//MatrixForm;
point1={ent[[#]],xinit[[1,#]]}&/@Range[Length[ent]];
offset={10,9.2,8.06,7.09,6.12,5.15,4.18,3.21,2.24,1.27,0.3};
newdata=ent[[#]]+offset[[#]]&/@Range[Length[ent]];
point2={newdata[[#]],xinit[[1,#]]}&/@Range[Length[ent]];
b=Fit[point2,{1,x,x^2,x^3,x^4},x];
inters=Solve[a[x]==b,x]
inters=x/. inters[[4]];
Plot[{a[x],b},{x,0,60},PlotRange->{{0,inters},{0,point1[[1,2]]}},ImageSize->1100,AspectRatio->1,Epilog->{Red,PointSize[0.01],Point[{point1}],Point[{point2}],Black,Point[{x,b}/.Solve[D[b,{x,2}]==0]],Purple,Dashed,Line[{point1[[#]],point2[[#]]}]&/@Range[11]}]