Consider the function $f(x)=\sqrt[3]{x^2}$. To find $f'(x)$, I use the rule $\color{red}{\boxed{\color{black}{\large\big(\sqrt[n]{u^m}\big)'=\frac{mu'}{n\sqrt[n]{u^{n-m}}}}}}$. Therefore $\boxed{\large f'(x)=\frac{2}{3\sqrt[3]{x}}}$. But Wolfram|Alpha says a different thing. See HERE. It says that the derivative is $\large\frac{2x}{3(x^2)^{\frac{2}{3}}}$ which is a different thing. Also, it states that the derivative is $\large\frac{2}{3\sqrt[3]{x}}$ assuming $\boldsymbol{x>0}$ while we know that this is incorrect, because the derivative is $\large\frac{2}{3\sqrt[3]{x}}$ but for all $\boldsymbol x$ in reals, and not assuming $x>0$. What's the problem?
$\begingroup$
$\endgroup$
5
2 Answers
$\begingroup$
$\endgroup$
1
D[CubeRoot[x^2], x]
Assuming[x > 0, Simplify[%]]
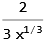
At Wolfram alpha, you asked for cubic root of x^2, which is for x>0. It said Assuming root is the principal root
Reduce[CubeRoot[x^2] == (x)^(2/3)]
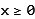
Hence
D[(x)^(2/3),x]
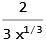
btw, this site is really for questions about Wolfram Mathematica, not Wolfram alpha. These two language are not exactly the same even though they both start with the name Wolfram.
-
$\begingroup$ Worse than that the same result is performed if "Assuming "root" is the real-valued root". $\endgroup$ Commented Feb 27 at 5:56
$\begingroup$
$\endgroup$
W|A and Mathematica agree here. Note that these two functions are not the same; the input in the question is f1[x].
f1[x_] := (x^2)^(1/3)
f2[x_] := (x^(1/3))^2
So the derivatives do not agree. Both are correct though.
{D[f1[x], x], D[f2[x], x]}
(* Out[256]= {(2 x)/(3 (x^2)^(2/3)), 2/(3 x^(1/3))} *)
(I'll make this a Community wiki and also vote to close since it reduces to a misunderstanding of the definition, not to mention off-topic if W|A is the issue.)
Series[D[(x^2)^(1/3), x], {x, 0, 5}]which is(2 x^(4/3))/(3 (x^2)^(2/3) x^(1/3))+O[x]^(16/3)strange? $\endgroup$