I have this equation i would like to solve:
e0 = (2 \[Alpha]1g)/((1 - v1g) (c^2 - b^2))
Integrate[r \[Tau]1[r, t], {r, b, c}] - (2 v1)/E1 A1;
Function e0 represent deformation in vertical (z) axis and based on the equation it is dependant on time t and location r.
Alpha1g, Alpha1, v1g,v1,E1 are material properties and are all known. Equation describes a tube with inner diameter b and outer diameter c, as it is shown on the picture.
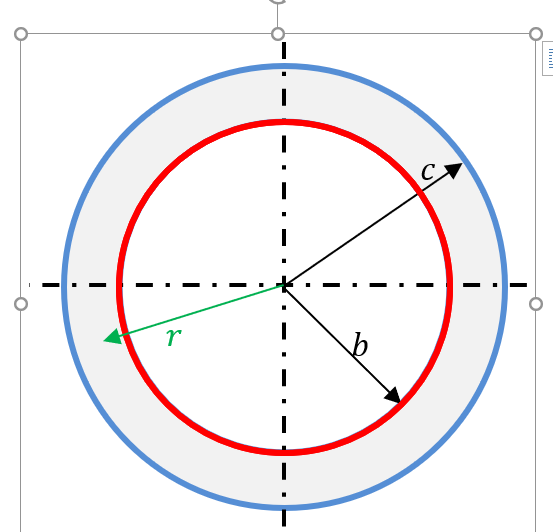
A1 is unknown constant which is determined from other equations and it is not the relevant part of the problem.
Tau[r,t] is temperature function in the tube, dependant on radius and time in form:
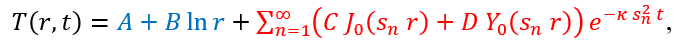
where J0 and Y0 are Bessel function. Temperature function is fully defined. Based on the background of the problem, I know that deformation e0 is a constant and not a function of time. How can i ignore the time component from the definition of equation (code)?
P.S.: Equation for temperature function Tau[r,t]
\[Tau]1[r,t]=-346.514 +
E^(-1.78154 t) (-14.3594 BesselJ[0, 2091.05 r] +
60.4664 BesselY[0, 2091.05 r]) +
E^(-7.14322 t) (42.1879 BesselJ[0, 4187.09 r] -
11.9601 BesselY[0, 4187.09 r]) +
E^(-16.0795 t) (-25.5497 BesselJ[0, 6282.05 r] -
25.0613 BesselY[0, 6282.05 r]) +
E^(-28.5902 t) (-7.80375 BesselJ[0, 8376.73 r] +
29.991 BesselY[0, 8376.73 r]) +
E^(-44.6755 t) (26.7297 BesselJ[0, 10471.3 r] -
7.32848 BesselY[0, 10471.3 r]) +
E^(-64.3353 t) (-17.9762 BesselJ[0, 12565.8 r] -
17.8034 BesselY[0, 12565.8 r]) +
E^(-87.5695 t) (-5.96861 BesselJ[0, 14660.3 r] +
22.6498 BesselY[0, 14660.3 r]) +
E^(-114.378 t) (21.1427 BesselJ[0, 16754.7 r] -
5.74734 BesselY[0, 16754.7 r]) +
E^(-144.762 t) (-14.6534 BesselJ[0, 18849.2 r] -
14.5594 BesselY[0, 18849.2 r]) +
E^(-178.719 t) (-5.01706 BesselJ[0, 20943.6 r] +
18.9434 BesselY[0, 20943.6 r]) +
E^(-216.252 t) (18.035 BesselJ[0, 23038. r] -
4.88343 BesselY[0, 23038. r]) +
E^(-257.359 t) (-12.6799 BesselJ[0, 25132.5 r] -
12.6188 BesselY[0, 25132.5 r]) +
E^(-302.04 t) (-4.41131 BesselJ[0, 27226.9 r] +
16.6113 BesselY[0, 27226.9 r]) +
E^(-350.296 t) (15.9886 BesselJ[0, 29321.3 r] -
4.31963 BesselY[0, 29321.3 r]) +
E^(-402.126 t) (-11.3357 BesselJ[0, 31415.7 r] -
11.292 BesselY[0, 31415.7 r]) +
E^(-457.531 t) (-3.98254 BesselJ[0, 33510.1 r] +
14.9715 BesselY[0, 33510.1 r]) +
E^(-516.51 t) (14.5108 BesselJ[0, 35604.5 r] -
3.91468 BesselY[0, 35604.5 r]) +
E^(-579.064 t) (-10.3447 BesselJ[0, 37698.9 r] -
10.3114 BesselY[0, 37698.9 r]) +
E^(-645.192 t) (-3.65854 BesselJ[0, 39793.3 r] +
13.7377 BesselY[0, 39793.3 r]) - 67.2882 Log[r]
