2
$\begingroup$

I'd like to plot the Poincaré section based on the problem from here: see exercise 8.1

Given that the standard logistic equation with harvesting function is $$\dfrac{dx}{dt} = ax(1 - x) - h (1 + \sin{2 \pi t})$$ with parameters $a >0, h>0$.

From some reading here and also here I try to manipulate some points with $a=5, h=0.8$

Manipulate[{sol, samples} = 
Reap@NDSolve[{x'[t] == 
5*x[t]*(1 - x[t]) - 0.8*(1 + Sin[2*\[Pi]*t]), 
x[0] == 1, WhenEvent[Mod[t, delT] == 0, Sow[{t, x[t]}]]}, 
    x, {t, 10}];

Plot[x[t] /. sol, {t, 0, 10}, Epilog -> {PointSize[Medium], Red, 
    Point @@ samples}], {{delT, .1}, .01, 0.5}, 
 SaveDefinitions -> True]

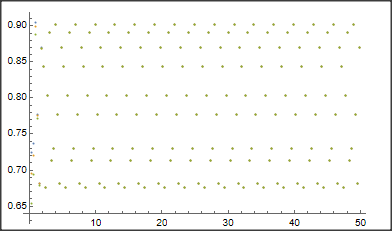
With delT=0.3 I got:

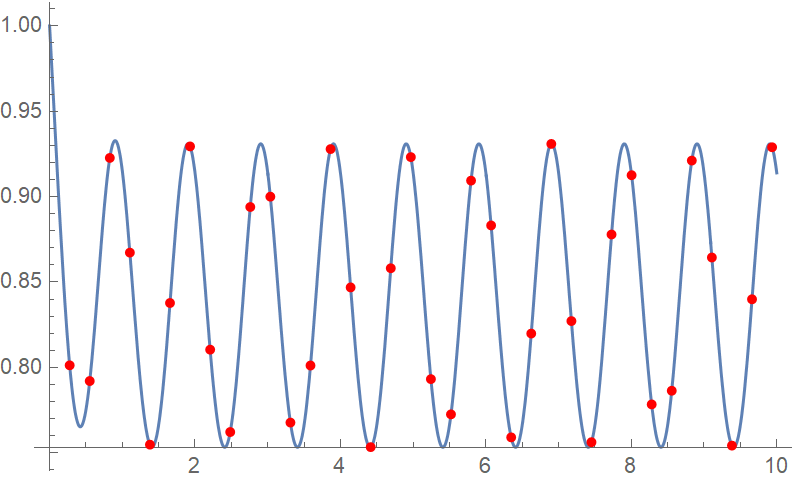
then I started to lost.

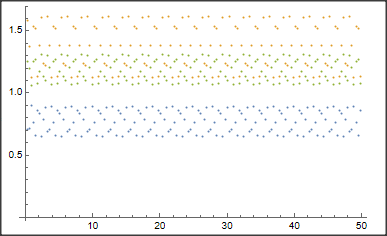
Question 1 How to plot from here? I (try to) allocate the points to map the Poincaré section, but I'm not sure if this is a correct protocol for first order DE

data = {x'[t] == 5* x[t]*(1 - x[t]) - 0.8*(1 + Sin[2*\[Pi]*t])};
psect[{x0_}] := Reap[NDSolve[{data, x[0] == x0 ,
     WhenEvent[Mod[t, 0.3] == 0, Sow[{t, x[t]}]]}, x, {t, 50}]];
adata = Map[psect, {0.92, 0.85, 0.76}];
ListPlot[adata, ImageSize -> Medium]

which I didn't get any result from here.

Question 2 What if, say parameter $h$ is normally distributed on the interval of $[5, 50]$ on each $t$-values. This is motivated from the discussion of logistic with (constant) harvesting in here where @march gave a really nice result using DiscreteVariable

So if I have this distribution as periodic (but random) $h$

delt = {RandomVariate[NormalDistribution[20, 2], 30]};
ListPlot[delt, Filling -> Axis, PlotStyle -> Red, DataRange -> {5, 50}]

which produce

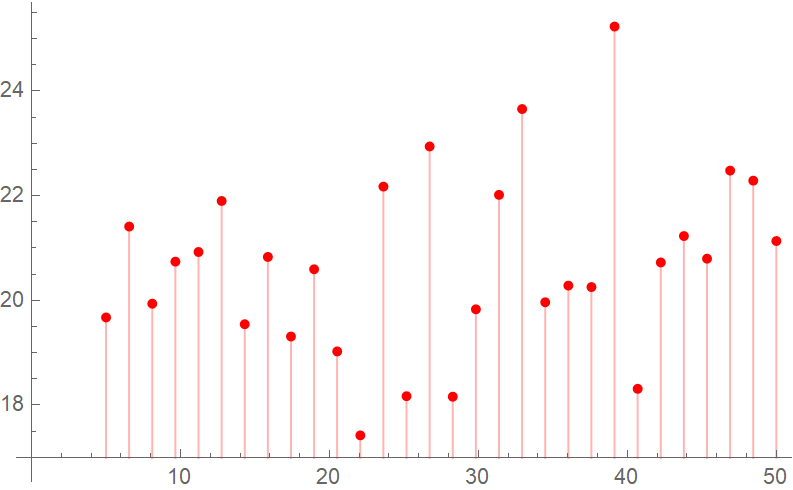
edit Note that, each $t$ on the graph is distributed randomly with interval of 1, which also can be specified using Table.

How can I tabulate this result into $x'[t]$?

I try with WhenEvent and DiscreteVariable but didn't get anything, I might have confuses myself along the way

Improve this question
$\endgroup$
2
  • $\begingroup$ Please don't use LaTeX for diacritics. It makes it impossible to find this post by searching that for that keyword. Use a proper Unicode é. $\endgroup$
    – Szabolcs
    Commented Nov 22, 2021 at 11:54
  • 1
    $\begingroup$ @Szabolcs I wasn't aware about it, thanks for editing it out :) $\endgroup$
    – nightcape
    Commented Nov 22, 2021 at 12:25

2 Answers 2

Reset to default
3
$\begingroup$

Question 1: Read the manual about "Reap". Here is the working code:

data = {x'[t] == 5*x[t]*(1 - x[t]) - 0.8*(1 + Sin[2*\[Pi]*t])};
psect[x0_] := 
  Reap[NDSolve[{data, x[0] == x0, 
      WhenEvent[Mod[t, 0.3] == 0, Sow[{t, x[t]}]]}, x, {t, 50}]][[2, 
    1]];
adata = Map[psect, {0.92, 0.85, 0.76}];

enter image description here

Question 2: MMA can not solve if h is too large. Reducing the mean of h gives (another run will create different output because h is stochastic):

h := RandomVariate[NormalDistribution[1, 2]];
data := {x'[t] == 5*x[t]*(1 - x[t]) - h *(1 + Sin[2*\[Pi]*t])};
psect[x0_] := 
  Reap[NDSolve[{data, x[0] == x0, 
      WhenEvent[Mod[t, 0.3] == 0, Sow[{t, x[t]}]]}, x, {t, 50}]][[2, 
    1]];
adata = Map[psect, {0.92, 0.85, 0.76}];
ListPlot[adata, ImageSize -> Medium]

enter image description here

Improve this answer
$\endgroup$
2
  • $\begingroup$ oh right, I use Reap but didn't specify the value. I realize that the normal distributed $h$ is still producing a single (but random) value every run. But, I'm looking for multiple distributed values of $h$s on each $t$ (like the second picture in my post) on every run, my guessing is I need to create a combination of tabulated arrays of values to create that? I'm still not sure, I'm editing the description on my post to make it clearer $\endgroup$
    – nightcape
    Commented Nov 22, 2021 at 21:05
  • $\begingroup$ I think, every time h is used you get another value. To make this more explicitly, you could write: h[t_] := RandomVariate[NormalDistribution[1, 2]]; $\endgroup$
    – Daniel Huber
    Commented Nov 23, 2021 at 9:10
1
$\begingroup$

For Question 1:

data = {x'[t] == 5*x[t]*(1 - x[t]) - 0.8*(1 + Sin[2*\[Pi]*t])};
solution = 
  Reap[sol = 
     NDSolve[{data, x[0] == 1, 
       WhenEvent[Mod[t, 0.3] == 0, Sow[{t, x[t]}]]}, x, {t, 0, 50}, 
      MaxSteps -> \[Infinity]]][[-1, 1]];
ListPlot[solution]
Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.