1
$\begingroup$

Let me show the code first.

 Gama[kx_, ky_, u_] := NIntegrate[96*Cos[p/2]*(1 + I*kx*Cos[p] + I*ky*Sin[p])^(-7/2)*1/(
6!*Sqrt[Pi])*(((Sqrt[2*u]/(
       Cos[p/2]*(1 + I*kx*Cos[p] + I*ky*Sin[p])^(1/2)))^6 + 
     15/2*(Sqrt[2*u]/(
        Cos[p/2]*(1 + I*kx*Cos[p] + I*ky*Sin[p])^(1/2)))^4 + 
     45/4*(Sqrt[2*u]/(
        Cos[p/2]*(1 + I*kx*Cos[p] + I*ky*Sin[p])^(1/2)))^2 + 15/
     8)*Sqrt[Pi]*
   Exp[(Sqrt[2*u]/(
      Cos[p/2]*(1 + I*kx*Cos[p] + I*ky*Sin[p])^(1/2)))^2]*
   Erfc[Sqrt[2*u]/(
    Cos[p/2]*(1 + I*kx*Cos[p] + I*ky*Sin[p])^(1/2))] - (Sqrt[
    2*u]/(Cos[p/2]*(1 + I*kx*Cos[p] + I*ky*Sin[p])^(1/2)))^5 - 
  7*(Sqrt[2*u]/(
     Cos[p/2]*(1 + I*kx*Cos[p] + I*ky*Sin[p])^(1/2)))^3 - 
  33/4*(Sqrt[2*u]/(
    Cos[p/2]*(1 + I*kx*Cos[p] + I*ky*Sin[p])^(1/2)))), {p, -Pi, 
Pi}];

Then you can use

 Plot[Gama[0, 0, u], {u, 0, 1}, PlotRange -> All]

You may find that the values are very large.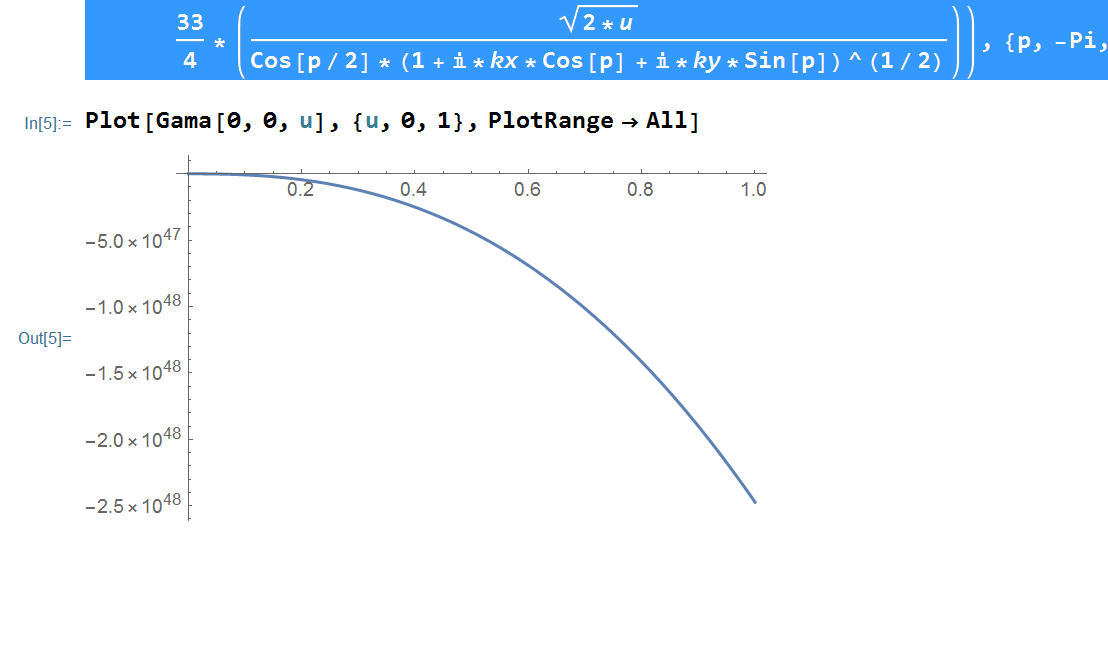enter image description here

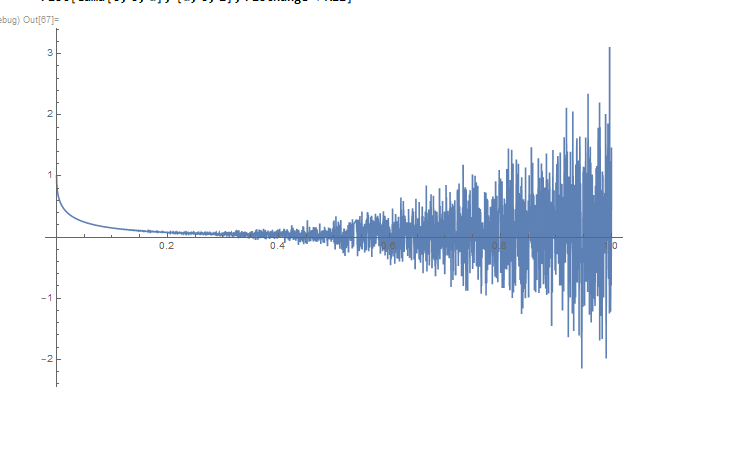
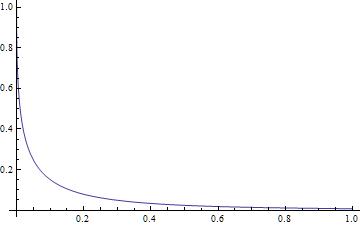
Then I try another computer, I get enter image description here

These days, I am really lucky. Life is tough!

Improve this question
$\endgroup$
12
  • $\begingroup$ Those two graphs look the same to me. What are we looking for? $\endgroup$
    – Chris K
    Commented Jul 12, 2020 at 13:39
  • $\begingroup$ see Fig1 and Fig3 @ChrisK $\endgroup$
    – Blueka
    Commented Jul 12, 2020 at 13:48
  • $\begingroup$ Here I upload three figures. The last two figures are the same. I do not know how to delete one of them. $\endgroup$
    – Blueka
    Commented Jul 12, 2020 at 13:49
  • 1
    $\begingroup$ @flinty version:11.3.0.0 11.2.0.0 $\endgroup$
    – Blueka
    Commented Jul 12, 2020 at 14:43
  • 2
    $\begingroup$ @Blueka The same result I got with versions 12.0.0 (your first picture) and 12.1.1 (your second picture). It means that result is unstable. We can check it just call Table[{u, Gama[0., 0., u]}, {u, .0, 1., .1}]. There are pole at p=Pi/2 and p=-Pi/2. So we should use some strategy to compute this integral. $\endgroup$
    – Alex Trounev
    Commented Jul 12, 2020 at 16:56

1 Answer 1

Reset to default
4
$\begingroup$

It's a question of numerical precision.

Set ky and ky to zero and plot the integrand ii, enlarge working precision and rationalize variable in Plot.

ii[p_, u_] = 
96*Cos[p/2]*(1 + I*kx*Cos[p] + I*ky*Sin[p])^(-7/2)*1/(6!*
   Sqrt[Pi])*(((Sqrt[
         2*u]/(Cos[
           p/2]*(1 + I*kx*Cos[p] + I*ky*Sin[p])^(1/2)))^6 + 
     15/2*(Sqrt[
          2*u]/(Cos[
            p/2]*(1 + I*kx*Cos[p] + I*ky*Sin[p])^(1/2)))^4 + 
     45/4*(Sqrt[
          2*u]/(Cos[
            p/2]*(1 + I*kx*Cos[p] + I*ky*Sin[p])^(1/2)))^2 + 
     15/8)*Sqrt[Pi]*
   Exp[(Sqrt[
        2*u]/(Cos[p/2]*(1 + I*kx*Cos[p] + I*ky*Sin[p])^(1/2)))^2]*
   Erfc[Sqrt[
      2*u]/(Cos[
        p/2]*(1 + I*kx*Cos[p] + I*ky*Sin[p])^(1/2))] - (Sqrt[
      2*u]/(Cos[p/2]*(1 + I*kx*Cos[p] + I*ky*Sin[p])^(1/2)))^5 - 
  7*(Sqrt[2*
        u]/(Cos[p/2]*(1 + I*kx*Cos[p] + I*ky*Sin[p])^(1/2)))^3 - 
  33/4*(Sqrt[
      2*u]/(Cos[
        p/2]*(1 + I*kx*Cos[p] + I*ky*Sin[p])^(1/2)))) /. {kx -> 0,
 ky -> 0} // Simplify[#, -Pi < p < Pi && 0 < u < 1] &

Plot3D[ii[p, u], {p, -Pi, Pi}, {u, 0, 1}, PlotRange -> {0, .1}, 
   PlotPoints -> 50, WorkingPrecision -> 30]

Ga00[u_] := NIntegrate[ii[p, u], {p, -Pi, Pi}, WorkingPrecision -> 30]

Plot[Ga00[Rationalize[u, 0]], {u, 0, 1}, PlotRange -> All]

enter image description here

Improve this answer
$\endgroup$
1
  • $\begingroup$ Cool! Thanks! I get the point. $\endgroup$
    – Blueka
    Commented Jul 13, 2020 at 8:47

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.