Following this question and user21 answered, I changed the BCs of the initial problem. The code following bellow.
<< NumericalDifferentialEquationAnalysis`;
Needs["NDSolve`FEM`"];
G = Rationalize[6.894745 10^9]
E1 = Rationalize[26.25 G]; E2 = Rationalize[1.49 G]; G12 =
Rationalize[1.04 G]; nu12 = Rationalize[0.28]; nu21 = (E2*nu12)/E1;
t = Rationalize[0.0050 .0254];
Son = {{1/E1, -nu12/E1, 0}, {-nu21/E2, 1/E2, 0}, {0, 0, 1/G12}};
Qon = Inverse[Son];
Q11 = Qon[[1, 1]]; Q12 = Qon[[1, 2]]; Q22 = Qon[[2, 2]]; Q66 =
Qon[[3, 3]];
U1 = (3 Q11 + 3 Q22 + 2 Q12 + 4 Q66)/8; U2 = (Q11 - Q22)/
2; U3 = (Q11 + Q22 - 2 Q12 - 4 Q66)/
8; U4 = (Q11 + Q22 + 6 Q12 - 4 Q66)/
8; U5 = (Q11 + Q22 - 2 Q12 + 4 Q66)/8;
alpha = 45 (\[Pi]/180);
a = 1; b = 1; d = a Cos[alpha] + b Sin[alpha];
omega = Rectangle[{0, 0}, {a, b}];
mesh = ToElementMesh[omega, MaxCellMeasure -> 0.001];
u0 = Rationalize[0.01];
angle1 = 10; angle0 = 0;
angles = {{angle0, angle1}, {-angle0, -angle1}, {angle0,
angle1}, {-angle0, -angle1}, {angle0,
angle1}, {-angle0, -angle1}, {-angle0, -angle1}, {angle0,
angle1}, {-angle0, -angle1}, {angle0,
angle1}, {-angle0, -angle1}, {angle0, angle1}};
num = Dimensions[angles][[1]]; h = num*t; pos = Table[0, num + 1];
pos[[1]] = -h/2;
For[i = 2, i <= num + 1, i++, pos[[i]] = pos[[i - 1]] + t];
\[Xi]A = {0, 0, 0, 0, 0};
\[Xi]B = {0, 0, 0, 0, 0};
\[Xi]D = {0, 0, 0, 0, 0};
For[i = 1, i <= num, i++, T0 = angles[[i, 1]];
T1 = angles[[i, 2]];
func[s_] :=
Simplify@((2/d) (T1 - T0) Sqrt[(s - d/2)^2] + T0) (\[Pi]/180);
theta[x_, y_] := alpha + func[x Cos[alpha] + y Sin[alpha]];
zA = pos[[i + 1]] - pos[[i]];
zB = pos[[i + 1]]^2 - pos[[i]]^2;
zD = pos[[i + 1]]^3 - pos[[i]]^3;
V1 = Cos[2 theta[x, y]]; V2 = Sin[2 theta[x, y]];
V3 = Cos[4 theta[x, y]]; V4 = Sin[4 theta[x, y]];
\[Xi]a = {1, V1, V2, V3, V4} zA;
\[Xi]b = {1, V1, V2, V3, V4} zB;
\[Xi]d = {1, V1, V2, V3, V4} zD;
\[Xi]A = \[Xi]A + \[Xi]a;
\[Xi]B = \[Xi]B + \[Xi]b;
\[Xi]D = \[Xi]D + \[Xi]d;];
mU = {{U1, U2, 0, U3, 0}, {U4, 0, 0, -U3, 0}, {U1, -U2, 0, U3, 0}, {0,
0, U2/2, 0, U3}, {0, 0, U2/2, 0, -U3}, {U5, 0, 0, -U3, 0}};
mA = mU.\[Xi]A; mB = (mU.\[Xi]B)/2; mD = (mU.\[Xi]D)/3;
A11[x_, y_] = mA[[1]]; A12[x_, y_] = mA[[2]]; A16[x_, y_] = mA[[4]];
A22[x_, y_] = mA[[3]]; A26[x_, y_] = mA[[5]]; A66[x_, y_] = mA[[6]];
D11[x_, y_] = mD[[1]]; D12[x_, y_] = mD[[2]]; D16[x_, y_] = mD[[4]];
D22[x_, y_] = mD[[3]]; D26[x_, y_] = mD[[5]]; D66[x_, y_] = mD[[6]];
Nx[x_, y_] =
A11[x, y] D[u[x, y], {x, 1}] + A12[x, y] D[v[x, y], {y, 1}] +
A16[x, y] (D[u[x, y], {y, 1}] + D[v[x, y], {x, 1}]);
Ny[x_, y_] =
A12[x, y] D[u[x, y], {x, 1}] + A22[x, y] D[v[x, y], {y, 1}] +
A26[x, y] (D[u[x, y], {y, 1}] + D[v[x, y], {x, 1}]);
Nxy[x_, y_] =
A16[x, y] D[u[x, y], {x, 1}] + A26[x, y] D[v[x, y], {y, 1}] +
A66[x, y] (D[u[x, y], {y, 1}] + D[v[x, y], {x, 1}]);
PDEs = {D[Nx[x, y], {x, 1}] + D[Nxy[x, y], {y, 1}],
D[Ny[x, y], {y, 1}] + D[Nxy[x, y], {x, 1}]};
gammaD = {DirichletCondition[{v[x, y] == u0}, y == 0],
DirichletCondition[{v[x, y] == -u0}, y == b]};
omega = Rectangle[{0, 0}, {a, b}];
mesh = ToElementMesh[omega, MaxCellMeasure -> 0.001];
PDEs = FullSimplify[PDEs];
{U, V} = NDSolveValue[{PDEs == {0, 0}, gammaD,
DirichletCondition[u[x, y] == 0, x == a/2]}, {u,
v}, {x, y} \[Element] mesh];
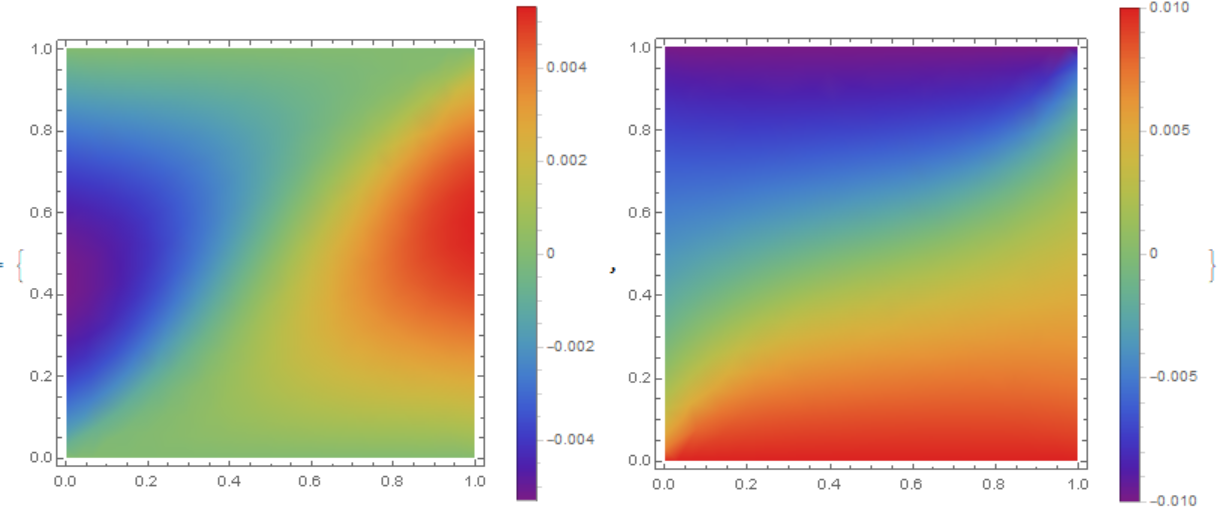
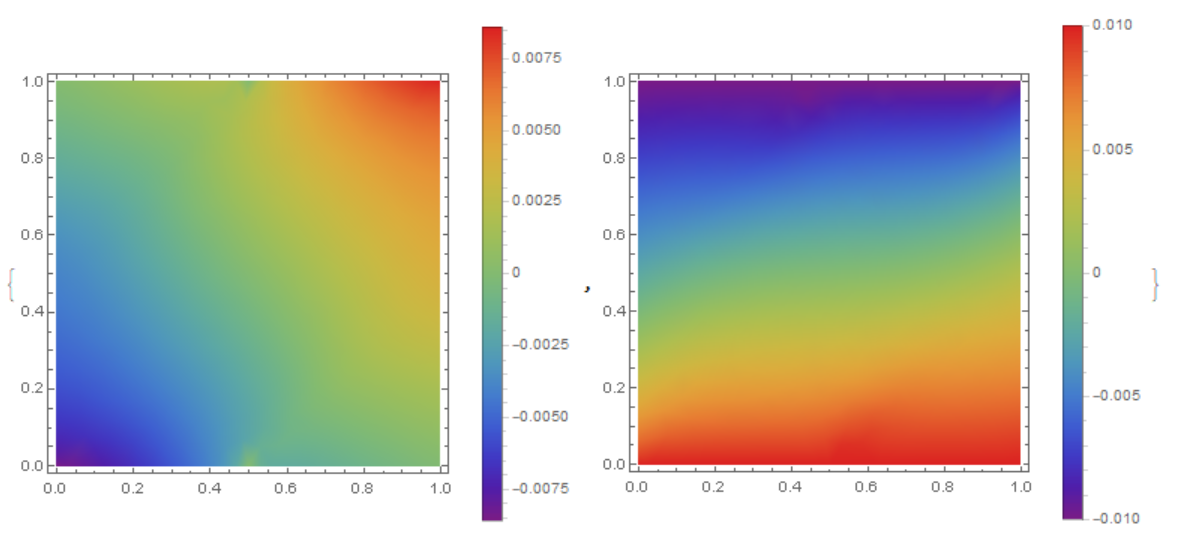
Plotting it the solution for U[x,y], it's possible to see two peaks.
Differentiation of x (D[U[x, y], {x, 1}]) potentiates these peaks as can be seen bellow
These are not expected results. Would anyone know the reason of these peaks? I've been tried to refine the mesh (~ 10000 quad elements) and these peaks keep happening. Am I losing some important thing here?

![U[x,y] plot](https://i.sstatic.net/X4E9W.png)
![D[U[x,y],x](https://i.sstatic.net/MqaOZ.png)