Clustering a set of points

Question

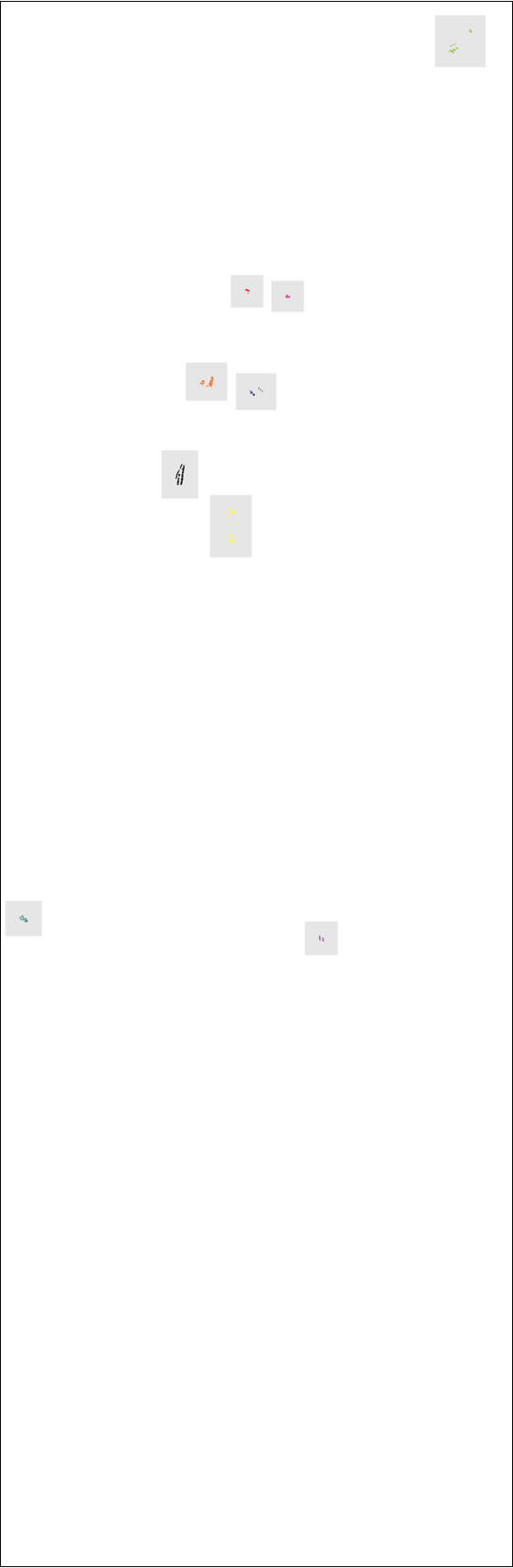
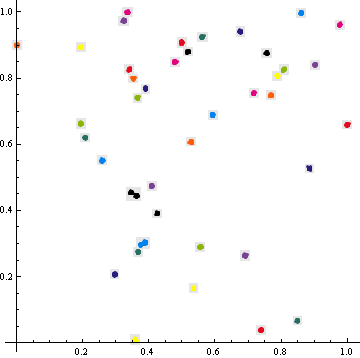
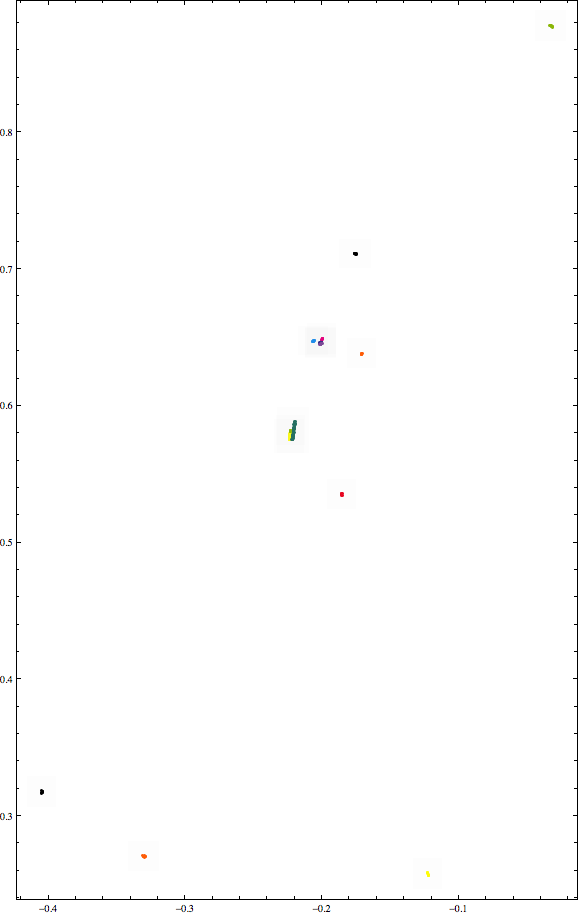
I have a set of 2D points in the square defined by {-1, -1} and {1, 1}. These points typically form compact groups. I need to break them into clusters in such a way that the rectangular bounding boxes of the clusters will not overlap. The bounding boxes are expanded by a pre-specified margin, denoted dist.

I managed to implement this by computing the pairwise Manhattan distance, building a corresponding graph and taking the connected components of the graph (see attached code).

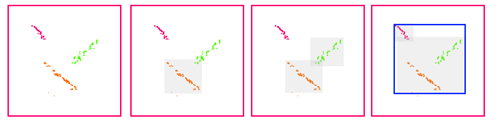
I was hoping that there would be a simper solution which avoids computing the complete pairwise distance matrix. I tried using FindClusters, but not having any experience with the underlying methods I did not manage to get it to return the appropriate number of clusters (it typically lumps everything together, even when points are "visually" separate). So the question is: Is it possible to implement this using FindClusters? The key is in choosing the correct Method option for FindClusters, which is unfortunately not documented in a way that's easy to understand for someone not familiar with these methods.

Requirements: The clustering does not need to be precise. If the method returns a bit fewer clusters than what I show in the image below, that's okay. I need the results for a heuristic decision anyway. But it should not lump together things which are rather far compared to the size of the visually perceived clusters. It is very easy for us humans to recognize these clusters, and I'd like to get the computer to give me same output one would naturally construct by hand after looking at the image. All points sets I have have a very similar structure to the one I show below, but the groups may have different size scales. This is why it makes sense to ask "I'd like to have the clusters similar to what I perceive visually". The method must work without any user intervention (manual estimation of parameters).

---

pts = Import["http://ge.tt/api/1/files/7sHEVob/0/blob?download", "WDX"];

dist = 0.01;

comp = ConnectedComponents@
   AdjacencyGraph[
    UnitStep[2 dist - Outer[ManhattanDistance, pts, pts, 1]]];

Graphics@MapIndexed[
  With[{p = pts[[#1]]}, {{GrayLevel[.9], 
      Rectangle[{Min[p[[All, 1]]], Min[p[[All, 2]]]} - 
        dist, {Max[p[[All, 1]]], Max[p[[All, 2]]]} + 
        dist]}, {ColorData[3][First[#2]], Point[p]}}] &, comp]

Click for a larger image:

Answer 1

This is roughly 30 times faster than your approach and can be tuned easier than FindClusters[]:

getOneCluster[pts_List, maxDist_?NumericQ] :=(*Returns a cluster*)
 Module[{f},
  f = Nearest[pts];
  FixedPoint[Union@Flatten[f[#, {Infinity, maxDist}] & /@ #, 1] &, {First@pts}]]
clusters[data_] := Module[{f, dist},
  (* Some Characteristic Distance, assuming no isolated points*)
  f = Nearest[data];
  dist = 3 Max[EuclideanDistance[Last@f[#, 2], #] & /@ data];
  Flatten[Reap[NestWhile[Complement[#, Sow@getOneCluster[#, dist]] &, data, 
                         # != {} &]][[2]], 1]
  ]

(* Gen some data *)

SeedRandom[42];
numberOfClusters = 42;
clustersCenters = RandomReal[{0, 1}, {numberOfClusters, 2}];
data = Flatten[RandomVariate[BinormalDistribution[#, .002 {1, 1}, .1], 100] & /@ 
                            clustersCenters, 1];
pad = .01;

Plotting the results:

Graphics[MapIndexed[With[{p = #1}, {{GrayLevel[.9], 
                   Rectangle[{Min[p[[All, 1]]], Min[p[[All, 2]]]} - pad, 
                             {Max[p[[All, 1]]], Max[p[[All, 2]]]} + pad]}, 
                             {ColorData[3][First[#2]], Point[p]}}] &, clusters[data]], 
         Axes -> True]

The problem with "merging" those clusters so that the bounding boxes don't overlap needs some heuristic and I think it should better be done as a post-processing step. The caveat is that the merging process done blindly (and worst, recursively) can aggregate much more points than seems reasonable. Take a look:

Answer 2

You can get the same results with:

FindClusters[pts, Method -> {"Agglomerate", "Linkage" -> "Complete", 
                             "SignificanceTest" -> {"Gap", "Tolerance" -> 3}}]

But it is impossible to test its significance until you post more point sets.

Answer 3

If you don't want to compute every pair-wise distance, one thing is to compute the Delaunay triangulation of all the points in the sets, this tends to be only ${\cal{O}}(n \log n )$ computation intesive.

We will use the ComputationalGeometry package for the Delauny triangulation. There are other faster options described in this site, also this package does not do 3-dimensional points triangulation, there are some alternatives also described on the site.

Clustering is usually done by setting a minimal distance between points. This tend however to cause problems, for the case of clusters that are connected by single/few points etc. One way to deal with this is to cluster based on density.

DBSCAN

This is an implementation of the DBSCAN. Here, the idea is to give a minimum distance and a minimal number of points in that distance. Hence you give a density. Any locations that have a density lower than that, will remain unclustered, and are considered noise. So it could also be a problem if you don't want to "lose" any points.

The first part is an implementation of a Breath-First Scan code, probably using mathematica's own BFS might be faster. All parts of the code could make due with a rewriting for efficiency, this was manly written for my education than anything else. So if you find a way to make it faster, do let me know!! please.

MyDistance[x_, y_, points_] := 
  Sqrt[ #[[1]]^2 + #[[2]]^2] &@(points[[x]] - points[[y]]);
CountBreadthFirst2[adjgraph_List, point_Integer, dmax_Real, allpoints_] := 
    Module[{queue = {point},marked = ConstantArray[False, Length[adjgraph]],
             visit, dis,a},
a = Last@Reap[
  While[Length[queue] != 0,
    visit = First@queue;
    marked[[point]] = True;
    Scan[Function[{fx},
      If[! marked[[fx]],
                   marked[[fx]] = True;
                   dis = MyDistance[fx, point, allpoints];
                   If[dis <= dmax,
                             Sow[fx];
                 queue = Append[queue, fx];
                ];      
        ];
      ], adjgraph[[visit]][[2]]];
    queue = Delete[queue, 1];
    ];];
 If[Length[a] == 0, Return[{}];, Return[First@a];];
  ];

The second part is the actual DBSCAN code, where eps is minimal distance, and npoints is the minimal number of points that need to be found within that distance.

 DBSCAN[allpoints_, eps_, npoints_, adjgraph_] := 
     Module[{marked,clustered,clustcount,neighborp,visit,neighborpprime, i},

     marked = ConstantArray[False, Length[adjgraph]];
     clustered = ConstantArray[False, Length[adjgraph]];
     clustcount = 0;
  Return[Last@Reap[
  For[i = 1, i <= Length@allpoints, i++,
    If[marked[[i]], Continue[];,

      marked[[i]] = True;

      neighborp = 
       CountBreadthFirst2[adjgraph, i, eps, allpoints];
      If[Length[neighborp] < npoints,
         Sow[allpoints[[i]], "Noise"];,
       clustcount++;
       Sow[allpoints[[i]], clustcount];
       clustered[[i]] = True;

       While[Length@neighborp > 0,
        visit = First@neighborp;
         If[! marked[[visit]],
         marked[[visit]] = True;

         neighborpprime = 
          CountBreadthFirst2[adjgraph, visit, eps, allpoints];
            If[Length[neighborpprime] >= npoints,

          neighborp = 
            DeleteDuplicates@Join[neighborp, neighborpprime];
                   ];
           ];

        If[! clustered[[visit]], 
         Sow[allpoints[[visit]], clustcount]; 
         clustered[[visit]] = True;];
        neighborp = Delete[neighborp, 1];
        ];

       ];
      ];
    ];
  ]];
 ];   

We then apply the Delaunay triangulation, with a visualization.

points=Import["http://ge.tt/api/1/files/7sHEVob/0/blob?download", "WDX"];
myadj = DelaunayTriangulation[points];

ee = DeleteDuplicates[Sort[#] & /@ ((#[[1]] -> #[[2]]) & /@ (Flatten[
    Thread[#] & /@ myadj, 1]))];
GraphPlot[ee,VertexCoordinateRules -> MapIndexed[Last@#2 -> #1 &, pts], 
           Frame -> True, FrameTicks->True]

Then apply the DBSCAN code, with say at least 5 points within 0.001:

clustersp = DBSCAN[pts, 0.001, 5, myadj];
Length[clustersp]-1

>14

We actually find some extra clusters! The periphery points remain unclustered. Note that the current code, puts all the "noise" points in the first part of the output of clustersp. Also note that npoints=2 is just the specific case you had before.

Graphics[MapIndexed[
   With[{p = #1}, {{GrayLevel[.9], Opacity[0.05], 
  Rectangle[{Min[p[[All, 1]]], Min[p[[All, 2]]]} - 
    0.01, {Max[p[[All, 1]]], Max[p[[All, 2]]]} + 
    0.01]}, {ColorData[3][First[#2]], Point[p]}}] &, 
    clustersp[[2 ;;]]], Axes -> True, Frame -> True]

Answer 4

Another way to approach this is to use some of the image processing tools. For example:

pts = Import["http://ge.tt/api/1/files/7sHEVob/0/blob?download", "WDX"];
img = Rasterize[ListPlot[pts, AspectRatio -> 1, Axes -> False], ImageSize -> 1000];
blocks = ColorNegate[Erosion[img, 10]];
ComponentMeasurements[blocks, "BoundingBox"]

{1 -> {{563., 792.}, {596., 817.}}, 2 -> {{678., 789.}, {712., 813.}},
 3 -> {{433., 729.}, {492., 758.}}, 4 -> {{577., 723.}, {632., 751.}}, 
 5 -> {{364., 663.}, {409., 699.}}, 6 -> {{503., 624.}, {562., 669.}}, 
 7 -> {{774., 356.}, {808., 382.}}}

provides a list of the locations of the various clusters. The method is simple enough. The first line makes a rasterized image of the points. The individual separated points are collected into blocks using the Erosion command. This can be easily visualized:

MorphologicalComponents[blocks] // Colorize 

The locations of the bounding boxes are then found using the ComponentMeasurements command.

Since the OP isn't looking for a precise answer but rather something "similar to what I perceive visually," using the image processing tools might be sensible. The two parameters at play are the size of the rasterization (I chose to make the image 1000-by-1000) and the amount of erosion (smaller will tend to detect more blocks, greater will tend to merge blocks if they are close).