How to include measurement error in FindDistributionParameters (using maximum likelihood)?

Question

I am trying to fit a non-standard PDF to data using FindDistributionParameters. My custom distribution is defined like this:

fs = Function[{so,l, n},
   TransformedDistribution[
    so - l/2 c,
    c \[Distributed] 
     NoncentralChiSquareDistribution[n, 2 so/l],
     Assumptions -> so > 0 && n > 0 && l > 0
    ]
   ];

My data corresponds to measurements of a variable on N individuals. For each individual I have multiple measurements to take into account the measurement error. My data is similar to this :

SeedRandom[1];
trueValue = RandomVariate[fs[so, l, n] /. {so -> 1, l -> 0.1, n -> 2}, 100];(* true value for each individual, which I don't know*)
errorSD = RandomVariate[ChiSquareDistribution[3], 100]/10; (*standard deviations of the measurment error for each individual*)

fullMeasurement = RandomVariate[NormalDistribution[#[[1]], #[[2]]], 5] & /@ Transpose[{trueValue, errorSD}];
myData = {Mean[#], StandardDeviation[#]} & /@ fullMeasurement(*Simulated data set similar to my real data*)

As a first approach, I used FindDistributionParameters on the mean of the measurements.

p = FindDistributionParameters[myData[[All,1]],
   fs[so, l, n],
   ParameterEstimator -> {"MaximumLikelihood", Method -> "NMaximize",
     MaxIterations -> 500, AccuracyGoal -> 5}];

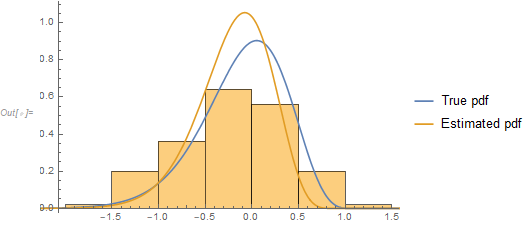
Show[
 Histogram[myData[[All,1]], 15, "ProbabilityDensity"],
 Plot[PDF[fs[so,l, n] /. p, x], {x, -2, 1}, PlotRange -> All, PlotStyle -> Thick]
 ]

However with this approach I am not using the information of the standard deviation of the measurements. As a second approach, assuming the error is normally distributed, I tried to make a convolution of my custom distribution and a normal distribution. I first tried to get the PDF of the convolution using either Convolve or directly integrating with Integrate but it did not work.

Then tried to use TransformedDistribution to create the convolution and use FindDistributionParameters:

fse = Function[{so, l, n, sd},
   TransformedDistribution[
    so - l/2 c + e,
    {c \[Distributed] NoncentralChiSquareDistribution[n, 2 so/l],
     e \[Distributed] NormalDistribution[0, sd]},
    Assumptions -> so > 0 && n > 0 && l > 0 && sd > 0
    ]
   ];

FindDistributionParameters[data,
  fse[so, l, n, sd] /. sd -> Mean[data[[All,2]]],
  ParameterEstimator -> {"MaximumLikelihood", Method -> "NMaximize", 
    MaxIterations -> 500, AccuracyGoal -> 5}];

However it runs indefinitely, and this approach is using only the mean of the standard deviations and not the value for each individual. Is their a better (and more efficient) way to include the measurement error in the estimation method ?

---

EDIT: Following @JimB comment, below is the code I used to try to estimate parameters with fse using data that were directly generated from it:

data = RandomVariate[fse[1, 0.1, 2, 0.01], 100];
FindDistributionParameters[data, fse[so, l, n, sd] /. sd -> 0.01, 
  ParameterEstimator -> {"MaximumLikelihood", Method -> "NMaximize", MaxIterations -> 500, AccuracyGoal -> 5}];

It also runs indefinitely

Answer 1

I led you astray with a bogus answer that I didn't think through appropriately. I've removed the previous answer and put in place a more correct approach. Here's what I missed: the variance associated with the added noise needs to be estimated with the replicate measurements. If just the mean of the replicates is used, then all of the information about the noise variance is essentially lost.

The Method of Moments will still work here. Standard errors could be obtained by bootstrapping. However, Mathematica complains if FindDistributionParameters is used because it can't determine the support of the distribution. Fortunately it is simple to still use the method of moments without FindDistributionParameters.

(* Define distributions *)
fs = Function[{so, l, n}, 
   TransformedDistribution[so - l/2 c, 
    c \[Distributed] NoncentralChiSquareDistribution[n, 2 so/l], 
    Assumptions -> so > 0 && n > 0 && l > 0]];

fse = Function[{so, l, n, σ}, 
   TransformedDistribution[so - l/2 c + e,
    {c \[Distributed] NoncentralChiSquareDistribution[n, 2 so/l], 
     e \[Distributed] NormalDistribution[0, σ/Sqrt[5]]}]];

(* Distribution of observed data with replicate measurments *)
fse2 = Function[{so, l, n, σ}, 
  TransformedDistribution[so - l/2 c + {e1, e2, e3, e4, e5}, 
  {c \[Distributed] NoncentralChiSquareDistribution[n, 2 so/l], 
  e1 \[Distributed] NormalDistribution[0, σ], e2 \[Distributed] NormalDistribution[0, σ], 
  e3 \[Distributed] NormalDistribution[0, σ], e4 \[Distributed] NormalDistribution[0, σ], 
  e5 \[Distributed] NormalDistribution[0, σ]}]];

Now generate data and estimate parameters.

(* Get data with replicate measurements *)
SeedRandom[1];
xx = RandomVariate[fse2[1, 0.1, 2, 1], 100];

(* Estimate standard deviation for replicates *)
sd = Mean[Variance[#] & /@ xx]^0.5
(* 0.98078 *)

(* Get mean for each set of replicates *)
x = Mean[#] & /@ xx;

(* Use method of moments where theoretical moments are set to the sample moments *)
mom = NSolve[{Mean[fse[so, l, n, sd]] == Mean[x],
    Variance[fse[so, l, n, sd]] == Variance[x],
    Skewness[fse[so, l, n, sd]] == Skewness[x]}, {so, l, n}];
(* Keep only the legitimate solutions *)
mom = Select[mom, (so /. # ) > 0 && (l /. #) > 0 && (n /. #) > 0 &] //
   Flatten
(* {so -> 0.847071, n -> 4.853, l -> 0.0808586} *)

(* Now plot observed histogram, theoretical pdf and estimated pdf *)
Show[Histogram[x, Automatic, "PDF"], 
 Plot[{PDF[fs[1, 0.1, 2], z], PDF[fs[so, l, n, sd] /. mom, z]}, {z, -3, 2}]]