9
$\begingroup$

I have a list of 120 elements of the form $\{q,x,y,z\}$. In fact, each element of the represents the nuclear charge and 3 coordinates of an atom of some large molecule. 

qxyz={{6, 6.10835, -0.710283, 0.211502}, {6, 7.34167, -1.43322, 0.}, {6, 7.32715, -2.79984, 0.126901}, {6, 6.11632, -3.53126, 0.296102}, {6,4.89643, -2.82695, 0.338403}, {6, 4.89915, -1.40962, 0.296102}, {6, 6.08831, -4.94558, 0.126901}, {6, 4.86614, -7.09492, -0.465304}, {6,4.91204, -5.64146, 0.}, {6, 3.6693, -4.93485, 0.211502},
{6,3.67034,-3.53798, 0.296102},{6, 8.57745, -0.666746, -0.465304}, {6, 6.08831, 4.94558, 0.126901}, {6,4.91204, 5.64146, 0.}, {6, 4.86614, 7.09492, -0.465304}, {6, 6.11632, 3.53126, 0.296102}, {6, 7.32715, 2.79984, 0.126901}, {6, 7.34167, 1.43322, 0.}, {6, 6.10835, 0.710283, 0.211502}, 
{6, 4.89915, 1.40962, 0.296102}, {6, 4.89643, 2.82695, 0.338403}, {6,3.67034, 3.53798, 0.296102}, {6, 3.6693, 4.93485, 0.211502}, {6,8.57745, 0.666746, -0.465304}, {6, -1.23884, 7.74542, 0.126901}, {6,0., 7.06252, 0.296102}, {6, 1.23884, 7.74542, 0.126901}, {6, 0., 5.65391, 0.338403}, {6, -1.22881, 4.94759, 0.296102}, {6, -2.43905, 5.64513, 0.211502}, 
{6, -2.42963, 7.07468, 0.}, {6, -3.71131, 7.76166, -0.465304}, {6, 3.71131, 7.76166, -0.465304}, {6, 2.42963, 7.07468, 0.}, {6, 1.22881, 4.94759, 0.296102}, {6, 2.43905, 5.64513,0.211502}, {6, -6.08831, 4.94558, 0.126901}, {6, -7.32715, 2.79984,0.126901}, {6, -6.11632, 3.53126, 0.296102}, {6, -7.34167, 1.43322,0.}, {6, -8.57745, 0.666746, -0.465304}, {6, -4.86614, 7.09492, -0.465304}, {6, -4.91204, 5.64146, 0.}, 
{6, -3.6693, 4.93485, 0.211502}, {6, -3.67034, 3.53798, 0.296102}, {6, -4.89643, 2.82695, 0.338403}, {6, -6.10835, 0.710283, 0.211502}, {6, -4.89915,1.40962, 0.296102}, {6, -8.57745, -0.666746, -0.465304}, {6, -7.34167, -1.43322, 0.}, {6, -7.32715, -2.79984, 0.126901}, {6, -6.11632, -3.53126,0.296102}, {6, -6.08831, -4.94558,0.126901}, {6, -6.10835, -0.710283, 0.211502}, {6, -4.89915, -1.40962, 0.296102}, {6, -4.89643, -2.82695, 0.338403}, {6, -3.67034, -3.53798, 0.296102}, 
{6, -3.6693, -4.93485,0.211502}, {6, -4.91204, -5.64146, 0.}, {6, -4.86614, -7.09492, -0.465304}, {6, -1.22881, -4.94759,0.296102}, {6, 0., -5.65391, 0.338403}, {6, 0., -7.06252, 0.296102}, {6, -1.23884, -7.74542, 0.126901}, {6, -2.42963, -7.07468, 0.}, {6, -2.43905, -5.64513, 0.211502}, {6, 1.23884, -7.74542, 0.126901}, {6, -3.71131, -7.76166, -0.465304}, {6, 1.22881, -4.94759, 0.296102}, {6,2.43905, -5.64513, 0.211502}, 
{6, 2.42963, -7.07468, 0.}, {6,3.71131,-7.76166, -0.465304}, {6, -1.22943, 3.52325, 0.0423004}, {6, 0., 2.81538, -0.253802}, {6, 0.,1.418, -0.676806}, {6, -1.22802, 0.708999, -0.676806}, {6, -2.43819,1.40769, -0.253802}, {6, -2.43651, 2.82635, 0.0423004}, {6,1.22802, 0.708999, -0.676806}, {6, -1.22802, -0.708999, -0.676806},{6, 0., -1.418, -0.676806}, {6, 1.22802, -0.708999, -0.676806}, {6,0., -2.81538, -0.253802}, 
{6, -1.22943, -3.52325,0.0423004}, {6, -2.43651, -2.82635,0.0423004}, {6, -2.43819, -1.40769, -0.253802}, {6, -3.66594,-0.696905, 0.0423004}, {6, -3.66594, 0.696905, 0.0423004}, {6,1.22943, 3.52325, 0.0423004}, {6, 2.43651, 2.82635, 0.0423004}, {6,2.43819, 1.40769, -0.253802}, {6, 3.66594, 0.696905, 0.0423004}, {6,3.66594, -0.696905, 0.0423004}, {6, 2.43819, -1.40769, -0.253802}, {6, 1.22943, -3.52325,0.0423004}, {6, 2.43651, -2.82635, 0.0423004}, {1, 5.79985,7.62243, -0.888307}, {1, 7.01997, 5.47808, -0.0423004}, {1, 8.25414,3.34043, -0.0423004}, 
{1, 9.50115, 1.21161, -0.888307}, {1, 9.50115, -1.21161, -0.888307}, {1,8.25414, -3.34043, -0.0423004}, {1, 7.01997, -5.47808, -0.0423004}, {1, 5.79985, -7.62243, -0.888307}, {1,3.70129, -8.83404, -0.888307}, {1,1.23417, -8.81851, -0.0423004}, {1,-1.23417, -8.81851,-0.0423004}, {1, -3.70129, -8.83404, -0.888307}, {1,-5.79985,-7.62243, -0.888307}, {1, -7.01997, -5.47808, -0.0423004}, {1,-8.25414, -3.34043, -0.0423004}, {1, -9.50115, -1.21161, -0.888307},{1,-9.50115, 1.21161, -0.888307}, 
{1, -8.25414, 3.34043, -0.0423004}, {1,-7.01997,5.47808, -0.0423004}, {1, -5.79985, 7.62243, -0.888307}, {1,-3.70129, 8.83404, -0.888307}, {1, -1.23417, 8.81851, -0.0423004}, {1,1.23417, 8.81851, -0.0423004}, 
{1, 3.70129, 8.83404, -0.888307}};

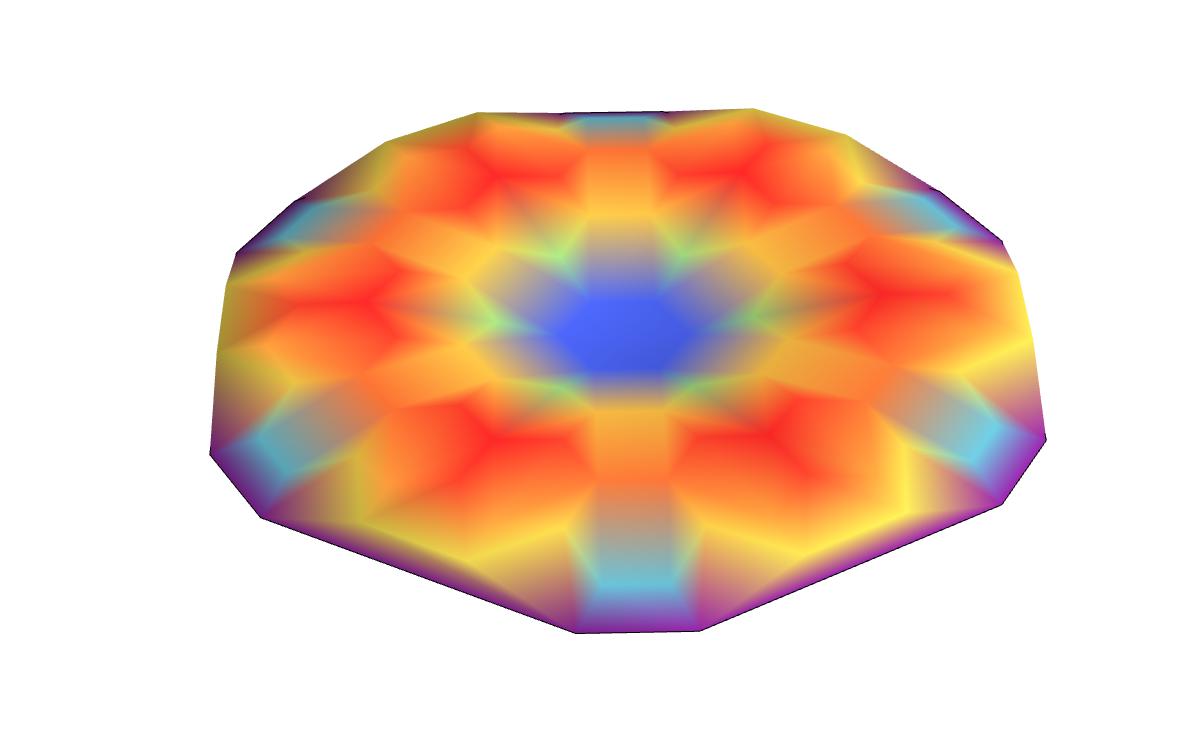
Let us select only the coordinates and plot the surface containing them using the 1st order interpolation:

xyz = qxyz[[All, 2 ;; 4]];
fig=ListPlot3D[xyz, 
     Mesh -> None, BoxRatios -> Automatic, Boxed -> False, 
     Axes -> False, InterpolationOrder -> 1, ColorFunction -> "Rainbow"]

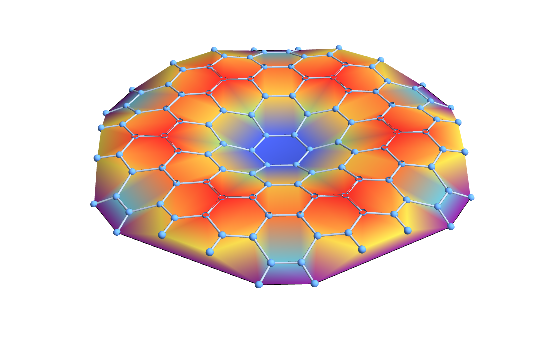
The result looks like that

surface

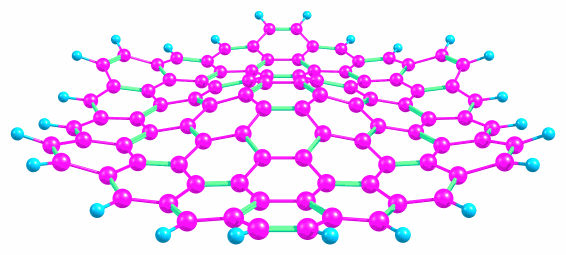
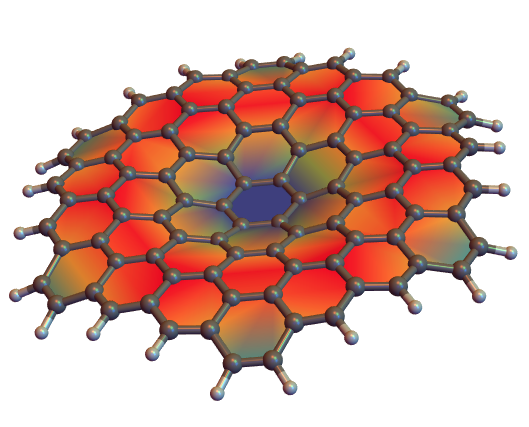
Just to give you an idea how the molecule looks like

molecule

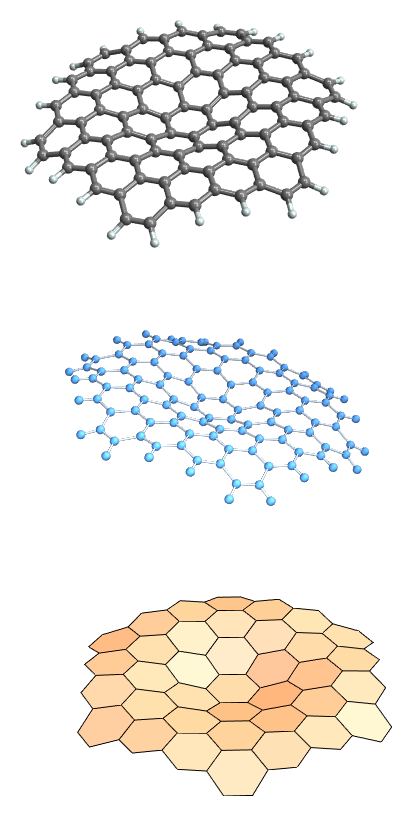
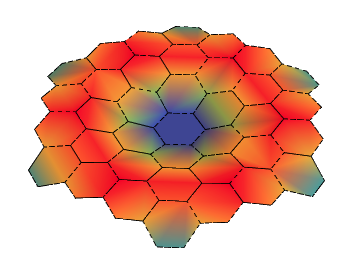
Now comes the question. I like the surface plot because it nicely depicts the vibrational mode. However, I want the surface to be composed to hexagons, like in the second picture. The hexagons' color should be a function of the hexagon's face normal, like we see in the ListPlot3D. How can I achieve this effect ? Thank you in advance.

Improve this question
$\endgroup$
2
  • 1
    $\begingroup$ Some of your hexagons don't lie in a single plane, and therefore the face doesn't have a well defined normal - the hexagon will have a crease down the middle. $\endgroup$
    – Quantum_Oli
    Commented Feb 10, 2017 at 23:09
  • $\begingroup$ @Quantum_Oli Indeed, good observation. Hopefully the creases will be not too pronounced $\endgroup$
    – yarchik
    Commented Feb 10, 2017 at 23:18

2 Answers 2

Reset to default
13
$\begingroup$

(grabbing a lot of code from this answer)

This is just to get you started, adding in colors to the final GraphicsComplex should be pretty easy. 

xyzString = ExportString[
   qxyz /. {a_Integer, b___} :> {ElementData[a, "Abbreviation"], b},
   "Table"];
{plot, coords, atoms} = 
  ImportString[
   xyzString, {"XYZ", {"Graphics3D", "VertexCoordinates", 
     "VertexTypes"}}];
bonds = UndirectedEdge @@@ 
   Graphics`MoleculePlotDump`InferBonds[atoms, coords, 40, 25];
vertexlist = Range@Length@atoms;
chemicalGraph = 
  Graph3D[vertexlist, UndirectedEdge @@@ bonds, 
   VertexCoordinates -> coords/200];
cycles = DeleteDuplicates[Flatten[List @@@ #]] & /@ 
   FindCycle[Graph[vertexlist, UndirectedEdge @@@ bonds], {6}, All];
polygons = Graphics3D[
   GraphicsComplex[coords, Polygon[cycles]],
   Boxed -> False];
{plot, chemicalGraph, polygons}

enter image description here

Here's a way to add vertex colors to the polygons

cf = ColorData[{"Rainbow", MinMax[Last /@ coords]}][Last@coords[[#]]] &;
Graphics3D[
 GraphicsComplex[
  coords, {EdgeForm[Dashed], 
   Polygon[#, VertexColors -> Map[cf, #]] & /@ cycles}],
 Boxed -> False]

enter image description here

or as Bob shows you can combine this with a Graphics3D, like the MoleculePlot you get from the XYZ importer,

enter image description here

Improve this answer
$\endgroup$
3
  • $\begingroup$ Nice and quick answer. @Quantum_Oli commented above that some polygons are not flat. Would it mean that the determination of polygons colors/shading is not so trivial? In your solution you let MA set the shading. The result is already good, and I do not think I will be able to invent a better shading. But I am wondering if the colors can be made more saturated? $\endgroup$
    – yarchik
    Commented Feb 10, 2017 at 23:38
  • $\begingroup$ @yarchik - in the edit I used the z-coordinate to color it using the VertexColors option. If you want each polygon to have just one color, that shouldn't be too hard to do $\endgroup$
    – Jason B.
    Commented Feb 10, 2017 at 23:41
  • $\begingroup$ Thanks a lot. The post you liked too is also very interesting and relevant. For the shading, I found another post mathematica.stackexchange.com/questions/130226/… where undocumented internal function Region`Mesh`MeshCellNormals[meshregion, dimension] is used. $\endgroup$
    – yarchik
    Commented Feb 10, 2017 at 23:50
9
$\begingroup$ 
xyz = Rest /@ qxyz;

Show[
 ListPlot3D[xyz,
  Mesh -> None,
  BoxRatios -> Automatic,
  Boxed -> False,
  Axes -> False,
  InterpolationOrder -> 1,
  ColorFunction -> "Rainbow"],
 NearestNeighborGraph[xyz, {All, 1.6}]]

enter image description here

Improve this answer
$\endgroup$
1
  • $\begingroup$ Although it is not exactly the visual form I had in mind, I like your idea of superimposing two objects $\endgroup$
    – yarchik
    Commented Feb 10, 2017 at 23:24

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.