Given the matrix wam:
wam={
{∞, ∞, ∞, ∞, ∞, ∞, 0.180744, ∞, ∞, ∞, ∞, ∞, 0.196146, ∞, ∞, 0.192559},
{∞, ∞, 0.199743, 0.189167, ∞, 0.177828, 0.136293, 0.198179,
0.170862, ∞, ∞, 0.150103, 0.152068, ∞, 0.145293, 0.147801},
{∞, 0.17492, ∞, ∞, ∞, ∞, ∞, 0.196928, ∞, 0.18818, ∞, ∞, ∞, ∞, ∞, ∞},
{∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞},
{∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞},
{∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞},
{0.164114, 0.189904, ∞, ∞, ∞, 0.142879, ∞, 0.173485, ∞, 0.195519, ∞,
0.179716, 0.152131, ∞, ∞, 0.197488},
{0.193476, 0.186542, ∞, 0.196847, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞,
0.184613, ∞, 0.195341, 0.190637},
{∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞},
{0.17967, ∞, ∞, ∞, ∞, 0.165566, ∞, ∞, ∞, ∞, ∞, ∞, 0.16862, ∞, ∞, ∞},
{∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞},
{∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞},
{∞, ∞, ∞, ∞, ∞, 0.183951, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞, ∞},
{∞, ∞, ∞, ∞, ∞, 0.189936, 0.16593, 0.197014, ∞, ∞, ∞, 0.194794, ∞, ∞, ∞, ∞},
{0.189579, 0.167198, ∞, ∞, ∞, 0.18947, ∞, ∞, ∞, 0.187049, ∞, ∞, ∞, ∞, ∞, ∞},
{∞, 0.149854, ∞, ∞, ∞, 0.188494, 0.150641, 0.192737, 0.194964, ∞, ∞, ∞,
0.14314, 0.15716, 0.14968, ∞}
};
I generate the directed graph and its community structure:
vnames = {"AGF", "OIL", "MA1", "MA2", "EGW", "CST", "WHS", "TRS",
"HOT", "INF", "FIN", "EST", "ADM", "EDU", "HLT", "ENT"};
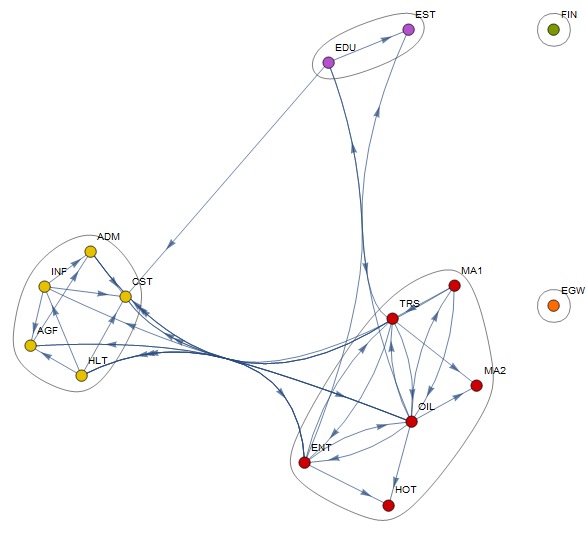
wag = WeightedAdjacencyGraph[vnames, wam, VertexLabels -> "Name",
ImageSize -> 250]
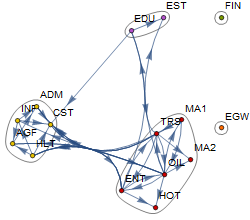
CommunityGraphPlot[wag, FindGraphCommunities[wag]]
Then I delete a vertex from the graph wag and find the communities in the resulting graph:
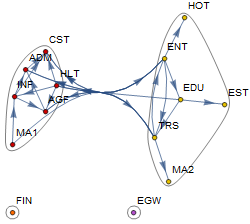
vdwag = VertexDelete[wag, {"WHS"}]
FindGraphCommunities[vdwag]
(* {{"OIL", "MA1", "MA2", "TRS", "HOT", "EST", "EDU", "HLT",
"ENT"}, {"AGF", "CST", "INF", "ADM"}, {"EGW"}, {"FIN"}} *)
Then I wanted to draw the communities using:
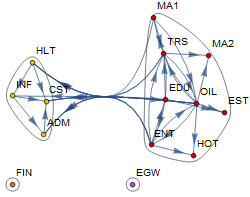
CommunityGraphPlot[vdwag, FindGraphCommunities[vdwag]]
However, this does not work, although vdwag is a graph. WHY?

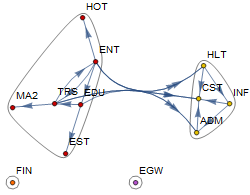
Graph. Ideally, use 12.2. $\endgroup$EdgeWeights are not properly modified byVertexDelete. A workaround: useEdgeDelete, that is, tryedwag = EdgeDelete[wag, DirectedEdge["WHS", _] | DirectedEdge[_, "WHS"]]$\endgroup$IGWeightedVertexDeletewhich can handle weighted graphs properly in v11.3 as well. But it will discard all edge properties except weights. There is alsoIGTakeSubgraph, which handles all properties correctly in versions prior to 12.0 as well, but it is very slow. $\endgroup$IGWeightedAdjacencyGraphandIGWeightedAdjacencyMatrixwhich are actually consistent with each other in the handling of zeros and infinities, unlike the builtinWeightedAdjacencyMatrixandWeightedAdjacencyGraph. $\endgroup$FindGraphCommunities$\endgroup$