I have a simple differential equation $$ \frac{df(T)}{dT} = g(T)(f(T)-f_{\text{eq}}(T)), $$ where $g,f_{\text{eq}}$ are some smooth functions, and $T$ is an analog of time, but the evolution corresponds to its decreasing. The initial condition is fixed at some large $T$.
As far as $g(T)$ is large, $f(T)$ tends to $f_{\text{eq}}(T)$.
The data and interpolations for $g, f_{\text{eq}}$ is given below:
datatable = {{0.001, -18.23707929395402, -3.4611075922132485*^51,
0.06611490804695141},
{0.0012589254117941675, -17.84461494544366, \
-1.731220410720289*^51, 0.06940434750265481},
{0.001584893192461114, -17.431914718313582, \
-8.51731766948239*^50, 0.07237676798672106},
{0.001995262314968879, -17.005104472877587, \
-4.3165099986682115*^50, 0.07395569051393182},
{0.0025118864315095794, -16.557706734429065, \
-2.1593973167434227*^50, 0.07467984975288536},
{0.0031622776601683794, -16.092497328595197, \
-1.074810138034652*^50, 0.07614210743368215},
{0.003981071705534973, -15.612016450430032, \
-5.402227503991311*^49, 0.07670151429807713},
{0.005011872336272725, -15.11979376331143, \
-2.7075274581505683*^49, 0.0770387920958333},
{0.00630957344480193, -14.619694946405195, \
-1.3569781967203665*^49, 0.07725366322862573},
{0.007943282347242814, -14.115080888527912, \
-6.801001485068046*^48, 0.07739012922334851},
{0.01, -13.607560271569687, -3.3982958779998454*^48,
0.0771018106537571},
{0.012589254117941675, -13.095519359896302, \
-1.710097520600141*^48, 0.07686997193087373},
{0.01584893192461114, -12.57502890910125, \
-8.690391178966214*^47, 0.0759855227210446},
{0.0199526231496888, -12.044506899327656, \
-4.371902972077502*^47, 0.07282260228552294},
{0.025118864315095808, -11.508867694748597, \
-2.1773382055232237*^47, 0.06994033587672496},
{0.03162277660168379, -10.974125604097805, \
-1.0633537829077974*^47, 0.06503886133118436},
{0.039810717055349734, -10.444363631208544, \
-5.112432708696619*^46, 0.06099002866348603},
{0.05011872336272725, -9.921345251439233, \
-2.501552056154496*^46, 0.05697924054878366},
{0.06309573444801933, -9.405008535108163, \
-1.1914528506215466*^46, 0.05377917472899783},
{0.07943282347242818, -8.893455863058334, \
-5.952272541408941*^45, 0.05042561999840631},
{0.1, -8.385806548209892, -2.914976254908381*^45,
0.04632976895359},
{0.12589254117941676, -7.880454160420435, \
-1.4737468497974092*^45, 0.039566117973202475},
{0.15848931924611143, -7.138243474959937, \
-7.174174780329404*^44, 0.02942287460063761},
{0.19952623149688808, -6.626336945176083, \
-2.965865068779192*^44, 0.017144010394089228},
{0.25118864315095824, -6.108043591225297, \
-9.038610774285183*^43, 0.01313376026977404},
{0.31622776601683794, -5.582083828910504, \
-4.548117030242562*^43, 0.012708083189742772},
{0.3981071705534973, -5.052661452093368, \
-2.1806808000591704*^43, 0.012360362229429312},
{0.5011872336272725, -4.524046758491513, -1.112629480001722*^43,
0.01199402899466483},
{0.6309573444801936, -3.9994743706180884, \
-5.3097114984886734*^42, 0.011537300885158159},
{0.7943282347242822, -3.479883065855086, \
-2.6955468924524333*^42, 0.011267163642615846},
{1., -2.965140354827245, -1.3165469387026805*^42,
0.010824589365892487},
{1.2589254117941675, -2.453828397690545, \
-6.4755820044992164*^41, 0.010584319966540402},
{1.584893192461114, -1.9454167886779412, \
-3.2633903693694033*^41, 0.010215016118577763},
{1.9952623149688808, -1.4391265773321897, \
-1.5547777621410808*^41, 0.010048630681173598},
{2.5118864315095824, -0.9347011259099397, \
-7.989912520361756*^40, 0.009903098920860035},
{3.1622776601683795, -0.4315440818394637, \
-3.8252581674440914*^40, 0.00972893547965847},
{3.981071705534973,
0.07068148630045823, -1.9247852842573809*^40,
0.00971813690860107},
{5.011872336272725, 0.5721584641714871, -9.626194155274167*^39,
0.009606387739884165},
{6.309573444801936, 1.0730475993443855, -4.7648846361866947*^39,
0.00964133432146204},
{7.943282347242821, 1.573490988036743, -2.3835091416492644*^39,
0.009554013386705201},
{10., 2.073808527167923, -1.1949012290701652*^39,
0.009564214098470244},
{12.589254117941687, 2.5742249035666016, -6.027369878562932*^38,
0.00955817523693132},
{15.848931924611142,
3.0743680427736577, -3.0297492350947725*^38,
0.009445529481161313},
{19.952623149688787, 3.574441950151861, -1.4987904366966382*^38,
0.009380697335597966},
{25.11886431509582, 4.0744637940244415, -7.670096311434633*^37,
0.009133085878059534},
{31.622776601683793, 4.574453067816407, -3.7568252576901522*^37,
0.009002785628402312},
{39.81071705534978, 5.074525988991956, -1.898737042484131*^37,
0.008740308444495148},
{50.11872336272725, 5.574464995295442, -9.045965296413293*^36,
0.008487702523222045},
{63.09573444801943, 6.0745291537589585, -4.569314012352323*^36,
0.008328828070587496},
{79.43282347242821, 6.574382102294367, -2.2428280122531448*^36,
0.008070902363797764}};
mass = 0.001;
Tini = 79;
Tfinal = 0.001;
rate[T_] =
10^Interpolation[datatable[[All, {1, 2}]], InterpolationOrder -> 1][
T];
jacobian[T_] =
Interpolation[datatable[[All, {1, 3}]], InterpolationOrder -> 1][T];
feq[T_] =
Interpolation[datatable[[All, {1, 4}]], InterpolationOrder -> 1][T];
fDynamics[f_, T_] = -rate[T]/(1 + 2.2*10^-7 T^6/mass^2)^2 (f - feq[T]);
When trying to solve the equation:
fsol = f /.
NDSolve[{D[f[T], T] jacobian[T]^-1 == fDynamics[f[T], T],
f[Tini] == 0}, f, {T, Tfinal, Tini}][[1]]
I get the error
NDSolve::ndsz: At T == 79., step size is effectively zero; singularity or stiff system suspected.
Could you please tell me how to avoid it?

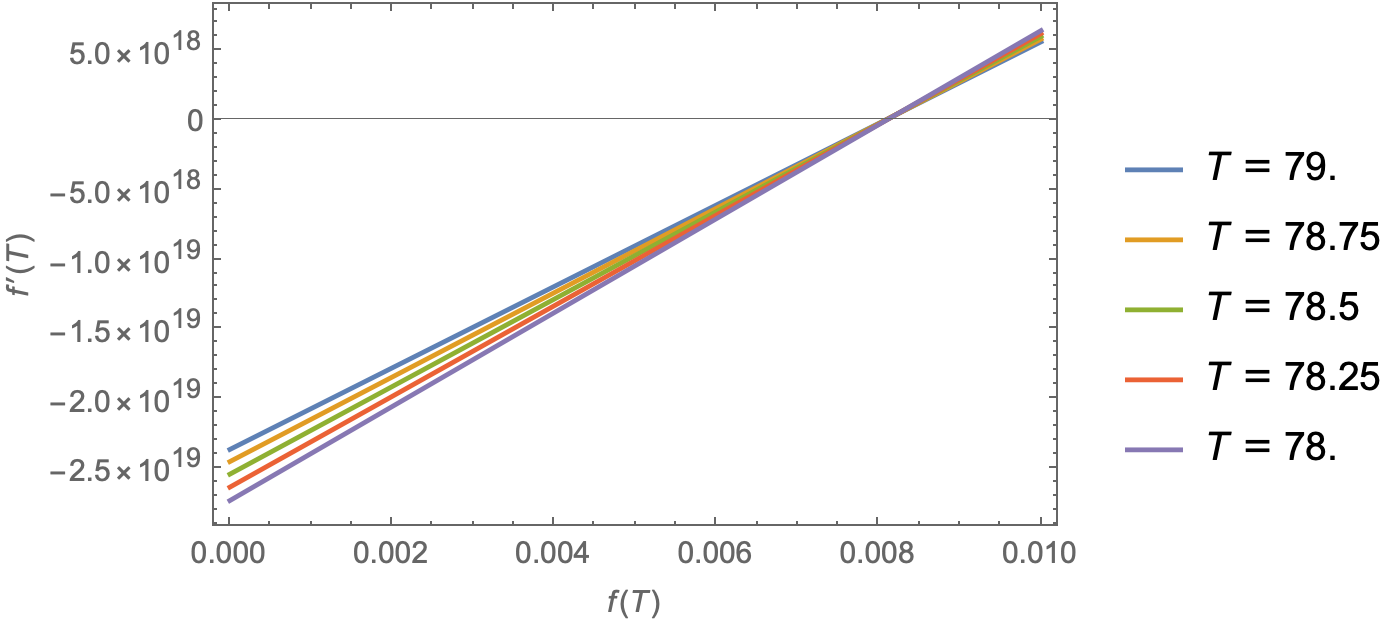
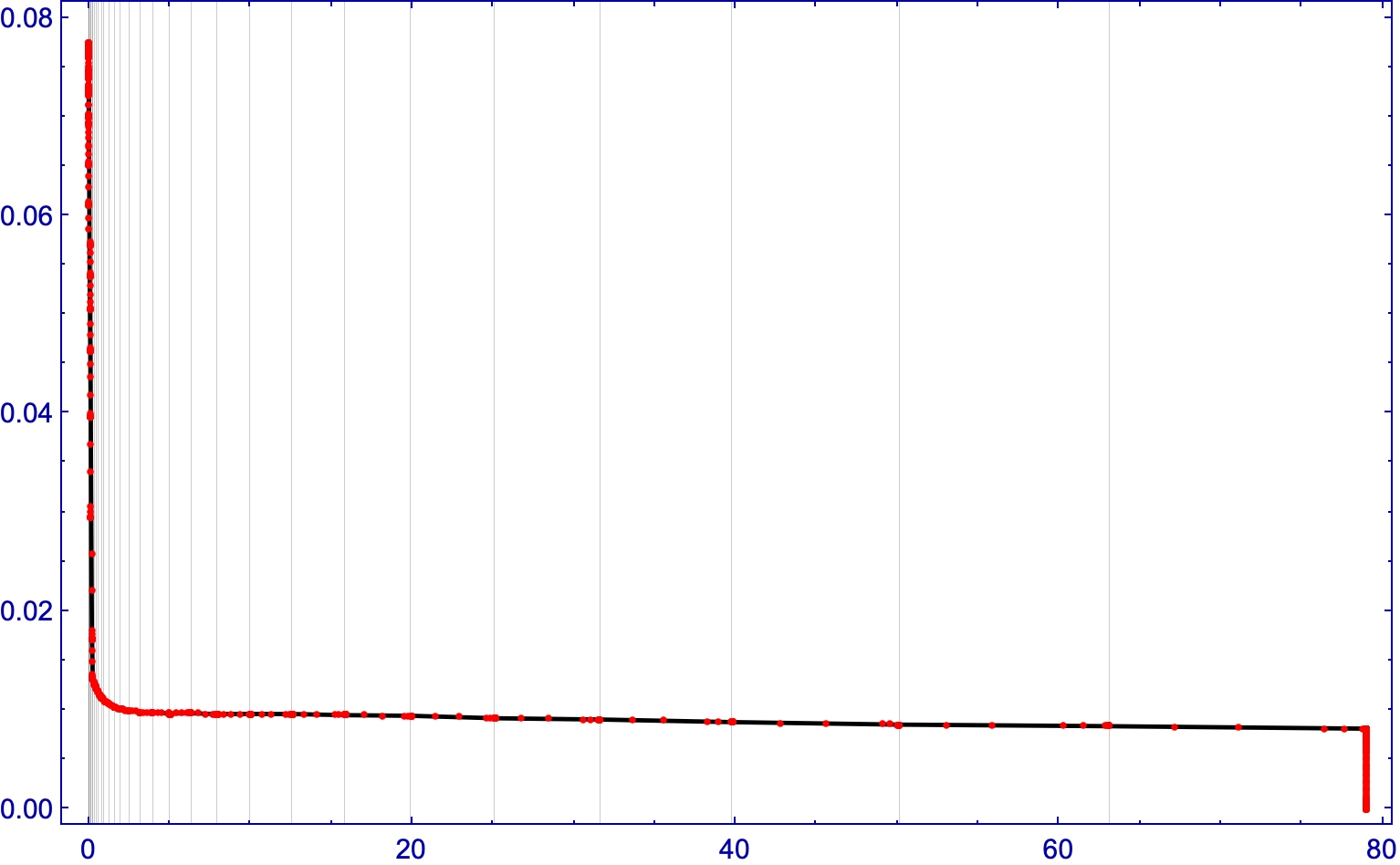
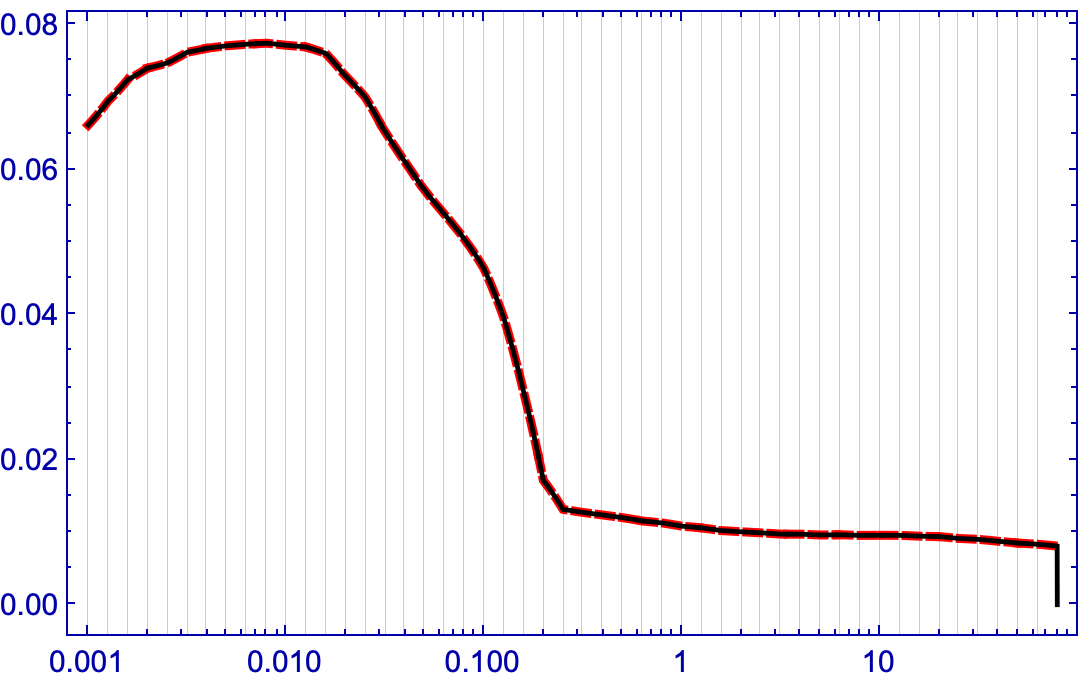

Method-> "StiffnessSwitching"andNDSolveevaluates without error message. $\endgroup$g[t]? $\endgroup$