Ticks and Frame don't play well together.
This get's you part of the way (Note edited 1 Dec 11:20 PM EST) ...
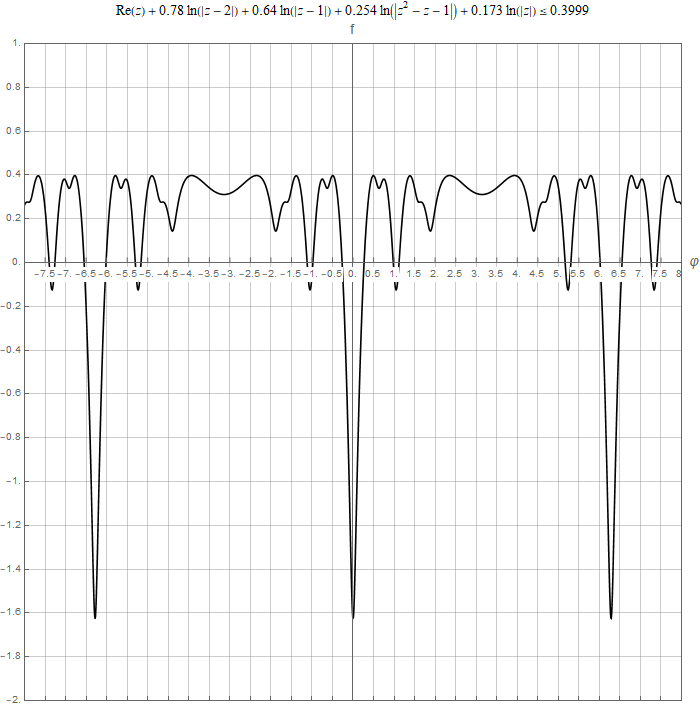
Plot[
With[{z = 1.1*E^(I u) + 0.908*E^(-I u)},
Re[z] + 0.78*Log[Abs[z - 2]] + 0.64*Log[Abs[z - 1]] +
0.254*Log[Abs[z^2 - z - 1]] + 0.173*Log[Abs[z]]], {u, -15, 15},
PlotStyle -> {Black},
PlotRange -> {{-8, 8}, {-2, 1}},
AspectRatio -> 1,
AxesLabel -> (Style[#, 14] & /@ {"\[CurlyPhi]", "f"}),
GridLines -> {Range[-8, 8, .5], Range[-2, 1, .2]},
AxesOrigin -> {-8, 0},
Ticks -> {Range[-8, 8, .5], Range[-2, 1, .2]},
(* Frame->True, *)
(* FrameTicks->{{Range[-2,1,.2],Automatic},None}, *)
PlotLabel ->
Style[TraditionalForm[
HoldForm[
Re[z] + 0.78*ln[Abs[z - 2]] + 0.64*ln[Abs[z - 1]] +
0.254*ln[Abs[z^2 - z - 1]] + 0.173*ln[Abs[z]] <= 0.3999]],
"TR", Black, 14],
ImageSize -> Large]
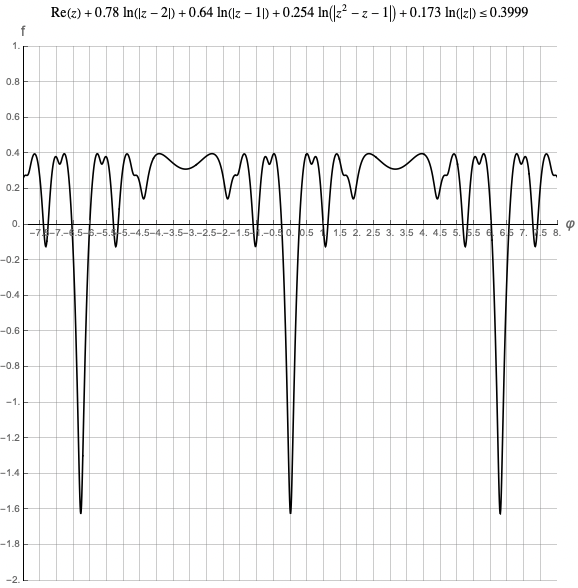
The solution does not have a Frame as your original solution did, perhaps someone can suggest how to add one (See new edit below).
I'll give it some more thought.
After some more thought a bit of a funky solution messing around with GridLines to give the Plot a pseudo Frame. I welcome any more elegant suggestions ;-) ...
Plot[
With[{z = 1.1*E^(I u) + 0.908*E^(-I u)},
Re[z] + 0.78*Log[Abs[z - 2]] + 0.64*Log[Abs[z - 1]] +
0.254*Log[Abs[z^2 - z - 1]] + 0.173*Log[Abs[z]]], {u, -15, 15},
PlotStyle -> {Black},
PlotRange -> {{-8, 8}, {-2, 1}},
AspectRatio -> 1,
AxesLabel -> (Style[#, 14] & /@ {"\[CurlyPhi]", "f"}),
GridLines -> {AppendTo[
Range[-8, 7.5, .5], {8, {Thickness[0.0025], Black}}],
AppendTo[
AppendTo[
Range[-2,
0.8, .2], {1, {Thickness[0.0005], Black}}], {-2, {Thickness[
0.0005], Black}}]},
AxesOrigin -> {-8, 0},
Ticks -> {Range[-8, 8, .5], Range[-2, 1, .2]},
PlotLabel ->
Style[TraditionalForm[
HoldForm[
Re[z] + 0.78*ln[Abs[z - 2]] + 0.64*ln[Abs[z - 1]] +
0.254*ln[Abs[z^2 - z - 1]] + 0.173*ln[Abs[z]] <= 0.3999]],
"TR", Black, 14],
ImageSize -> Large]
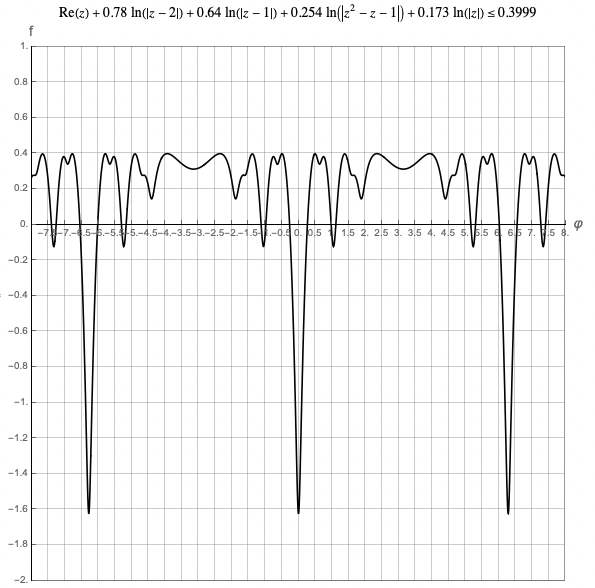

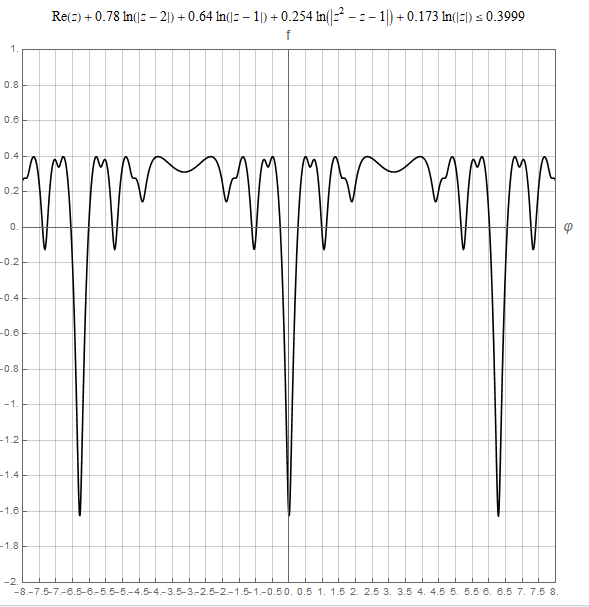
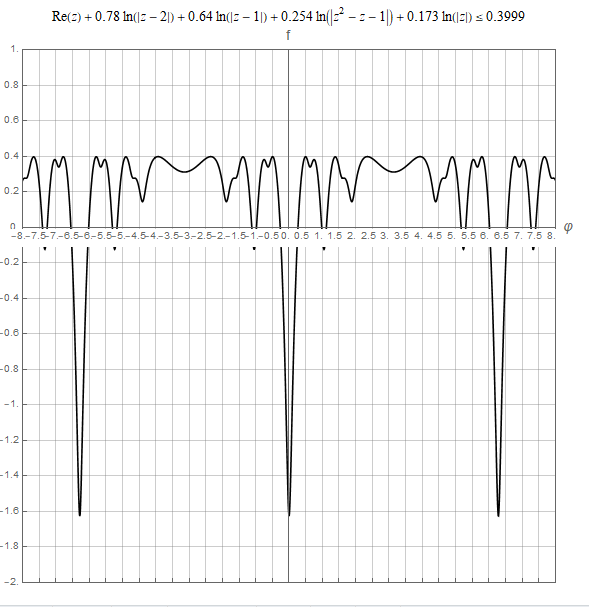


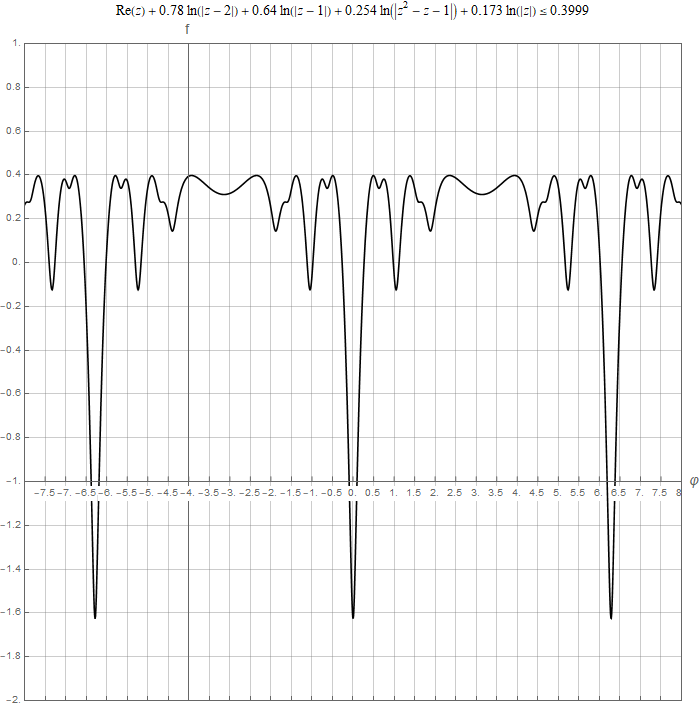
AxesOrigin -> {0, 0}inside yourPlotexpression. $\endgroup$