Using NDSolve on wave PDE on string, when solution given at 2 different times instead of initial velocity?

Question

This is a PDE taken from a Maple document. Mathematica DSolve currently unable to solve it.

I wanted to verify Maple solution using NDSolve. This is string of length 1, fixed on the left, and free to move on the right. Given an initial position and let go.

Here is the specs of the PDE

Solve for $0<x<1, t>0$ the wave PDE $$ -u_{tt} + u(x,t)= u_{xx} + 2 e^{-t} \left( x - \frac{1}{2} x^2 + \frac{1}{2} t - 1 \right) $$

With boundary condition

\begin{align*} u(0,t) &= 0 \\ \frac{\partial u(1,t)}{\partial x} &= 0 \end{align*}

And initial conditions

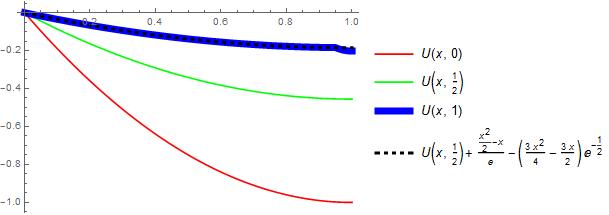
\begin{align*} u(x,0) &= x^2-2 x \\ u(x,1)&= u\left(x,\frac{1}{2}\right) + e^{-1} \left( \frac{1}{2} x^2-x\right) - \left( \frac{3}{4} x^2- \frac{3}{2}x \right) e^{\frac{-1}{2}} \end{align*}

The tricky part in this, is that no initial velocity is given. But only initial position at $t=0$, and then a relation on the solution at 2 different times is give instead.

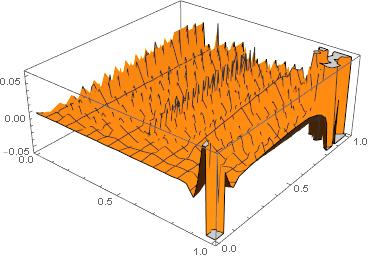
NDSolve complain with that dreaded error

Boundary condition is not specified on a single edge of the boundary of the computational domain.

And I do not know how to get rid of it. Here is the code

ClearAll[u, x, t];
pde = -D[u[x, t], {t, 2}] + u[x, t] == 
           D[u[x, t], {x, 2}] + 2*Exp[-t]*(x - (1/2)*x^2 + (1/2)*t - 1);

bc = {u[0, t] == 0, Derivative[1, 0][u][1, t] == 0};

ic = {u[x, 0] == x^2 - 2*x, 
    u[x, 1] == u[x, 1/2] + ((1/2)*x^2 - x)*Exp[-1] - ((3*x^2)/4 - (3/2)*x)* Exp[-2^(-1)]};

sol = NDSolve[{pde, ic, bc}, u, {x, 0, 1}, {t, 0, 1}]

Here is the Maple code and the analytical solution it gives

pde := -diff(u(x, t), t, t) + u(x, t) = 
       diff(u(x, t), x, x)+ 2*exp(-t)*(x-(1/2)*x^2+(1/2)*t-1);
ic  := u(x, 0) = x^2-2*x, 
       u(x, 1) = u(x, 1/2)+((1/2)*x^2-x)*exp(-1)-(3/4*(x^2)-3/2*x)*exp(-1/2);
bc  := u(0, t) = 0, eval(diff(u(x, t), x), {x = 1}) = 0;
pdsolve([pde, ic, bc],u(x,t))

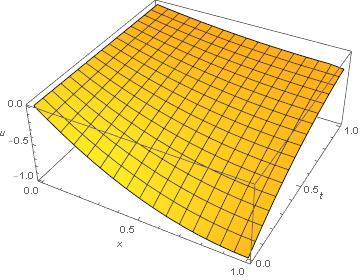
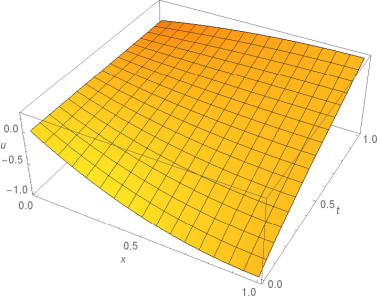
$$ u(x,t) = -\frac{e^{-t}}{2} (x^2-2 x) (t-2) $$

Here is animation of Maple solution, which I wanted to verify

mapleSol[x_, t_] := -(Exp[-t]/2) (x^2 - 2 x) (t - 2)
Manipulate[
 Plot[mapleSol[x, t], {x, 0, 1}, PlotRange -> {{0, 1}, {-1, .1}}],
 {{t, 0, "time"}, 0, 10, .1}
 ]

Any suggestion how to get rid of the error from NDSolve?

Using V 12 on windows 10. ps. I solved this by hand also, but can't get Maple solution, and my solution looks wrong. I still need to find out why.

Answer 1

I don't have time to make a solid answer, but this seems to work :

pde = -D[u[x, t], {t, 2}] + u[x, t] == 
   D[u[x, t], {x, 2}] + 2*Exp[-t]*(x - (1/2)*x^2 + (1/2)*t - 1) + 
    NeumannValue[0, x == 1];

bc = {u[0, t] == 0};

ic = {u[x, 0] == x^2 - 2*x
   , PeriodicBoundaryCondition[
    u[x, t] - (((1/2)*x^2 - x)*Exp[-1] - ((3*x^2)/4 - (3/2)*x)*
        Exp[-2^(-1)])
    , t == 1 && 0 < x < 1
    , Function[xy, xy - {0, 1/2}]]};

U = NDSolveValue[{pde, ic, bc}, u, {x, 0, 1}, {t, 0, 1}];
Plot3D[U[x, t], {x, 0, 1}, {t, 0, 1}, AxesLabel -> {x, t, u}]  

Plot[{
  U[x, 0]
  , U[x, 1/2]
  , U[x, 1]
  , U[x, 1/2] + ((1/2)*x^2 - x)*Exp[-1] - ((3*x^2)/4 - (3/2)*x)*
    Exp[-2^(-1)]}, {x, 0, 1}, 
 PlotStyle -> {Red, Green, Directive[Blue, AbsoluteThickness[7]], 
   Directive[Black, Dashed, AbsoluteThickness[3]]}, 
 PlotLegends -> "Expressions"]  

Here is the error :

Plot3D[Evaluate[-D[U[x, t], {t, 2}] + 
   U[x, t] - (D[U[x, t], {x, 2}] + 
     2*Exp[-t]*(x - (1/2)*x^2 + (1/2)*t - 1))], {t, 0, 1}, {x, 0, 1}]  

The method automaticaly chosen by NDSolve is Method -> {"PDEDiscretization" -> {"FiniteElement"}} (as opposed to Method] -> {"PDEDiscretization" -> {"MethodOfLines", "SpatialDiscretization" -> {"FiniteElement", femopts}}}) . This is the reason why one can impose boundaries condition on the variable "time".

Note also that the term "PeriodicBoundaryCondition" is a little bit misleading because the source of the "boundary condition" does not need to be a boundary.

Answer 2

Just an extended comment:

If you change the second bc to NeumannValue Mathematica is able to solve the modified initial value problem u[x, 1] ==(* u[x,1/2]+*) ((1/2)*x^2 - x)*Exp[-1] - ((3*x^2)/4 - (3/2)*x)*Exp[-2^(-1)]

pde = -D[u[x, t], {t, 2}] + u[x, t] ==D[u[x, t], {x, 2}] + 2*Exp[-t]*(x - (1/2)*x^2 +(1/2)*t - 1) +NeumannValue[0, x == 1];

bc = {u[0, t] == 0};

ic = {u[x, 0] == x^2 - 2*x, 
u[x, 1] ==(* u[x,1/2]+*) ((1/2)*x^2 - x)*Exp[-1] - ((3*x^2)/4 - (3/2)*x)*Exp[-2^(-1)]};

U = NDSolveValue[{pde, ic, bc}, u, {x, 0, 1}, {t, 0, 1} ];
Plot3D[U[x, t], {x, 0, 1}, {t, 0, 1}, AxesLabel -> {x, t, u}]

The coupling u[x,1],u[x,1/2] still remains unsolved!