Making a List For Use in an Integral

Question

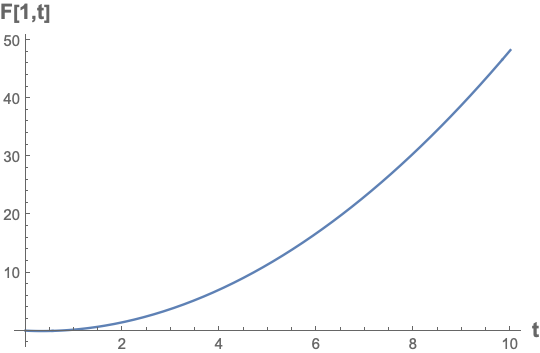
I have an equation of the form

$R_{min}+t= R_{min}\, e^{R_{min}}$

where for every time $t$ there is a corresponding position $R_{min}$.

How can I complete the integral

$F(t)=\int_{R_{min}(t)}^{r(t)}R\,dR$ $\, \, \, \, \, \, \, \, \,$where $r(t)=Kt$ and $K$ is a constant.

and create a plot of time $t$ vs. $F(t)$?

Answer 1

Clear[Rmin, F, K]

Rmin[t_?NumericQ] := r /. NSolve[{r + t == r*E^r, 0 < r < 10}, r][[1]]

F[K_, t_] = Integrate[R, {R, Rmin[t], K*t}] // Simplify

(* 1/2 (K^2 t^2 - Rmin[t]^2) *)

Plot[F[1, t], {t, 0, 10},
 AxesLabel -> (Style[#, 14, Bold] & /@ {"t", "F[1,t]"})]