Overlaying StreamPlot onto a Plot3D as its Mesh Lines

Question

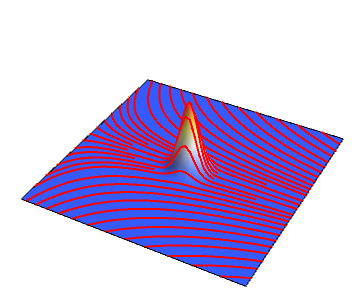
I am trying to replace the grid lines of a Plot3D with the output of a StreamPlot using MeshFunction. Here is an example:

mu = {{0, 0, 0}};
c = 0.1;
sigma = {{c, 0, 0}, {0, c, 0}, {0, 0, c}};
sigmaInv = Inverse[sigma];
dsigma = Det[sigma];
gauss3d[x_, y_, z_] := Exp[-(1/2)
     (({{x, y, z}} - mu).sigmaInv.Transpose[{{x, y, z}} - mu])[[1, 1]]
    ] / Sqrt[(2 Pi)^3 dsigma];
bl = -4;
bh = 4;
Plot3D[gauss3d[x, y, 0.] , {x, bl, bh}, {y, bl, bh}, 
 PlotRange -> {-0.01, 2.5}, ColorFunction -> "TemperatureMap", 
 Axes -> None, Boxed -> {},
 MeshFunctions -> { Sqrt[#1^2 + #2^2 + #3^2] & },
 Mesh -> 10, LabelStyle -> {White}]
kb = 0.9;
kn = 0.2;
kt = 0;
theta[x_, y_, z_] := ArcTan[ (kt*x - kn*y)/(1 + kn*x - kt*y) ] + kb*z;
vvvZero = 
 StreamPlot[{-Cos[theta[x, y, 0]], -Sin[theta[x, y, 0]]}, {x, bl, 
   bh}, {y, bl, bh},
  StreamStyle -> {"Line", Red, Thick}, Frame -> False]

I would essentially like the lines of the StreamPlot to become the mesh lines of Plot3D, instead of the Sqrt[#1^2 + #2^2 + #3^2] &.

Any other suggestions are welcome; thanks!

Answer 1

Not too hard, if you're willing to manipulate primitives directly. Using the same variables as in the OP:

Show[Plot3D[gauss3d[x, y, 0.], {x, bl, bh}, {y, bl, bh}, PlotRange -> {-0.01, 2.5},
            ColorFunction -> "TemperatureMap", Axes -> None,
            Boxed -> False, Mesh -> None], 
     Graphics3D[Cases[vvvZero, GraphicsComplex[pts_, rest__] :> 
                      GraphicsComplex[Append[#, gauss3d[Sequence @@ #, 0.]] & /@ pts, 
                                      rest], ∞]]]