You more or less said that you weren't interested in a "hand-made" image processing solution, but since TextRecognize is notoriously unreliable, I thought I'd try it anyway. Classify does most of the heavy lifting, so the solution isn't simple, but it's not hard to understand, either.
Ok, first step: import the image, adjust for the inhomogeneous lightning and find the individual characters:
img = Import["https://i.sstatic.net/BQOAQ.jpg"]
white = Closing[img, 10];
whiteAdjusted = ImageApply[Divide, {img, white}];
components =
ComponentMeasurements[
MorphologicalBinarize[ColorNegate@whiteAdjusted], {"BoundingBox",
"Area"}, #2 > 10 &][[All, 2, 1]];
then sort the components by their left X-coordinate, i.e. from left to right (this makes it easier to just join the characters in each table cell later on).
components = SortBy[components, #[[1, 1]] &];
Next step: Train a Classifier to recognize digits. Since we know the font, this is much easier than the task TextRecognize has to do:
characters = Flatten[Table[
ImageCrop[
Rasterize[Text[Style[#, FontFamily -> "Times New Roman"]],
ImageSize -> size]] -> # & /@
Characters["0123456789t"], {size, {10, 15, 20, 30, 40, 50}}]];
simpleOcr = Classify[characters];
Let's try it:
ImageTrim[img, #] & /@ components // GroupBy[simpleOcr] // KeySort

That looks very promising.
Step 3: Tell mathematica where the table grid is. It's probably possible to recognize this automatically, but if you only have this one table, that would be overkill. So I just used LocatorPane to move the grid to the right spot:
gridPts = {{85, 678}, {837, 687}, {65, 42}, {862, 48}};
LocatorPane[Dynamic[gridPts], Dynamic[Show[img,
With[{transform =
Last@FindGeometricTransform[
gridPts, {{0, 0}, {1, 0}, {0, 1}, {1, 1}}*10 + 1.5,
TransformationClass -> "Perspective"]},
Graphics[
{Red,
Table[Line[transform /@ {{i, 1}, {i, 12}}], {i, 12}],
Table[Line[transform /@ {{1, i}, {12, i}}], {i, 12}]}]]]]]

A few definitions for readability:
This transforms image-coordinates into {row,column}-grid coordinates:
imageToGrid =
Last@FindGeometricTransform[{{0, 0}, {0, 1}, {1, 0}, {1, 1}}*10 +
1.5, gridPts, TransformationClass -> "Perspective"];
This reads a single digit given the bounding box:
readDigit =
Function[boundingBox, simpleOcr[ImageTrim[img, boundingBox]]];
This returns the (integer) row/column coordinates of a bounding box:
gridCell =
Function[boundingBox, Floor[imageToGrid[Mean[boundingBox]]]];
And this reads the full grid:
readGrid =
ToExpression[StringJoin[#]] & /@
GroupBy[components, gridCell -> readDigit]
<|{11, 1} -> t10, {10, 1} -> t9, {9, 1} -> t8, {8, 1} -> t7, {7, 1} ->
t6, {6, 1} -> t5, {5, 1} -> t4, {4, 1} -> t3, {3, 1} ->
t2, {2, 1} -> t1, {11, 2} -> 5, {9, 2} -> 4, {10, 2} ->
1, {8, 2} -> 2, {7, 2} -> 6, {6, 2} -> 4, {5, 2} -> 7, {4, 2} ->
6, {3, 2} -> 2, {1, 2} -> t1, {2, 2} -> 6, {11, 3} ->
10, {10, 3} -> 2, {9, 3} -> 7, {8, 3} -> 6, {7, 3} -> 3, {6, 3} ->
2, {5, 3} -> 2, {1, 3} -> t2, {4, 3} -> 2, {3, 3} -> 3, {2, 3} ->
3, {10, 4} -> 3, {11, 4} -> 1, {4, 4} -> 10, {9, 4} -> 8, {7, 4} ->
9, {8, 4} -> 1, {6, 4} -> 8, {1, 4} -> t3, {5, 4} -> 6, {3, 4} ->
8, {2, 4} -> 7, {11, 5} -> 6, {10, 5} -> 4, {9, 5} -> 2, {1, 5} ->
t4, {8, 5} -> 7, {7, 5} -> 7, {6, 5} -> 9, {5, 5} -> 8, {4, 5} ->
3, {3, 5} -> 6, {2, 5} -> 2, {1, 6} -> t5, {11, 6} -> 2, {10, 6} ->
6, {9, 6} -> 5, {8, 6} -> 5, {7, 6} -> 8, {4, 6} -> 9, {3, 6} ->
8, {2, 6} -> 8, {6, 6} -> 1, {5, 6} -> 1, {8, 7} -> 10, {6, 7} ->
10, {2, 7} -> 10, {1, 7} -> t6, {7, 7} -> 2, {5, 7} -> 9, {3, 7} ->
9, {10, 7} -> 9, {9, 7} -> 6, {4, 7} -> 5, {11, 7} -> 7, {9, 8} ->
10, {1, 8} -> t7, {3, 8} -> 5, {5, 8} -> 3, {8, 8} -> 4, {6, 8} ->
3, {4, 8} -> 1, {2, 8} -> 1, {10, 8} -> 5, {7, 8} -> 1, {11, 8} ->
3, {3, 9} -> 10, {1, 9} -> t8, {2, 9} -> 9, {5, 9} -> 4, {4, 9} ->
7, {7, 9} -> 4, {6, 9} -> 5, {8, 9} -> 9, {9, 9} -> 9, {10, 9} ->
8, {11, 9} -> 8, {5, 10} -> 10, {1, 10} -> t9, {2, 10} ->
5, {3, 10} -> 7, {10, 10} -> 10, {4, 10} -> 8, {6, 10} ->
6, {7, 10} -> 5, {8, 10} -> 3, {9, 10} -> 3, {11, 10} ->
4, {1, 11} -> t10, {2, 11} -> 4, {7, 11} -> 10, {4, 11} ->
4, {3, 11} -> 1, {6, 11} -> 7, {5, 11} -> 5, {8, 11} ->
8, {10, 11} -> 7, {9, 11} -> 1, {11, 11} -> 9|>
Or, in matrix form:
MatrixForm[SparseArray[Normal[readGrid], Automatic, ""]]
Returns:
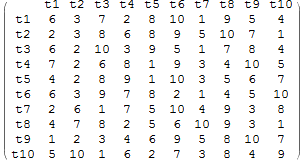
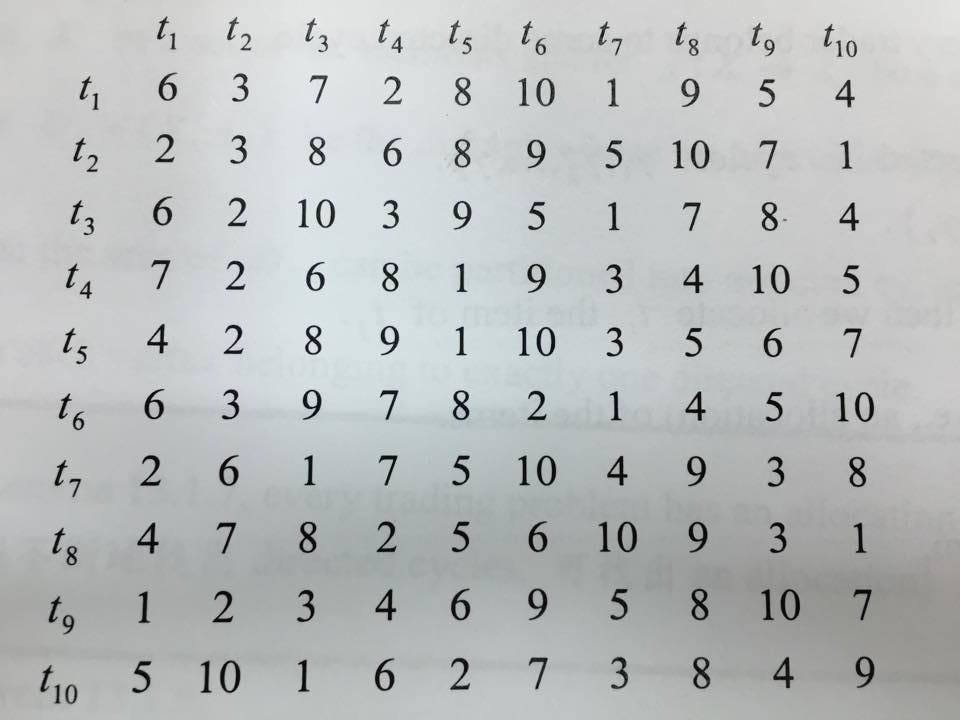





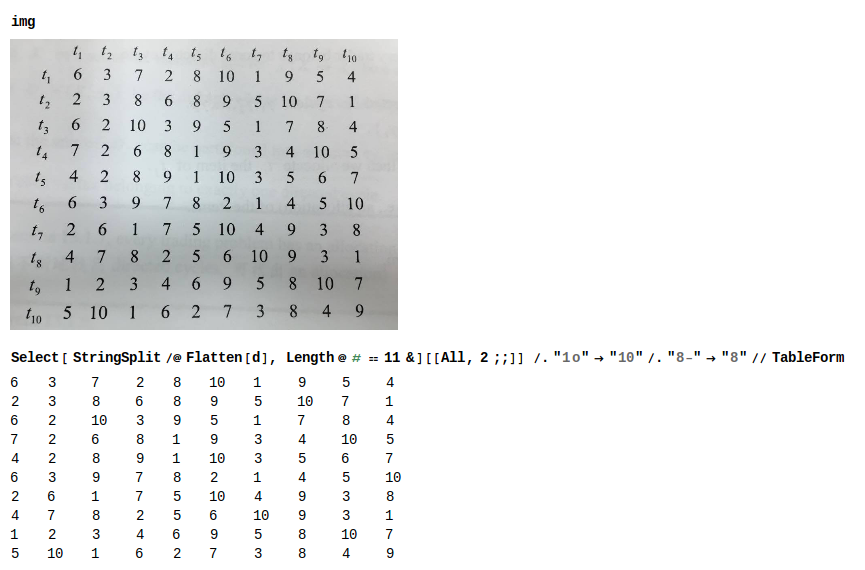
TextRecognize: (4464), (18683), (27045). $\endgroup$