How can I solve the equation with integers as a solution?

Question

I want to solve the equation $$(x-1)^2 + (y-1)^2 + (z-1)^2 = 49$$ where $x$, $y$, $z$ are integer and $x \neq 1$, $y \neq 1$, $x \neq 1$. How do I tell Mathematica to do that?

Answer 1

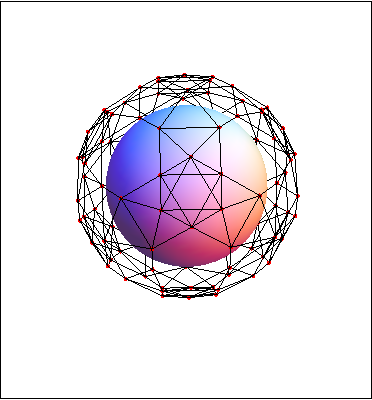
A geometrical view of the solutions:

s = Solve[(x - 1)^2 + (y - 1)^2 + (z - 1)^2 == 49, {x, y, z},  Integers];
pts = {x, y, z} /. s;
subs = Subsets[pts, {2}];
minds = Union[dists = N[EuclideanDistance @@@ subs]][[1 ;; 3]];
Show[Graphics3D[Sphere[{1, 1, 1}, 13/2]], 
     Graphics3D[Line /@ Extract[subs, Position[dists, Alternatives @@ minds]]],
     ListPointPlot3D[pts, PlotStyle -> Directive[PointSize[Medium], Red]],
     Boxed -> False]

Edit

Another useful visualization:

s = Solve[(x - 1)^2 + (y - 1)^2 + (z - 1)^2 == 49 && 
             x != 1 && y != 1 && z != 1, {x, y, z}, Integers];
pts = {x, y, z} /. s;

w = Sqrt@13;
f[i_, k_] := RotateLeft[{w Cos@u, w Sin@u, k} + {1, 1, 0}, i];

Show[Graphics3D[Sphere[{1, 1, 1}, 13/2]],
     ParametricPlot3D[Flatten[{f[1, #], f[2, #], f[3, #]} & /@ {-5, 7}, 1], {u, 0, 2 Pi}],
     ListPointPlot3D[pts, PlotStyle -> Directive[PointSize[Large], Red]],
     Boxed -> False]

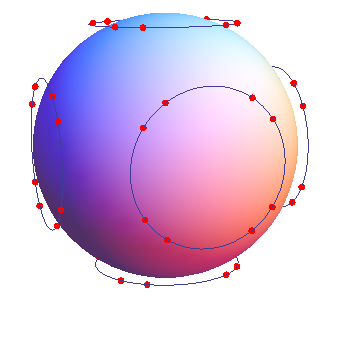
Edit

Note that the problem turns religious if you use 81 instead of 49:

Answer 2

Try :

Solve[(x - 1)^2 + (y - 1)^2 + (z - 1)^2 == 49, {x, y, z}, Integers]

or

Reduce[(x - 1)^2 + (y - 1)^2 + (z - 1)^2 == 49, {x, y, z}, Integers]

You can add inequalities as well as :

Solve[{(x - 1)^2 + (y - 1)^2 + (z - 1)^2 == 49, x != 1, y != 1, z != 1}, {x, y, z}, Integers]

Answer 3

Or use my favorite command:

FindInstance[
{(x - 1)^2 + (y - 1)^2 + (z - 1)^2 == 49, x != 1, y != 1,z != 1}, {x, y, z},Integers, 100]