We can adapt the MonitorMethod:
Options[MonitorMethod] = {Method -> Automatic,
"MonitorFunction" ->
Function[{h, state, meth},
Print[{"H" -> h, "SD" -> state@"SolutionData"}]]};
MonitorMethod /:
NDSolve`InitializeMethod[MonitorMethod, stepmode_, sd_, rhs_,
state_, OptionsPattern[MonitorMethod]] := Module[{submethod, mf},
mf = OptionValue["MonitorFunction"];
submethod = OptionValue[Method];
submethod =
NDSolve`InitializeSubmethod[MonitorMethod, submethod, stepmode,
sd, rhs, state];
MonitorMethod[submethod, mf]];
MonitorMethod[submethod_, mf_]["Step"[f_, h_, sd_, state_]] :=
Module[{res},
res = NDSolve`InvokeMethod[submethod, f, h, sd, state];
If[Head[res] === NDSolve`InvokeMethod,
Return[$Failed]]; (* submethod not valid for monitoring *)
mf[h, state, submethod];
If[SameQ[res[[-1]], submethod], res[[-1]] = Null,
res[[-1]] = MonitorMethod[res[[-1]], mf]];
res];
MonitorMethod[___]["StepInput"] = {"Function"[All], "H",
"SolutionData", "StateData"};
MonitorMethod[___]["StepOutput"] = {"H", "SD", "MethodObject"};
MonitorMethod[submethod_, ___][prop_] := submethod[prop];
If the Method implements the "StepError" method, it will return the (scaled) step error estimate. (The only way to know the true error is to know the true solution and compare.) By "scaled," Mathematica means
$$\text{scaled error}
= {|\text{error}| \over 10^{-\text{ag}} + 10^{-\text{pg}} | x |} \,,$$
which will be between 0 and 1 when the $\text{error}$ satisfies the AccuracyGoal $\text{ag}$ and the PrecisionGoal $\text{pg}$.
The MonitorMethod takes a "MonitorFunction" option, which should be a function of the form
Function[{h, state, meth}, <...body...>]
where h is the step size, state is the NDSolve`StateData object, and meth is the Method object of the submethod.
Example use:
{sol, {errdata}} = Reap[
NDSolveValue[{x''[t] + x[t] == 0, x[0] == 1, x'[0] == 1},
x, {t, 0, 2},
Method -> {MonitorMethod,
"MonitorFunction" ->
Function[{h, state, meth},
Sow[meth@"StepError", "ScaledStepError"]]},
MaxStepFraction -> 1, WorkingPrecision -> 100,
PrecisionGoal -> 23, AccuracyGoal -> 50],
"ScaledStepError"];
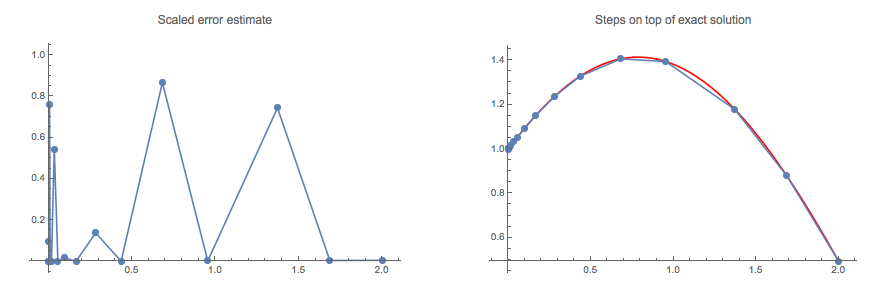
GraphicsRow[{
ListLinePlot[Transpose@{Flatten@Rest@sol@"Grid", errdata},
Mesh -> All, PlotRange -> {0, 1}, PlotRangePadding -> Scaled[.05],
PlotLabel -> "Scaled error estimate"],
Show[
Plot[Sin[t] + Cos[t], {t, 0, 2}, PlotStyle -> Red],
ListLinePlot[sol, Mesh -> All],
PlotRangePadding -> Scaled[.05],
PlotLabel -> "Steps on top of exact solution"]
}]
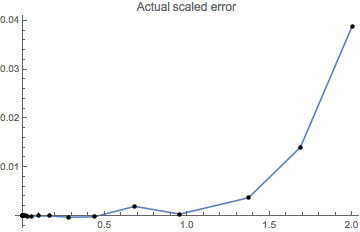
In our example, we know the exact solution, so we can check the actual error:
Block[{t = Flatten@sol@"Grid", data},
data = Transpose@{t, (Sin[t] + Cos[t] - sol[t])/(
10^-50 + 10^-23 Abs[Sin[t] + Cos[t]])};
ListLinePlot[data,
Epilog -> {PointSize@Medium, Tooltip[Point[#], N@Last@#] & /@ data},
PlotRange -> All, PlotLabel -> "Actual scaled error"]
]
Of course, when I'm this interested in the error, it's usually because I have reasons for wondering whether the error estimates, which are based on discrete approximations to a function assumed to have a certain smoothness, are unreliable.