FindFormula is great start on the path to full symbolic regression and I realize it's level of development is only experimental at this stage. But if I happen to know that the underlying function I seek (for which I only have a small sample of data points) is monotonically decreasing with the independent variable x, can I communicate this desired feature to FindFormula? The intent would be for FindFormula to then return only those formulas that are monotonically decreasing with x.
Of course, one can think of many other features/constraints that could be desired, some could be based on a defined range (interval) of x.
If this capability is not yet possible, would this be of interest to others for the developers to consider for future versions of Mathematica ?
Here is a simple example
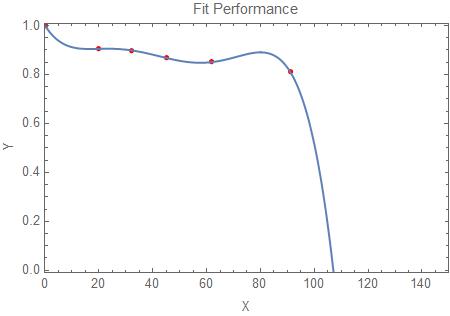
xvalue = {0, 20, 32, 45, 62, 91};
yvalue = {1, .905, .899, .868, .851, .812};
data = Transpose[{xvalue, yvalue}];
plot1 = ListPlot[data, Frame -> True, FrameLabel -> {"X", "Y"},
PlotRange -> {{0, 150}, {-.01, 1.01}}, PlotStyle -> Red]
model1 = FindFormula[data, x]
plot2 = Plot[model1, {x, 0, 150}, Frame -> True,
FrameLabel -> {"X", "Y"}, PlotRange -> {{0, 150}, {-.01, 1.01}}]
Show[plot1, plot2, PlotLabel -> "Fit Performance"]
The returned formula is
1 - 0.0172917 x + 0.00114523 x^2 - 0.0000336748 x^3 + 4.26823*10^-7 x^4 - 1.92155*10^-9 x^5
And the plot comparison
Now if I require the formula to be monotonically decreasing (everywhere) is it possible to tell FindFormula that ?
What if I require the formula to have Y values constrained between zero and 1 inclusive ?
What if I know the underlying function is asymptotic to a Y value of 0.7 ? Or asymptotic to a Y value of zero ?
Such control with singular and multiple constraints would be very useful.