Only one root at specific interval

Question

I need a help to find k here:

$f(x)=-k^x + x^2$

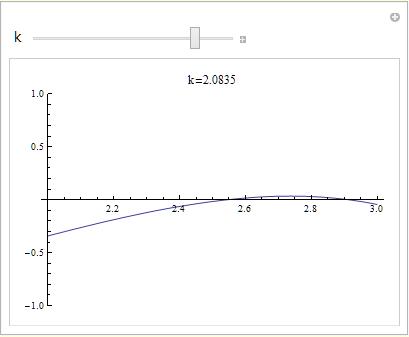
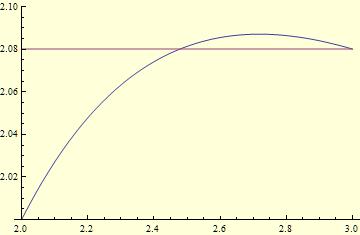
It is not too simple. I have interest to find the single value of "k" where, inside interval {x,2,3}, the function have only one value to x when f(x) = 0.

In fact, using try and error I have already found the value:

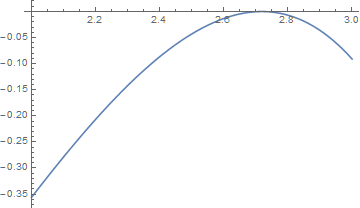
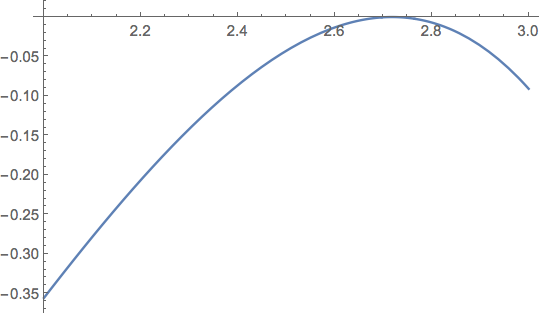
ratio = -2.0870652286345332^x + x^2

Plot[ratio, {x, 2.715, 2.720}] 

FindMaximum[ratio, {x, 2.715, 2.720}]

NSolve[ratio == 0 && x >= 2, x]

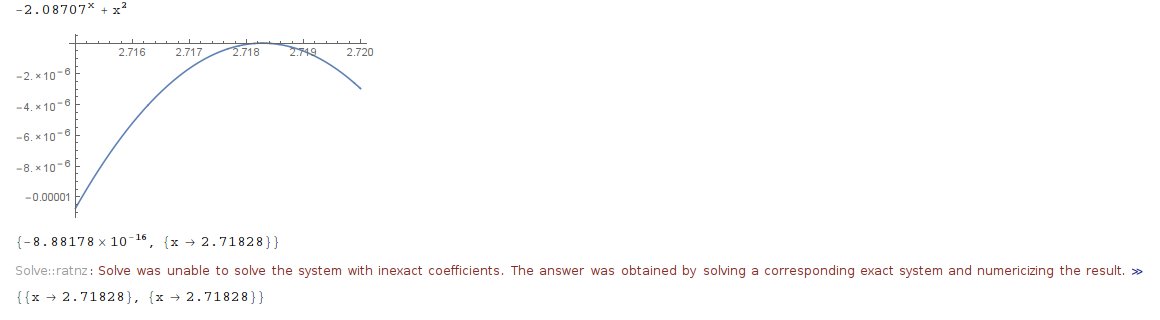
I need to know, a better way to reach the value -2.0870652286345332 to k. Optimization? Solving? I was unable to got it.

Any insight are welcome.

Best regards

Answer 1

Having just one root requires

eq1 = -k^x + x^2 == 0;
eq2 = D[-k^x + x^2, x] == 0
(* 2*x - k^x*Log[k] *)

FindRoot can solve for x and k simultaneously.

FindRoot[{eq1, eq2}, {{x, 2.5}, {k, 2}}]
(* {x -> 2.71828, k -> 2.08707} *)

Plot[(-k^x + x^2) /. %[[2]], {x, 2, 3}]

Answer 2

For an exact solution:

f[k_, x_] = -k^x + x^2;

soln = Reduce[{f[k, x] == 0, D[f[k, x], x] == 0, x > 0}, {k, x}, 
   Reals] // ToRules

{k -> E^(2/E), x -> E}

f[k, x] /. soln

0

k /. soln // N[#, 17] &

2.0870652286345330

Plot[f[k /. soln, x], {x, 2, 3}]

Answer 3

There is not only one single value of k, where f[x,k]==0 in the range [2,3] at only one x-value, but the range of from k=2 to k=3^(2/3) matches to that condition. Get an impression with

    Manipulate[Plot[f[x, k], {x, 2, 3}, PlotRange -> 1], {k, 2, 2.1}]

Solve for k

    sol = 
    First[Solve[Reduce[f[x, k] == 0, k, Reals]]] // PowerExpand

     (*   {k -> x^(2/x)}    *)

    Plot[Evaluate[{k /. sol, k /. sol /. x -> 3}], {x, 2, 3}, 
         PlotRange -> {2, 2.1}]

For k in the range k=2 to k=3^(2/3)=2.08008 there f[x,k]==0 is fulfilled at only one x-value.

     {k /. sol /. x -> 2, k /. sol /. x -> 3, k /. sol /. x -> 3.}

     (*   {2, 3^(2/3), 2.08008}    *)

Here is the corresponding x value

    {sol2 = Solve[f[x, 3^(2/3)] == 0, x], N[sol2]} // Quiet

     (*   {{{x -> 
          3}, {x -> -((3 ProductLog[-(Log[3]/3)])/Log[3])}, {x -> -((
          3 ProductLog[Log[3]/3])/Log[3])}}, {{x -> 3.}, {x -> 
          2.47805}, {x -> -0.757697}}}    *