1
$\begingroup$

Consider the following integrals that I asked Mathematica to do, 

Assuming[m >= 1 && q >= 1 && k >= 1 && m ∈ Integers && k ∈ Integers, 
  Integrate[Exp[-2 π[m q Sqrt[λ + 1/4] + k Sqrt[λ]]], {λ, 0, ∞}]]

Integrate[Tanh[π Sqrt[λ]] Log[1 - Exp[-2 π q Sqrt[λ + 1/4]]], {λ, 0, ∞}]

for various different positive values of $m,k,q$ or in general

In each of these cases, Mathematica just rewrote back the integral doing nothing. Does this mean that the integral converges, but Mathematica doesn't know how to do it?

It would be an added bonus if someone can point out if and how these integrals can be done!

Improve this question
$\endgroup$
10
  • $\begingroup$ @Nasser Why do you think so? There is no error message. And this displays correctly on WolframAlpha - (the second integral with q=3) wolframalpha.com/input/?i=integral_0^%E2%88%9E+tanh%28%CF%80+sqrt%28%CE%BB%29%29+log%281-exp%28-2+%CF%80+3+sqrt%28%CE%BB%2B1%2F4%29%29%29+d%CE%BB&lk=1&a=ClashPrefs_*Math- $\endgroup$
    – user6818
    Commented Dec 8, 2013 at 8:53
  • 2
    $\begingroup$ see !Mathematica graphics You can't write m[Element] Integers, you can't write Sqrt[[Lambda]] etc...as I said, W/A accepts messy human input. But Mathematica needs exact syntax. May be in version 10, when the system becomes WL and unified, then your input will work. But now in version 9.01 it is giving errors. $\endgroup$
    – Nasser
    Commented Dec 8, 2013 at 8:56
  • $\begingroup$ I am doing here "copy as Input Text" - and Mathematica doesn't give any error messages on these - Integrate [ Tanh[ [Pi] Sqrt[[Lambda]]]* Log[ 1 - Exp[-2 [Pi] 3 Sqrt [ [Lambda] + 1/4 ] ]] , {[Lambda], 0, Infinity }] $\endgroup$
    – user6818
    Commented Dec 8, 2013 at 8:58
  • $\begingroup$ Can you see my reformatted pasting of the input? Its not really [pi] its actually "escape p i escape" and so on and so forth $\endgroup$
    – user6818
    Commented Dec 8, 2013 at 9:04
  • $\begingroup$ I copy pasted my text back into the file and there are no errors - I am on Mathematica 9 BTW $\endgroup$
    – user6818
    Commented Dec 8, 2013 at 9:06

1 Answer 1

Reset to default
1
$\begingroup$

This isn't really an answer but it is too long for a comment.

I didn't look at your first integral, but the second is fairly easy to investigate because it only depends on one parameter. I used two Mathematica tools that often help in kind of situation you find yourself in.

Manipulate[
  Plot[Tanh[π Sqrt[λ]] Log[1 - Exp[-2 π q Sqrt[λ + 1/4]]], {λ, 0, 100}],
  {q, 0., 5., 1., Appearance -> "Labeled"}]

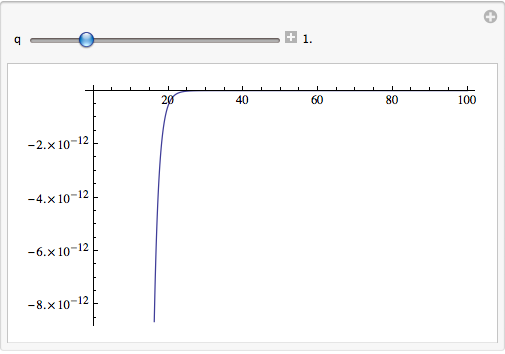
manip.png

Looking a the plot for various values of q suggest the integral converges and goes to zero as q gets large, which in turn suggests that NIntegrate might be fruitful.

Table[NIntegrate[Tanh[π Sqrt[λ]] Log[1 - Exp[-2 π q Sqrt[λ + 1/4]]], {λ, 0, ∞}], {q, 1, 8}]

NIntegrate::slwcon: Numerical integration converging too slowly; suspect one of the following: singularity, value of the integration is 0, highly oscillatory integrand, or WorkingPrecision too small. >>

NIntegrate::ncvb: NIntegrate failed to converge to prescribed accuracy after 9 recursive bisections in λ near {λ} = {0.193495}. NIntegrate obtained -9.30632*10^-14 and 9.31004105035578`*^-19 for the integral and error estimates. >>

{-0.00710267, -0.000110031, -2.62324*10^-6, -7.42753*10^-8, 
 -2.31044*10^-9, -7.62815*10^-11, -2.62445*10^-12, -9.30632*10^-14}

The results from NIntegrate also suggest the integral goes to zero as q goes to ∞, but that Mathematica runs out of computing steam at q = 8 when confined to machine precision reals.

You should make yourself familiar with the use these tools (and others found in Mathematica) to investigate your integration problems.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Just because $f(x)$ decreases with increasing $x$ is no guarantee that $\int _0 ^\infty f(x) dx$ exists. I don't think such a theorem exists. But here if the $N-integrate$ converges it seems to be integrable for at least the values of $q$ that you are testing but its likely that the two things are unrelated. I have never used the manipulate command - let me see if I can learn about it from documentation. [...or can you write a brief explanation of what those {q,0,5,1,Appearance -> Labvelled} mean? :D...] $\endgroup$
    – user6818
    Commented Dec 8, 2013 at 20:26
  • $\begingroup$ Of course, what I did is no proof that your integral exists, nor that Mathematica can solve it. I was just trying to give you some ideas about how you could use Mathematica to get more insight in your problem. $\endgroup$
    – m_goldberg
    Commented Dec 9, 2013 at 6:26

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.