Dt is one way to work with differentials, unless you're after the more advanced functionality Timothy Wofford linked to in his comment. I'm not sure whether you want to do calculus with differentials, for instance in differential equations, or just want them to be formatted in a certain way.
Formatting
For formatting derivatives and differentials, these may be of help:
The derivative and Dt
The differential of a multivariable function:
w = f[x, y, t];
Dt[w]
(* Dt[t] * Derivative[0, 0, 1][f][x, y, t] +
Dt[y] * Derivative[0, 1, 0][f][x, y, t] +
Dt[x] * Derivative[1, 0, 0][f][x, y, t] *)
The (matrix) derivative of f may be obtained with D
D[w, {{x, y, t}}]
(* {Derivative[1, 0, 0][f][x, y, t],
Derivative[0, 1, 0][f][x, y, t],
Derivative[0, 0, 1][f][x, y, t]}
The output of Dt[w] above may also be obtained with
D[w, {{x, y, t}}] . Dt[{x, y, t}]
One can substitute functions for x and y to make them explicitly depending on t, and the Chain Rule is automatically applied (of course):
Dt[w] /. {x -> x[t], y -> y[t]} // Factor // InputForm
(* Dt[t]*
(Derivative[0, 0, 1][f][x[t], y[t], t] +
y'[t]*Derivative[0, 1, 0][f][x[t], y[t], t] +
x'[t]*Derivative[1, 0, 0][f][x[t], y[t], t]) *)
Differential equations and Dt
One can turn expressions in terms of Dt[x] etc. into differential equations:
dtToDE[diffForm_, vars_List, var_] /; MemberQ[vars, var] :=
With[{indep = DeleteCases[vars, var]},
0 == diffForm /.
Flatten[{Thread[Dt /@ indep -> D[Through[indep[var]], var]],
Thread[indep -> Through[indep[var]]]}] /. Dt[var] -> 1
];
dtToDE[diffForm_, vars_List, var_] :=
D[Through[vars[var]], var] ==
Extract[CoefficientList[diffForm, Dt /@ vars] /.
Thread[vars -> Through[vars[var]]],
1 + IdentityMatrix[Length[vars]]];
orthogonalTrajectory[diffForm_, vars_List] :=
diffForm /. Thread[# -> Cross[#]] &[Dt /@ vars];
Examples
An exact differential.
Dt[x^2 + y^2 x - y^2] (* the differential of f *)
ode = dtToDE[Dt[x^2 + y^2 x - y^2], {x, y}, x] (* as a derivative equation *)
DSolve[ode, y[x], x] (* solution to the ODE *)
Solve[x^2 + y^2 x - y^2 == C[1]/2, y] (* solution to f = constant *)
(* 2 x Dt[x] + y^2 Dt[x] - 2 y Dt[y] + 2 x y Dt[y] *)
(* 0 == 2 x + y[x]^2 - 2 y[x] Derivative[1][y][x] + 2 x y[x] Derivative[1][y][x] *)
(* {{y[x] -> -(Sqrt[-2 x^2 - C[1]]/(Sqrt[2] Sqrt[-1 + x]))},
{y[x] -> Sqrt[-2 x^2 - C[1]]/(Sqrt[2] Sqrt[-1 + x])}} *)
(* {{y -> -(Sqrt[-2 x^2 + C[1]]/(Sqrt[2] Sqrt[-1 + x]))},
{y -> Sqrt[-2 x^2 + C[1]]/(Sqrt[2] Sqrt[-1 + x])}} *)
A differential with an integrating factor (pasted from an old test's TeX file, converted to a differential form with Dt):
ode = dtToDE[x dy + y dx + 4 x^3 y^4 dy /. {dx -> Dt[x], dy -> Dt[y]}, {x, y}, x]
sol = DSolve[ode, y[x], x];
ode /. {y'[x] -> Hold[D[y[x], x]]} /. sol // ReleaseHold // Simplify
Times @@ Subtract @@@ DeleteDuplicates@Simplify[x y^2 == x y[x]^2 /. sol] == 0 // Simplify
(* 0 == y[x] + x y'[x] + 4 x^3 y[x]^4 y'[x] -- ODE *)
(* {True, True, True, True} -- all branches of sol satisfy the ODE *)
(* x^2 (4 y^4 - 2 y^2 C[1]) == 1 -- the integral curve (hacked from sol) *)
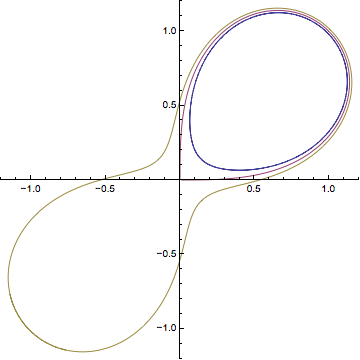
A system of equations, following three orthogonal trajectories to the gradient of a function.
sols = NDSolveValue[
{dtToDE[orthogonalTrajectory[Dt[(x^2 + y^2)^2 - 4 x y], {x, y}], {x, y}, t],
x[0] == #, y[0] == #},
{x, y}, {t, -1.35, 1.35}] & /@ {0.99, 1., 1.01};
ParametricPlot @@ {Through[#[t]] & /@ sols, Flatten @ {t, sols[[1,1]]["Domain"]}}
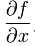
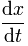



DifferentialD[x]work for your purposes? $\endgroup$Solve[Dt[w[l2[t], lp[t], t], t] == 0, Dt[lp[t], t]], I would then for instance like to see dlp. I tried to use your (adjusted) example instead ofDt[lp[t], t]. It did not work. What do you think? $\endgroup$Notationpackage we can makeDtappear asDifferentialD, and bothDt[w[x[t],y[t],t]]andDt[w[x,y,t]]seem to expand as I would expect. If you are after the functionality of exterior derivatives, you may need to define it. Maybe one of these packages can help. 1, 2, 3 $\endgroup$