I am working on a Mathematica project where I want to plot the group delay. The problem is this code is running too slow. Is there any possibility of speeding up the code? Here is the original code:
Clear["Global`*"]
plotset = {FrameStyle -> Directive[Thickness[0.004]],
TicksStyle -> Directive[Black, 18]};
plotset2 = FrameTicksStyle -> {{Directive[Black, 18], Directive[FontOpacity -> 0, FontSize -> 0]}, {Directive[Black, 18], Directive[FontOpacity -> 0, FontSize -> 0]}};
G = 0.5 k1; \[Theta] = 0;
\[CapitalPhi]m = 0;
B = 5*10^-10;
\[Gamma] = 2*\[Pi]*28*10^9;
N1 = Sqrt[3*10^16];
Em = Sqrt[5]/4*(\[Gamma])*N1*B;
\[Omega]c = 2*\[Pi]*7.86*10^9;
\[Omega]b = 2*\[Pi]*11.42*10^6;
\[CapitalDelta]a = \[Omega]b;
k1 = \[Pi]*3.35*10^6;
k2 = k1;
J = 0.5 k1;
kb = 300*\[Pi];
km1 = \[Pi]*1.12*10^6;
km2 = \[Pi]*1.12*10^6;
\[CapitalDelta]m1 = \[Omega]b;
\[CapitalDelta]m2 = \[Omega]b;
\[HBar] = 1.054*10^-34;
Subscript[g, 1] = 2 k1;
Subscript[g, 2] = 2 k1;
wl = 2*\[Pi]*7.9*10^9;
sss = Sqrt[p1/(\[HBar]*wl)];
E1 = sss;
Ep = 0.0001 E1;
\[Eta] = 0.5 ;
gmb = 2*Pi;
h1 = -I*\[CapitalDelta]a + I*\[Delta] - k1;
SuperStar[h1] = I*\[CapitalDelta]a - I*\[Delta] - k1;
h2 = -I*\[CapitalDelta]a - I*\[Delta] - k1;
SuperStar[h2] = I*\[CapitalDelta]a + I*\[Delta] - k1;
h3 = -I*\[CapitalDelta]a + I*\[Delta] + k2;
SuperStar[h3] = I*\[CapitalDelta]a - I*\[Delta] + k2;
h4 = -I*\[CapitalDelta]a - I*\[Delta] + k2;
SuperStar[h4] = I*\[CapitalDelta]a + I*\[Delta] + k2;
h5 = -I*\[Omega]b + I*\[Delta] - kb;
SuperStar[h5] = I*\[Omega]b - I*\[Delta] - kb;
h6 = -I*\[Omega]b - I*\[Delta] - kb;
SuperStar[h6] = I*\[Omega]b + I*\[Delta] - kb;
h7 = -I*\[CapitalDelta]m1 + I*\[Delta] - km1;
SuperStar[h7] = I*\[CapitalDelta]m1 - I*\[Delta] - km1;
h8 = -I*\[CapitalDelta]m1 - I*\[Delta] - km1;
SuperStar[h8] = I*\[CapitalDelta]m1 + I*\[Delta] - km1;
h9 = -I*\[CapitalDelta]m2 + I*\[Delta] - km2;
SuperStar[h9] = I*\[CapitalDelta]m2 - I*\[Delta] - km2;
h10 = -I*\[CapitalDelta]m2 - I*\[Delta] - km2;
SuperStar[h10] = I*\[CapitalDelta]m2 + I*\[Delta] - km2;
\[Alpha] = ((k1 + I \[CapitalDelta]a)*((k2 - I \[CapitalDelta]a)) -
J^2)/(k2 - I \[CapitalDelta]a);
\[Beta] = (k1 - I \[CapitalDelta]a) + Subscript[g, 1]^2/(-I \[CapitalDelta]m1 + km1) + Subscript[g,2]^2/(-I \[CapitalDelta]m2 + km2) -
J^2/(k2 + I \[CapitalDelta]a);
\[Gamma]1 = ((\[Alpha]*\[Beta] - 4 G^2)/\[Beta]) + (Subscript[g,1]^2/(I \[CapitalDelta]m1 + km1)) + (Subscript[g, 2]^2/(I \[CapitalDelta]m2 + km2));
\[Psi]1 = (1/\[Gamma]1)*(E1 Sqrt[2*k1* \[Eta]] + ((2*G*Exp[I*\[Theta]])/\[Beta]*(E1 Sqrt[2*k1* \[Eta]])));
\[Psi]2 = (-I*Subscript[g, 2])/(km1 + I \[CapitalDelta]m1);
ms = \[Psi]1*\[Psi]2;
F = ms*gmb;
\[Alpha]1 = h7*SuperStar[h6]* h5 + F^2*(SuperStar[h6] - h5) // Simplify;
\[Alpha]2 = SuperStar[h2] + J^2/SuperStar[h4] + Subscript[g,1]^2/SuperStar[h10] // Simplify;
\[Alpha]3 = \[Alpha]2*SuperStar[h8] + Subscript[g, 2]^2 // Simplify;
\[Alpha]4 = 2*G*Exp[-I*\[Theta]]*I*Subscript[g, 2] +
I*Subscript[g, 2]*\[Alpha]2 // Simplify;
\[Alpha]5 = (-I*Subscript[g, 2]*\[Alpha]4 -
2*G*Exp[-I*\[Theta]]*\[Alpha]3) // Simplify;
\[Alpha]6 = I*Subscript[g, 2]*h7*\[Alpha]2 // Simplify;
\[Alpha]7 = -I*Subscript[g, 2]*\[Alpha]5 +
I*Subscript[g, 2]*\[Alpha]2*\[Alpha]3 // Simplify;
\[Alpha]8 =
I*Subscript[g, 2]*\[Alpha]6 + \[Alpha]2*\[Alpha]3*h7 // Simplify;
\[Alpha]9 = SuperStar[h8]*\[Alpha]2*\[Alpha]3 // Simplify;
\[Xi]1 = (Subscript[g, 2]^2*\[Alpha]2 - \[Alpha]3*\[Alpha]2) //
Simplify;
\[Xi]2 = F^2*(SuperStar[h6] - h5)*\[Xi]1 -
h5*SuperStar[h6]*\[Alpha]9 // Simplify;
\[Alpha]10 = \[Alpha]1*\[Alpha]9 -
F^2*(SuperStar[h6] - h5)*\[Alpha]8 // Simplify;
\[Alpha]11 = (I*Subscript[g, 2]* h5*SuperStar[h6]*\[Alpha]9 -
F^2*(SuperStar[h6] - h5)*\[Alpha]7) // Simplify;
\[Xi]3 = ((\[Alpha]6*\[Xi]2 )/(\[Alpha]2*\[Alpha]3*\[Alpha]10) + (
I*Subscript[g, 2]*\[Alpha]2)/(\[Alpha]2*\[Alpha]3)) // Simplify;
\[Alpha]12 = ((\[Alpha]5*\[Alpha]10 +
\[Alpha]6*\[Alpha]11)/(\[Alpha]10*\[Alpha]2*\[Alpha]3)) // Simplify;
\[Alpha]13 = h1 + J^2/h3 - (I*Subscript[g, 1])/h9 - (I*Subscript[g, 2]*\[Alpha]11)/\[Alpha]10 + 2*G*Exp[-I*\[Theta]]*\[Alpha]12 // Simplify;
\[Xi]4 = ((-I*Subscript[g, 2]*\[Xi]2 )/\[Alpha]10 + 2*G*Exp[-I*\[Theta]]*\[Xi]3) // Simplify;
A1 = (\[Xi]4*Em*Exp[I*\[CapitalPhi]m] - Sqrt[2*\[Eta]*k1]*Ep)/\[Alpha]13 // Simplify;
Tp = (Sqrt[2*\[Eta]*k1]*A1)/Ep // Simplify;
rr = Abs[(1 - ( Sqrt[2*\[Eta]*k1]*(\[Xi]4*Em*Exp[I*\[CapitalPhi]m] -
Sqrt[2*\[Eta]*k1]*Ep))/(Ep *\[Alpha]13))]^2 // Simplify;
v = ComplexExpand[Im[Tp]] // Simplify;
R = ComplexExpand[Re[Tp]] // Simplify;
\[Phi]12 = ArcTan[v/R] // Simplify;
pp1 = N[D[\[Phi]12, \[Delta]]] // Simplify;
\[Delta] = \[Omega]b;
Plot[Evaluate[pp1], {p1, 0.001, 0.01}, Frame -> True,
PlotLegends -> Placed[LineLegend[{""}, LegendLayout -> {"Column", 1},
LegendMarkerSize -> {{30, 20}}], {Right, 0.90}, Pane[#, 450, Alignment -> Right] &], ImageSize -> 450, PlotStyle -> {Blue, Dashing[Large], Thickness[0.007]}, GridLines -> Automatic,
FrameLabel -> {Style["", 18, Bold], Style["", 18, Bold]},
Evaluate@plotset, Evaluate@plotset2, Axes -> True, PlotRange -> All]
This is the link to my group delay formula.

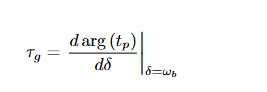
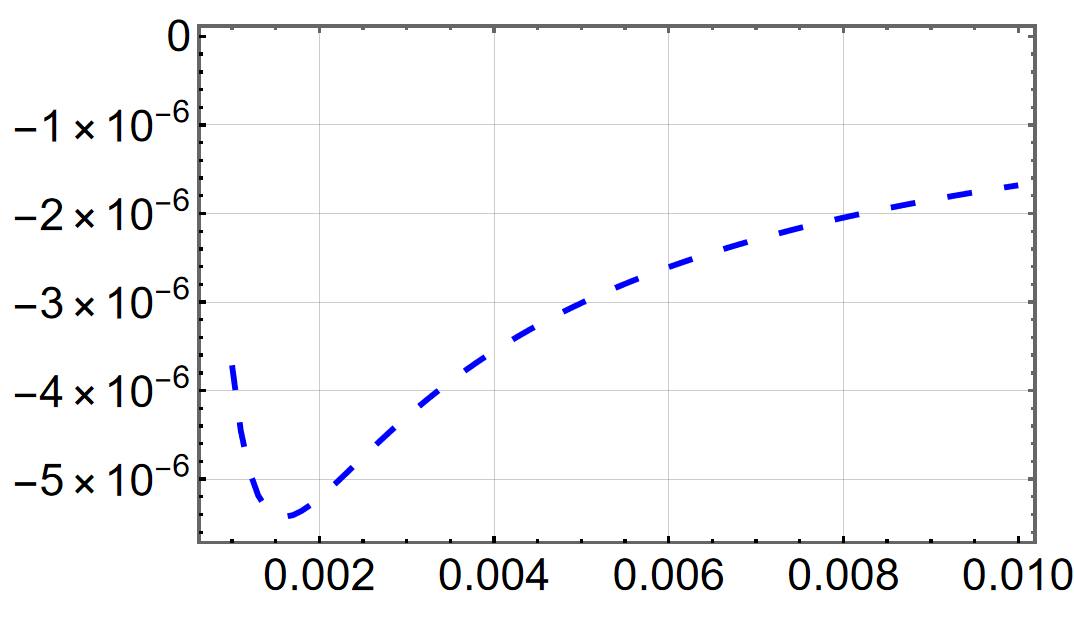
Simplifyappear to be just taking up time, leaving in the very last one may help. TheComplexExpandmay not be doing anything. I might try without that and see if it really changes the result or not. When aPlotis too slow the first thing I try isListPlot[Table[pp1,{p1,0.001,0.01,0.001}],Joined->True]and if that is too slow then I tryp1=0.001;pp1and see how long that takes. Then I expectPlotwill take 100 or 1000 times longer than that to plot the 100 or 1000 points. $\endgroup$