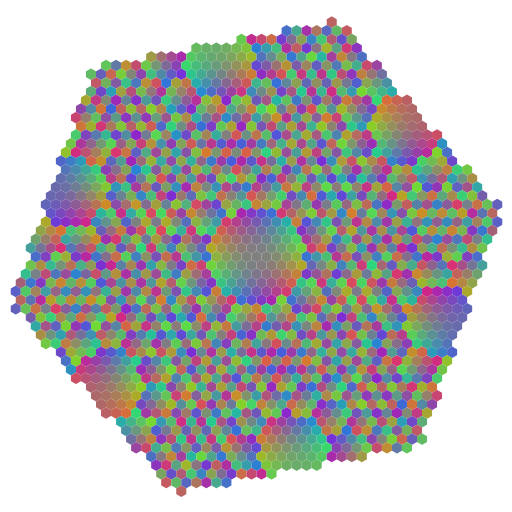
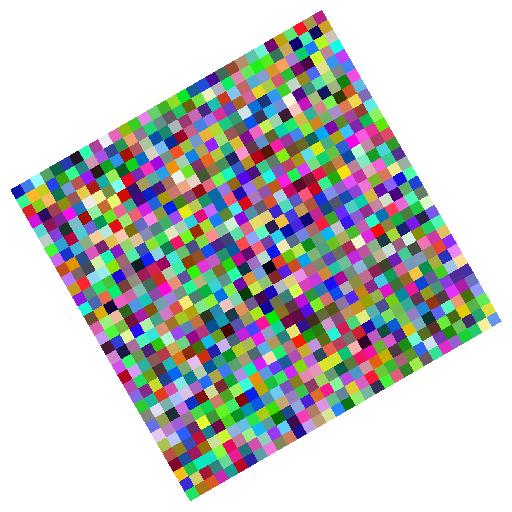
I'm trying to plot a function taking discrete values on hexagonal lattice.
However, there are visible white seams between hexagonal pixels. As a comparison, the built-in Raster primitive does not produce seams, even if rotated.
Image[Graphics@GeometricTransformation[
Raster@RandomReal[1., {32, 32, 3}],RotationMatrix[2 \[Pi]/3]
],
ImageSize -> {512, 512}]
Here's the code I used to produce hex-plot:
(*algebraic definitions*)
RandomPrime3np1[range : {_Integer, _Integer}] := Block[{p},
Do[p = RandomPrime[range];
If[Mod[p, 3] == 1, Return[p]], {\[Infinity]}]
]
EisensteinFactorizableQ[q_Integer] :=
PrimePowerQ[q] && Mod[q, 3] == 1
EisensteinMat = {{1, 0}, {-1, Sqrt[3]}/
2};(*point_in_hex == point_in_rect.EisensteinMat*)
EisensteinNorm[{x_, y_}] := (x^2 - x y + y^2)
EisensteinPeriod[q_Integer /; EisensteinFactorizableQ[q]] :=
Block[{m, n}, {m, n} /.
FindInstance[{m^2 - m n + n^2 == q, 0 < m < q, 0 < n < q,
m < n}, {m, n}, Integers][[1]]]
HexReduce[{x0_, y0_}] := Block[{x = x0, y = y0},
With[{s = Floor[(x - y + 1)/2]}, x -= s; y += s;];
With[{s = 3*Floor[(x + y + 1)/6]}, x -= s; y -= s];
If[Min[x, y] > 2, x -= 3; y -= 3
, If[Max[x, y] > 1, If[x > y, x -= 2; y -= 1, x -= 1; y -= 2]]
]; {x, y}]
EisensteinReduce[xy : {_Integer, _Integer}, q_Integer] :=
With[{mat1 = {{1, 0}, {-1, Sqrt[3]}/2}}, With[
{ep = EisensteinPeriod[q] .
mat1 . {{Sqrt[3], -1}, {1, Sqrt[3]}}/(2 Sqrt[3])},
With[{mat = mat1 . Inverse@{ep, RotationMatrix[2 \[Pi]/3] . ep}},
Simplify[HexReduce[xy . mat] . Inverse[mat]]
]]]
EisensteinReduce[n_Integer, q_Integer] := EisensteinReduce[{n, 0}, p]
EisensteinRange[q_Integer /; EisensteinFactorizableQ[q]] :=
Table[EisensteinReduce[i, q], {i, 0, q - 1}]
(*plot function over F_p on hexagonal lattice*)
p = RandomPrime3np1[{1, 2}*1000];
pts = EisensteinRange[p];
hexPts = {{2, 1}, {1, 2}, {-1, 1}, {-2, -1}, {-1, -2}, {1, -1}};
edgePts =
DeleteDuplicates[Flatten[Outer[Plus, pts*3, hexPts, 1], 1]];
edgePts2Idx =
Association @@ MapThread[Rule, {edgePts, Range@Length@edgePts}];
faceList = Table[With[{pt3 = 3*pt},
Table[edgePts2Idx[pt3 + ipt], {ipt, hexPts}]], {pt, pts}];
colorList =
With[{ep =
EisensteinPeriod[p] . EisensteinMat . RotationMatrix[\[Pi]/6]*
Sqrt[1/3]},
With[{tmat =
N[(1/2)*EisensteinMat . Inverse[{ep, ep . {{0, 1}, {-1, 0}}}] .
Normalize /@ {{-1, 1, 0}, {-1, -1, 2}}]},
(# + {1, 1, 1}/2) & /@ (pts . tmat)]];
fnList = Table[Mod[i^2, p], {i, 0, p - 1}];
Graphics[GeometricTransformation[#, Transpose@EisensteinMat] &@
GraphicsComplex[
edgePts,
Flatten[MapThread[{RGBColor @@ #1, Polygon@#2} &, {colorList[[
fnList + 1]], faceList}]]
]]
How do I remove seams between hex-pixels?
Some backgrounds:
Let prime number $p = 3n + 1 (n \in \mathbb{Z})$, let $\mathbb{Z}[\omega]$ be ring of eisenstein integers where $\omega = e^{\frac{2 \pi i}{3}}$. There exists $p_{\omega} \in \mathbb{Z}[\omega]$ where $|p_{\omega}|^2 = p$. The field $\mathbb{Z}[\omega]/p_{\omega} \mathbb{Z}[\omega]$ is isomorphic to $\mathbb{F}_p$. Hence it's possible to "embed" elements of $\mathbb{F}_p$ into hexagonal lattice.
Let $y = f(x)$ where $x, y \in \mathbb{F}_p$. For each hex-pixel, its coordinate is hex-embedding of $x$ and its color is hex-embedding of $y$ placed into hex-slice of RGB unit cube.