Using FindRoot[] to find an inflection point

Question

FindRoot[
  {Derivative[2][SinIntegral[x]], 0 < x < (Pi)},
  x
]

I hoped that my code would return a value for x=0 but it is returning an error: "search specification should be a list with 1 to 5 elements"

edit: I want to iterate this multiple times so it will be helpful to be able to find the root within a given range for x

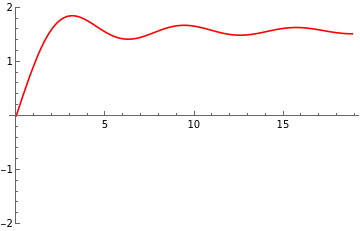
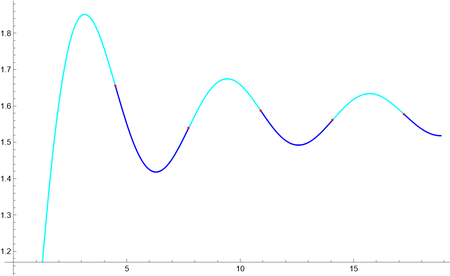
To see the shape: Plot[SinIntegral''[x], {x, 0, 6 \[Pi]}] and then choose a suitable initial guess: FindRoot[SinIntegral''[x], {x, 5}] — Syed, Commented Jan 18, 2022 at 5:26
For the interval 0 <= x <= 6 Pi use sol = FindRoot[SinIntegral''[x], {x, #}] & /@ {1/20, 5, 8, 11, 14, 17} — Bob Hanlon, Commented Jan 18, 2022 at 6:16

cvgmt · Accepted Answer · 2022-01-18 14:55:42Z

4

Edit

FindRoot[Derivative[2][SinIntegral][x], {x, 1/2}]

{x -> 1.15898*10^-10}

sol=NSolve[{Derivative[2][SinIntegral][x], 0 < x < 6 Pi}, x]

{{x -> 4.49341}, {x -> 7.72525}, {x -> 10.9041}, {x -> 14.0662}, {x -> 17.2208}}

The concave and convex parts as below.

Plot[SinIntegral[x], {x, 0, 6 Pi}, Mesh -> {sol}, MeshStyle -> Red, 
 MeshShading -> {Cyan, Blue}]

answered Jan 18, 2022 at 6:17

cvgmt

84.1k6 gold badges97 silver badges179 bronze badges

$\begingroup$ Limit[SinIntegral''[x], x -> 0] also evaluates to 0 $\endgroup$
– Bob Hanlon
Commented Jan 18, 2022 at 6:23
$\begingroup$ The important thing for the OP to keep in mind here is that FindRoot (used in their original code) is designed to find one root and one root only, while NSolve can (sometimes) find all of the roots in a given range. $\endgroup$
– Michael Seifert
Commented Jan 18, 2022 at 14:49

Add a comment |

1 Answer 1