I am trying to calculate eigenvalues of a sparse matrix with only two distinct non-zero elements, here Alpha and Beta, which are both negative reals. Mathematica returns some complex expressions with Root[] values when using the Eigenvalues[] command on the following matrixA:
In all cases the matrices are symmetric and real and hence have real eigenvalues.
matrixA={
{α, β, 0, 0, 0, 0, β, 0, 0, β},
{β, α, β, 0, 0, 0, 0, 0, 0, 0},
{0, β, α, β, 0, 0, 0, 0, 0, 0},
{0, 0, β, α, β, 0, 0, 0, 0, 0},
{0, 0, 0, β, α, β, 0, 0, 0, 0},
{0, 0, 0, 0, β, α, β, 0, 0, 0},
{β, 0, 0, 0, 0, β, α, β, 0, 0},
{0, 0, 0, 0, 0, 0, β, α, β, 0},
{0, 0, 0, 0, 0, 0, 0, β, α, β},
{β, 0, 0, 0, 0, 0, 0, 0, β, α}
}
For comparison, with all the other similar matrices I've tried (see below e.g. matrixB) Mathematica will put out simple decimal approximations (using Eigenvalues[matrixB] // N // Simplify)
Can anyone point out a way to get expressions for the matrixA as simple as for matrixB?
And yes, the desired simple answers for matrixA do exist, I can get them with other programs, but I want to use Mathematica!
I should add that I already have already used $Assumptions = α<0 && β <0 at the top of my worksheet.
matrixB={
{α, β, 0, 0, 0, 0, 0, 0, 0, β},
{β, α, β, 0, 0, 0, 0, 0, 0, 0},
{0, β, α, β, 0, 0, 0, β, 0, 0},
{0, 0, β, α, β, 0, 0, 0, 0, 0},
{0, 0, 0, β, α, β, 0, 0, 0, 0},
{0, 0, 0, 0, β, α, β, 0, 0, 0},
{0, 0, 0, 0, 0, β, α, β, 0, 0},
{0, 0, β, 0, 0, 0, β, α, β, 0},
{0, 0, 0, 0, 0, 0, 0, β, α, β},
{β, 0, 0, 0, 0, 0, 0, 0, β, α}
}



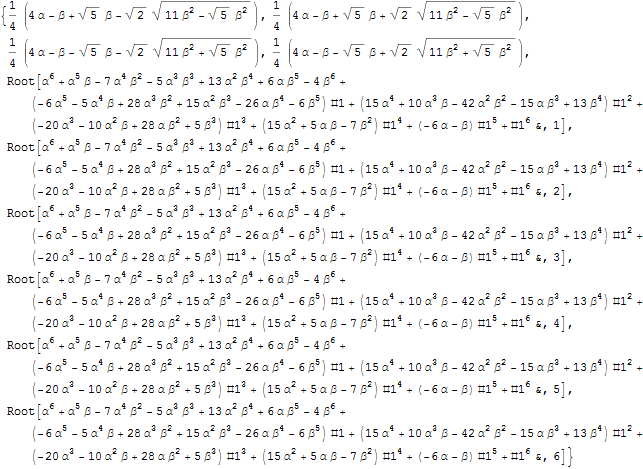
//NformatrixB: there is an analytical solution. If the highest power of the eigenvalues for matrixA is higher than those for matrixB, they won't be "as simple". $\endgroup$