Too many symbolic constants usually turn out to be obstructive to calculate integrals symbolically. Another problem comes up when no restriction for parameters is given. We can set specific values to a few constants using With and (or) restrict constants with an option Assumptions in Integrate e.g.
With[{n = 1, a = 1, z = 1},
Table[{t, Integrate[ Exp[-L y] Gamma[t, (Sqrt[z (y + w)/a])/n],
{y, 0, Infinity},
Assumptions -> L > 0 && w > 0]},
{t, 4}]]
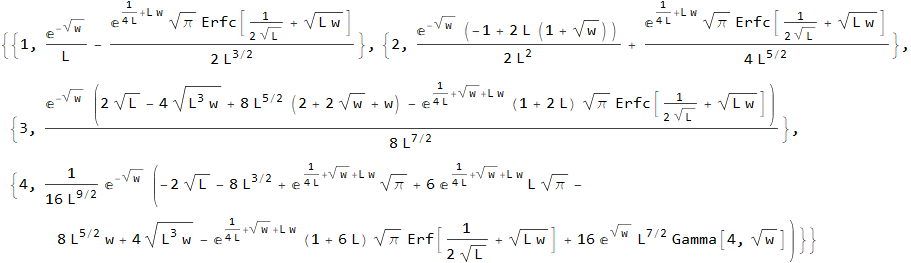
We could also restrict appropriately all constant but one, however then Mathematica cannot provide symbolic results for certain values of t, e.g. for t = 3 the integral is not found
Table[{t, Integrate[ Exp[-L y] Gamma[t, (Sqrt[z (y + w)/a])/n],
{y, 0, Infinity},
Assumptions -> L > 0 && n > 0 && a > 0 && z > 0 && w > 0]},
{t, 4}]

Perhaps next versions of the system should provide more general results.


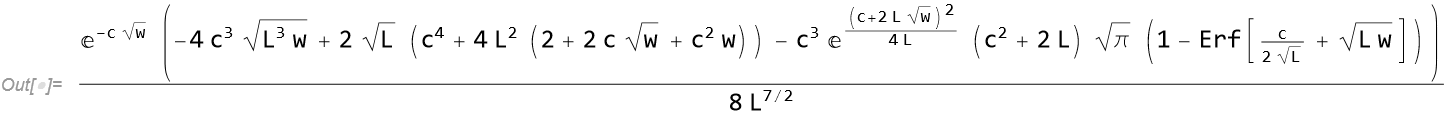
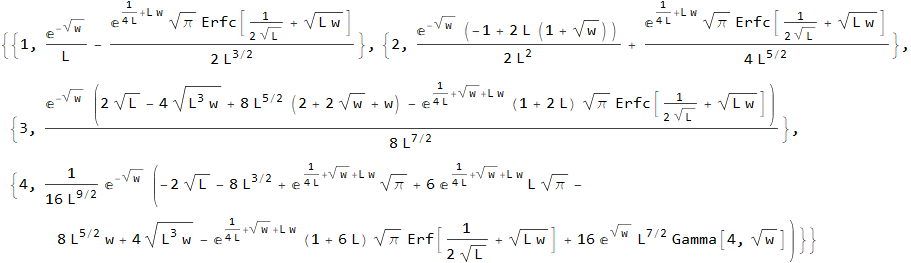

NIntegratewith specific values of $L$, $t$, $z$, $w$, $a$, and $n$? $\endgroup$probability-or-statistics. More details regarding constants is crucial. $\endgroup$