How to plot spherical harmonics using two primary colors?

Question

I did go through Density plot on the surface of sphere where great examples are provided. What I am very much interested is in plotting spherical harmonics (real/imaginary or lets say just the assoc. Legendre Polynomials) on a 2-sphere (of unit radius) but just using two primary colors, say blue and red. I would like to use red when the value of the function goes to zero and blue when it peaks (to whatever it peaks) and a nice combination of these two somewhere in the middle. Is that possible? Rainbow doesn't help at all in visualizing. Have spent a lot of time but with no results! Any help would be highly appreciated.

I used

SphericalPlot3D[1, {θ, 0, π}, {Φ, 0, 2 π}, 
     ColorFunction -> Function[{x, y, z, θ, Φ, r}, 
         ColorData["Rainbow"][Re@SphericalHarmonicY[1,0,θ,Φ]]], 
     ColorFunctionScaling -> False, Mesh -> False, Boxed -> False, Axes -> False]

Answer 1

Clear["Global`*"]

SphericalHarmonicY[1, 0 , θ, Φ] is real for real {θ, Φ}

FunctionDomain[
 SphericalHarmonicY[1, 0, θ, Φ], {θ, Φ}]

(* True *)

The min and max values are

{min, max} = #[{Re@SphericalHarmonicY[1, 0, θ, Φ], 
     0 <= θ <= Pi, 
     0 <= Φ <= 2 Pi}, {θ, Φ}] & /@ {MinValue, 
   MaxValue}

(* {-(Sqrt[(3/π)]/2), Sqrt[3/π]/2} *)

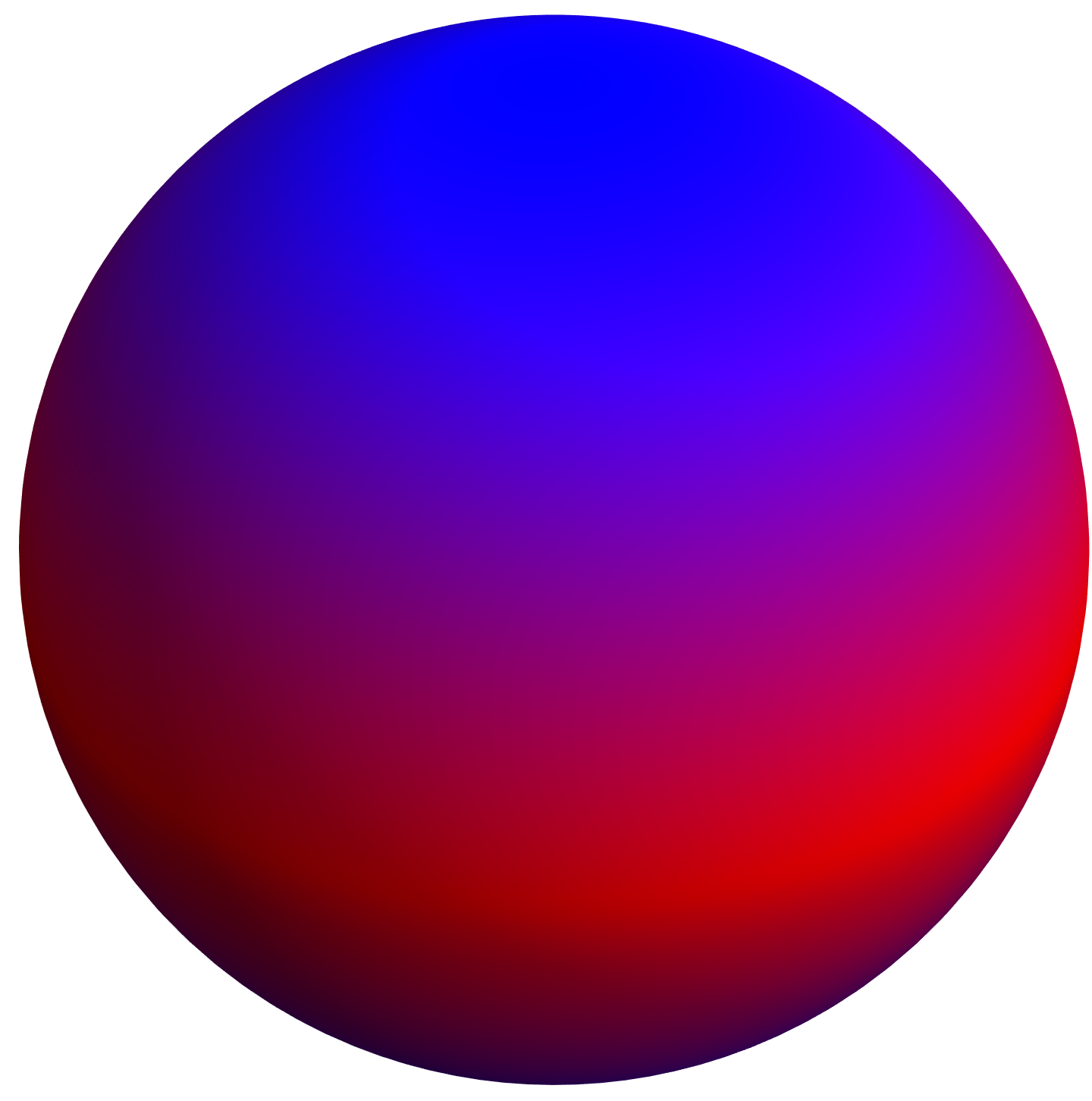
For Red for zero and Blue at both of the extremes:

SphericalPlot3D[1, {θ, 0, π}, {Φ, 0, 2 π},
 ColorFunction -> Function[{x, y, z, θ, Φ, r},
   Blend[{Blue, Red, Blue},
    Rescale[SphericalHarmonicY[1, 0, θ, Φ], {min, max}]]],
 PlotPoints -> 100,
 ColorFunctionScaling -> False,
 Mesh -> False,
 Boxed -> False,
 Axes -> False]

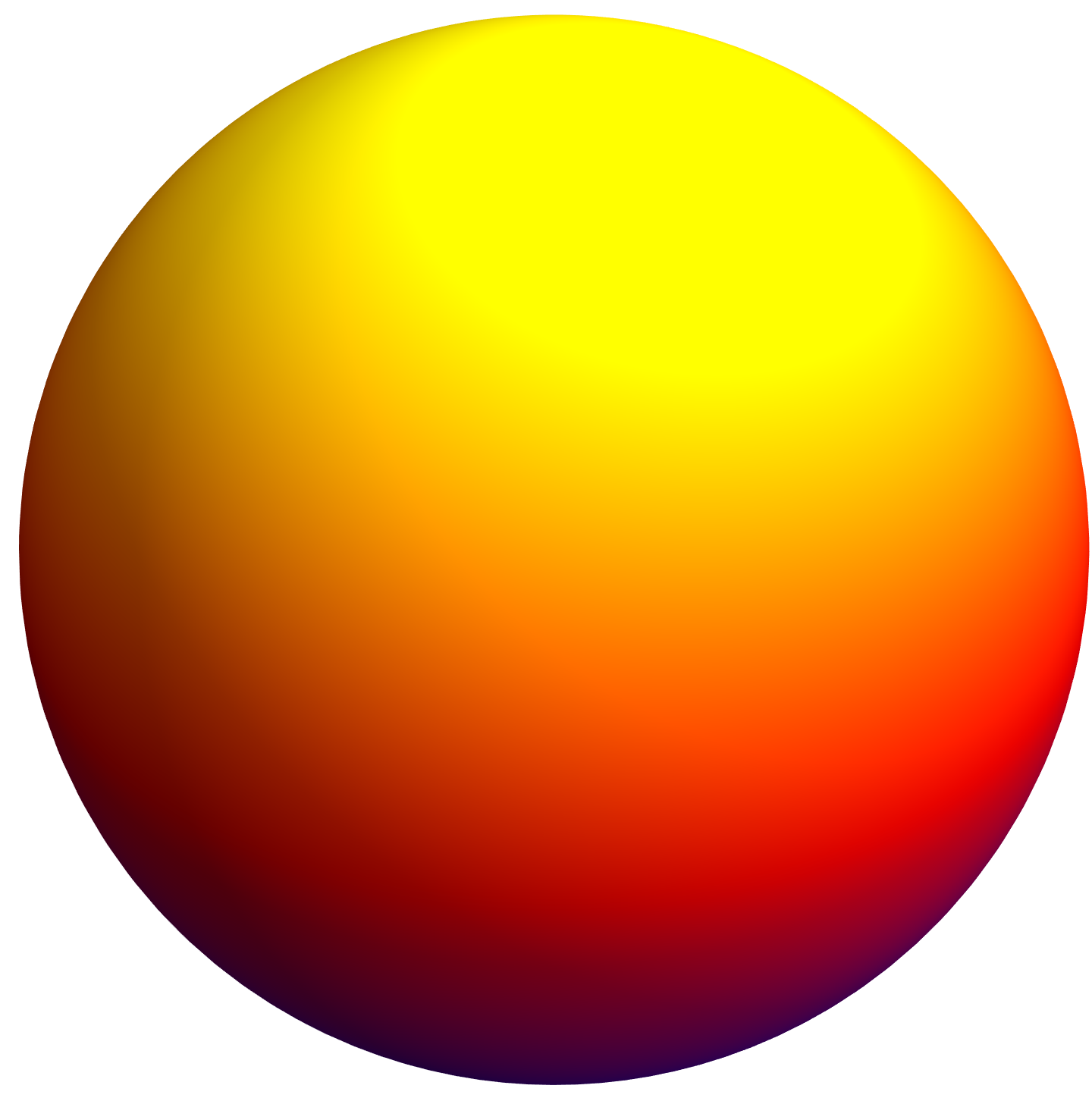
For Blue at negative extreme, Red at zero, and Yellow at positive extreme:

SphericalPlot3D[1, {θ, 0, π}, {Φ, 0, 2 π},
 ColorFunction -> Function[{x, y, z, θ, Φ, r},
   Blend[{Blue, Red, Yellow},
    Rescale[SphericalHarmonicY[1, 0, θ, Φ], {min, max}]]],
 PlotPoints -> 100,
 ColorFunctionScaling -> False,
 Mesh -> False,
 Boxed -> False,
 Axes -> False]

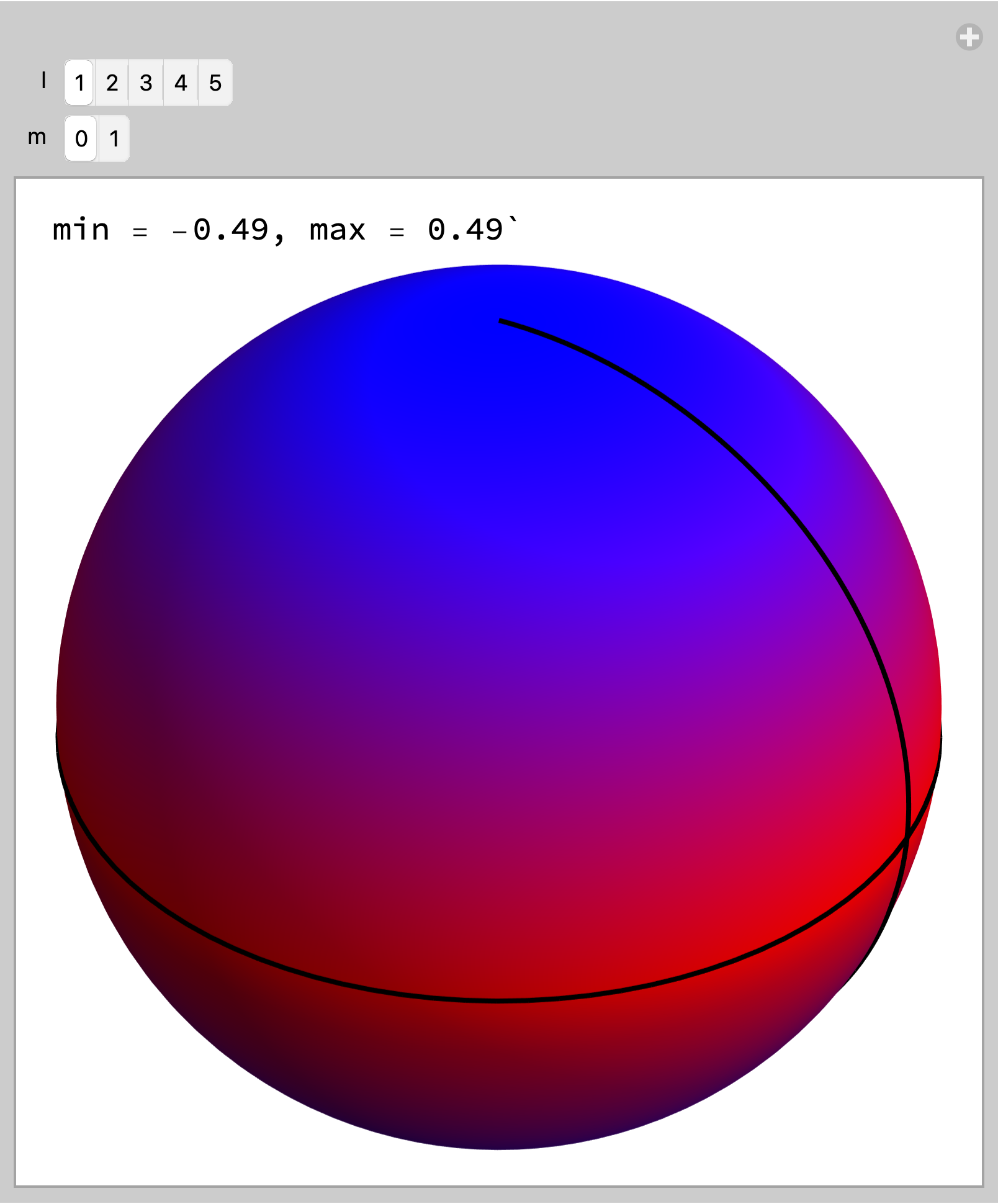
EDIT: For variable {l, m} in SphericalHarmonicY[l, m, θ, Φ]

Manipulate[
 Module[{min, max},
  m = Min[m, l];
  {min, max} = 
   N[#[{Re@SphericalHarmonicY[l, m, θ, Φ],
        0 <= θ <= Pi, 0 <= Φ <= 2 Pi}, {θ, Φ},
       WorkingPrecision -> 15] & /@
     {NMinValue, NMaxValue}];
  Column[{
    StringForm["min = ``, max = ``",
     Round[min, 0.01], Round[max, 0.01]],
    SphericalPlot3D[
     1, {θ, 0, π}, {Φ, 0, 2 π}, 
     ColorFunction -> Function[{x, y, z, θ, Φ, r},
       Blend[{Blue, Red, Blue},
        Rescale[
         Re@SphericalHarmonicY[l, m, θ, Φ], {min, 
          max}]]],
     PlotPoints -> 100,
     ColorFunctionScaling -> False,
     Mesh -> {{0.}},
     MeshFunctions -> {Function[{x, y, z, θ, Φ, 
         r},
        Re@SphericalHarmonicY[l, m, θ, Φ]]},
     MeshStyle -> {Black, Thick},
     Boxed -> False,
     Axes -> False,
     ImageSize -> Medium]}]],
 {{l, 1}, Range[5], ControlType -> SetterBar},
 {{m, 0}, Range[0, l], ControlType -> SetterBar}]