3
$\begingroup$

Is there a built-in function in Mathematica for this function?

\begin{equation} {\displaystyle \operatorname {Circ} (r)=\left\{{\begin{array}{rl}1,&{\text{if }}r<{1}\\{\frac {1}{2}},&{\text{if }}r={1}\\0,&{\text{if }}r>{1}.\end{array}}\right.} \end{equation} where $r=\sqrt{x^2+y^2}$

I use:

UnitBox[1/2*Sqrt[x^2 + y^2]]

and the function is equivalent, but Mathematica doesn't do the Fourier transform in my system (MMA v. 11.0).

Improve this question
$\endgroup$
5
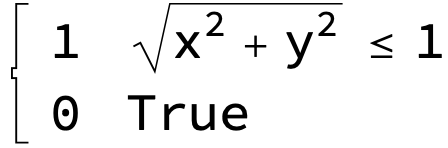
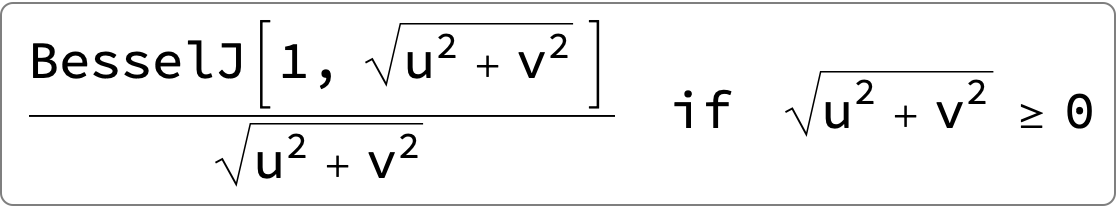
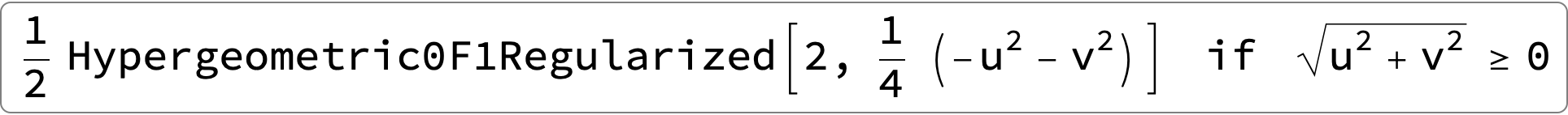
  • $\begingroup$ FourierTransform[UnitBox[1/2*Sqrt[x^2 + y^2]], {x, y}, {u, v}] returns a result on my machine. $\endgroup$
    – Greg Hurst
    Commented Jan 22, 2021 at 14:14
  • $\begingroup$ MMA version 12.1 on PC returns: 1/2 Hypergeometric0F1Regularized[2, 1/4 (-u^2 - v^2)] $\endgroup$
    – Daniel Huber
    Commented Jan 22, 2021 at 14:21
  • $\begingroup$ well, my computer is simple, maybe because of this he doesn´t do the transform, my wolfram version is 11.0 $\endgroup$
    – user740332
    Commented Jan 22, 2021 at 14:26
  • $\begingroup$ the fourier transform of circ is a bessel function J1(2*Pi*r)/r $\endgroup$
    – user740332
    Commented Jan 22, 2021 at 14:27
  • $\begingroup$ Welcome to Mathematica.SE! I suggest the following: 1) As you receive help, try to give it too, by answering questions in your area of expertise. 2) Take the tour! 3) When you see good questions and answers, vote them up by clicking the gray triangles, because the credibility of the system is based on the reputation gained by users sharing their knowledge. Also, please remember to accept the answer, if any, that solves your problem, by clicking the checkmark sign! $\endgroup$
    – Michael E2
    Commented Jan 22, 2021 at 17:15

2 Answers 2

Reset to default
4
$\begingroup$ 
$Version

(* "12.2.0 for Mac OS X x86 (64-bit) (December 12, 2020)" *)

The functions are equivalent for integration but they have different values for r == 1. Use PiecewiseExpand to convert the expression

Assuming[Element[{x, y}, Reals],
 UnitBox[1/2*Sqrt[x^2 + y^2]] // PiecewiseExpand]

enter image description here

Assuming[Element[{x, y}, Reals],
 FourierTransform[
  UnitBox[1/2*Sqrt[x^2 + y^2]] // PiecewiseExpand, {x, y}, {u, v}]]

enter image description here

% // FullSimplify

enter image description here

Improve this answer
$\endgroup$
4
$\begingroup$

You can obtain a result in terms of Bessel functions using FunctionExpand:

FunctionExpand@
 FourierTransform[UnitBox[1/2*Sqrt[x^2 + y^2]], {x, y}, {u, v}]

(* Out: BesselI[1, Sqrt[-u^2 - v^2]]/Sqrt[-u^2 - v^2] *)
Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.