Issues with Plotlegend

Question

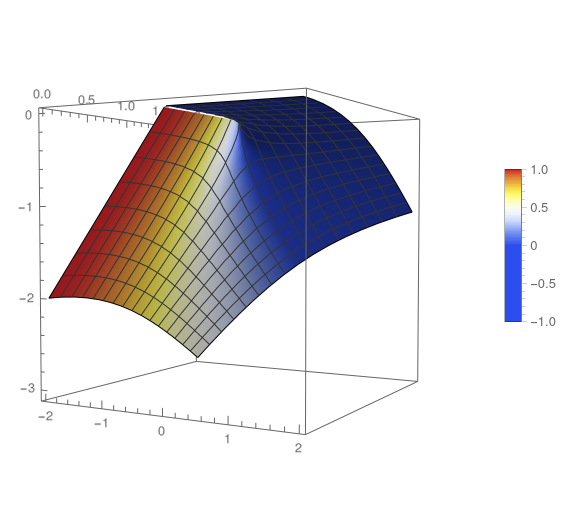
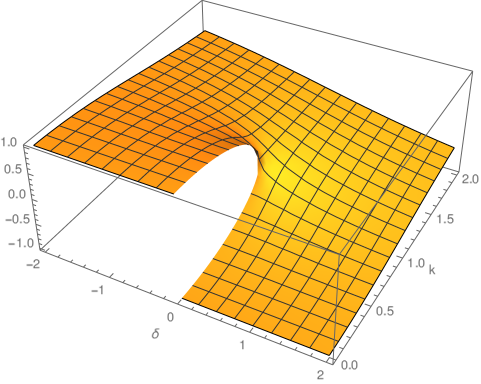
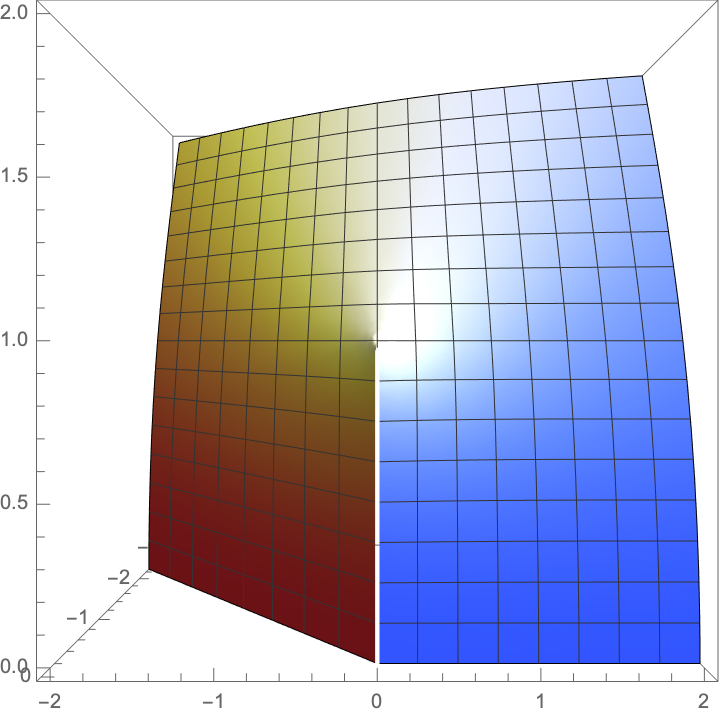
I'm making a 3D plot of the function En1 and I'm attributing its color to the sz1 function below, which contains values spanning from -1 to 1.

En1[δ_, g1_, g2_, k_] := 1/2(-I g1 + I g2 -Sqrt[-(g1 + g2 - 2 k + I δ) (g1 + g2 + 2 k + Iδ)] + δ)

vec1[δ_, g1_, g2_,k_] := {{-((I g1 + I g2 + Sqrt[-(g1 + g2 - 2 k + I δ) (g1 + g2 + 2 k + I δ)] - δ)/1), 2 k}}

vec1d[δ_, g1_, g2_,k_] := {{(I g1 + I g2 - Sqrt[-(g1 + g2 - 2 k - I δ) (g1 + g2 + 2 k - I δ)] + δ)/1, 2 k}}

σz = PauliMatrix[3];
σ0 = IdentityMatrix[2];

sz1[δ_, g1_, g2_, k_] := Flatten[vec1d[δ, g1, g2, k]. σz . Transpose[vec1[δ, g1, g2, k]]][[1]]/Flatten[vec1d[δ, g1, g2, k].Transpose[vec1[δ, g1, g2, k]]][[1]]

g1 = 1;  g2 = 1;

Plot3D[
  {Re[En1[δ, g1, g2, k]]}, 
  {δ, -2, 2}, {k, 0, 2},
  ColorFunction -> Function[{δ, k, z}, ColorData["TemperatureMap"][sz1[δ, g1, g2, k]]], 
  ColorFunctionScaling -> False, 
  PlotLegends -> BarLegend[{ColorData["TemperatureMap"], {-1, 1}}], 
  BoxRatios -> {1, 1, 1}
]

As we can see, the color is responding to the function sz1. However, there is an issue with my legend since the gradient of color seems not linear. Is there a way to impose the legend color to vary linearly from -1 to 1?

If we plot sz1 we see that it's odd with respect to the $\delta$ for a fixed k, i.e.,

Plot3D[{sz1[δ, g1, g2, k]}, {δ, -2, 2}, {k, 0, 2}, AxesLabel -> {"δ", "k"}]

However, the color of the 3D plot of Re[En1] is not odd with respect to the color. Do you see any reason for this?

Answer 1

You can explicitly rescale the color function in the legend:

BarLegend[{ColorData["TemperatureMap"][Rescale[#, {-1, 1}]] &, {-1, 1}}]

Without that, it appears the legend uses the default range 0 to 1 for the color function. So values below zero are clipped to the color corresponding to zero.

To address your revised question, you can also rescale the color function in the plot:

ColorFunction -> Function[{\[Delta], k, z},
   ColorData["TemperatureMap"][Rescale[sz1[\[Delta], g1, g2, k], {-1, 1}]]
] 

Setting ViewPoint -> Top to more clearly show the color variation gives:

p.s., there's a typo in your definition of En1: in the last factor I think you intend to have a space between I and delta.