Analytical solution for ODE with a power-law term?

Question

I want to solve the following differential equation which is a very common growth law model in biology — a generalization of the logistic equation. In this case, the equation possesses a power-law factor 3/4.

$$\frac{dx}{dt}= 4 A k \left(\frac{x(t)}{A}\right)^{3/4}-3 k x(t)$$

The problem is to obtain the analytical solution for a certain initial condition $x(t)=B_0$, where $A$ and $k$ and $B_0$ are positive constants.

I evaluated

DSolve[{x'[t] == -2 k x[t] + 3 A k (x[t]/A)^(2/3), x[0] == B}, x, t]

and obtained four solutions. It is difficult for me to interpret the solutions.

I am interested in solving for an arbitrary initial condition, X(0)=B y A (without giving a particular value)

I get the following message:

Solve::ifun: Inverse functions are being used by Solve, so some solutions may not be found; use Reduce for complete solution information.

How can I solve this differential equation using Mathematica? What more can be done with Mathematica?

Answer 1

To make things a little simpler, we solve for x[t] instead of x, like this

soln = DSolve[{x'[t] == -2 k x[t] + 3 A k (x[t]/A)^(2/3), x[0] == B}, 
   x[t], t];

We obtain 6 possible solutions.

Using Reduce to check the solutions

Let's check the first solution at the initial condition x[0] == B using Reduce

Reduce[(x[t] == B) /. soln[[1]] /. t -> 0, {A, B}, Reals]

$$\left(A<0\land B=\frac{27 A}{8}\right)\lor \left(A>0\land B=\frac{27 A}{8}\right)$$ This says that soln[[1]] satisfies the IC for any any positive $A$ provided $B = 27A/8$. However, with this IC we get x'[0] = 0, the steady-state condition, which leads to the trivial solution $x(t)=27A/8$.

Now let's see if soln[[2]] satisfies the IC for any positive values of A & B:

Reduce[(x[t] == B) /. soln[[2]] /. t -> 0, {A, B}, Reals]

$$ \left(A<0\land \frac{27 A}{8}\leq B<0\right)\lor \left(A>0\land \left(B<0\lor 0<B\leq \frac{27 A}{8}\right)\right) $$

For $0<A$, soln[[2]] satisfies the IC for $0<B\le 27A/8$. For the other 4 solutions Reduce is unable to find real values of A & B that satisfy the IC.

Plotting the real solution

The second solution looks good at t=0. Let's plot its real and imaginary parts like this

Manipulate[ ReImPlot[
  Evaluate[x[t] /. soln[[{2}]] /. k -> kk /. A -> a /. B -> b], 
    {t, 0, 5}, PlotRange -> {All, {-5, 20}}],
 {{a, 3, "A"}, .1, 5, .1},
 {{b, 2, "B"}, 0.01, 15, .01},
 {{kk, 1, "k"}, 0, 5, 1}]

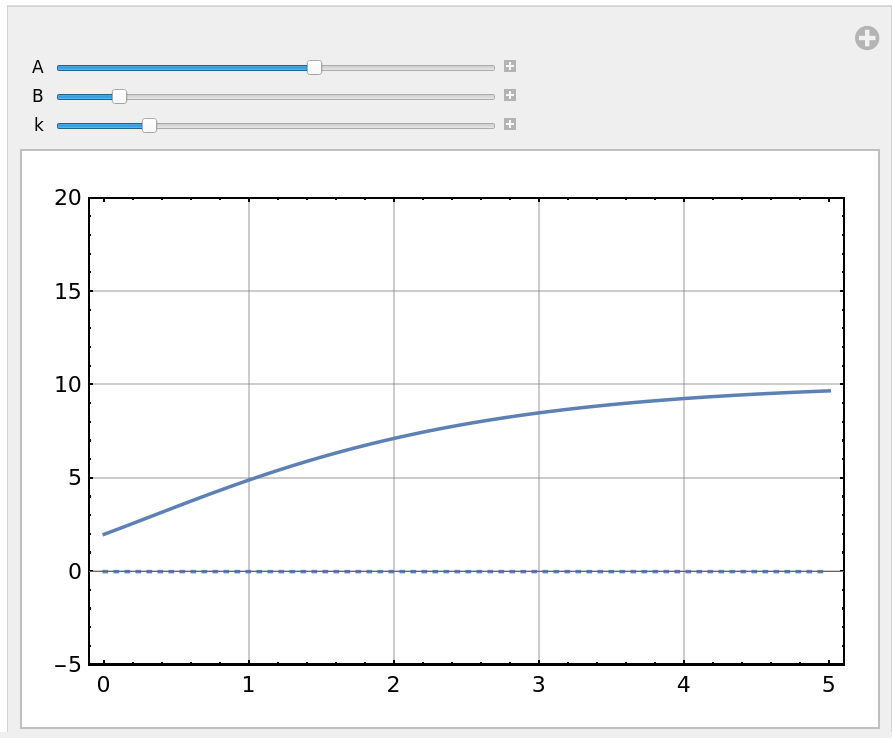
This plot shows the imaginary part of soln[[2]] remains zero for all $t>0$ provided the value of B is sufficiently small.

Plotting the complex solutions

We can use almost the same code to examine the other "solutions". For example, We can replace soln[[{2}]] with soln[[{3,5}]] and adjust the plot range in the above statement to obtain a plot of soln[[3]] and soln[[5]].

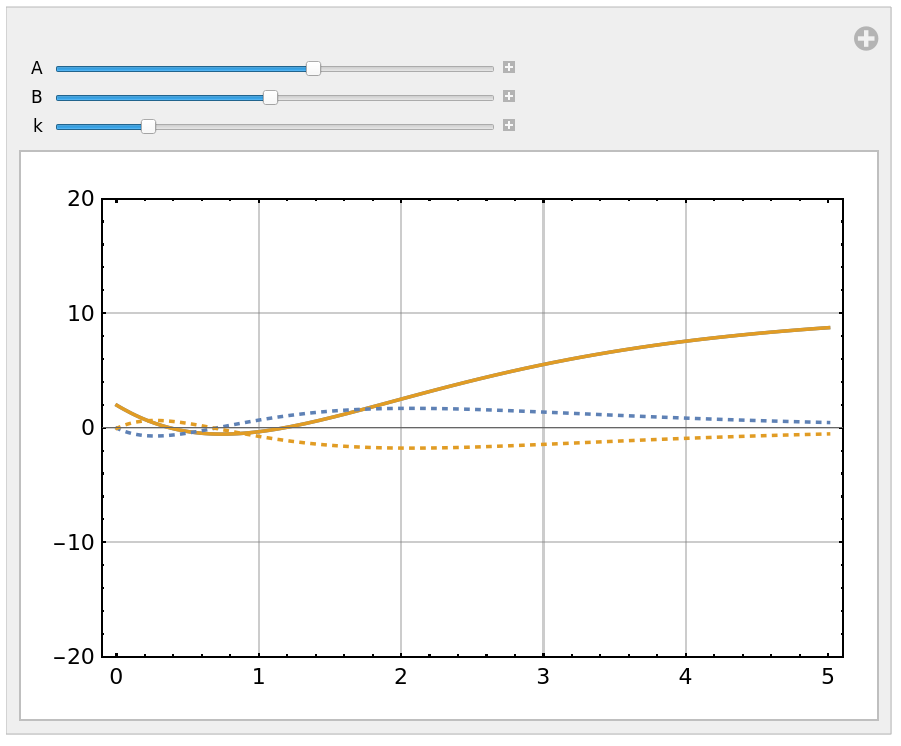
This plot shows those complex conjugate functions have imaginary parts for values of $t>0$.

We conclude that the analytical solution we want is given by soln[[2]] and the conditions $A>0$, $0<B< 27A/8$.