FiniteElement isn't necessary for this problem. The old good TensorProductGrid handles the problem quite well:
system = With[{Ψ = Ψ[x, y, t]},
{D[Ψ, t] == I (Laplacian[Ψ, {x, y}]/2 - ((x^2 + y^2) + Sin[t]^2 (x + y)) Ψ),
Ψ == 0 /. {{x -> -10}, {x -> 10}, {y -> -10}, {y -> 10}},
Ψ == Exp[-1/2 (x^2 + y^2)] /. t -> 0}];
sol = NDSolveValue[system, Ψ, {t, 0, 1}, {x, -10, 10}, {y, -10, 10}];
Plot3D[Abs@sol[x, y, 1], {x, -10, 10}, {y, -10, 10}, PlotRange -> All, PlotPoints -> 50]
NMaximize[Abs[sol[x, y, 1]], {x, y}]
(* {1.4014, {x -> -0.0593488, y -> -0.0593488}} *)
Test passes in v12.1.1.
Futher tests show v9.0.1 and v8.0.4 have difficulty in solving the system with defaullt setting, so this turns out to be another example indicating NDSolve is improved silently these years. Nevertheless, with the magic of Pseudospectral, we can still solve the problem in v8 and v9:
If[$VersionNumber < 9, Laplacian = D[#, x, x] + D[#, y, y] &;
NDSolveValue = #2 /. First@NDSolve[##] &];
mol[n:_Integer|{_Integer..}, o_:"Pseudospectral"] := {"MethodOfLines",
"SpatialDiscretization" -> {"TensorProductGrid", "MaxPoints" -> n,
"MinPoints" -> n, "DifferenceOrder" -> o}}
system = With[{Ψ = Ψ[x, y, t]},
{D[Ψ, t] == I (Laplacian[Ψ, {x, y}]/2 - ((x^2 + y^2) + Sin[t]^2 (x + y)) Ψ),
Ψ == 0 /. {{x -> -10}, {x -> 10}, {y -> -10}, {y -> 10}},
Ψ == Exp[-1/2 (x^2 + y^2)] /. t -> 0}];
sol = NDSolveValue[system, Ψ, {t, 0, 1}, {x, -10, 10}, {y, -10, 10},
Method -> mol[55]]; // AbsoluteTiming
(* v8.0.4: {178.4673377, Null} *)
(* v9.0.1: {40.305892, Null} *)
FindMaximum[Abs@sol[x, y, 1], {x, y}]
(* v8.0.4: {1.38975, {x -> -0.0438577, y -> -0.0438577}} *)
(* v9.0.1: lstol warning, {1.38918, {x -> -0.0439239, y -> -0.043924}} *)
NMaximize isn't used to find the maximum because it spits out a Experimental`NumericalFunction[…] as output in v8 and v9, which is obviously a (now fixed) bug.


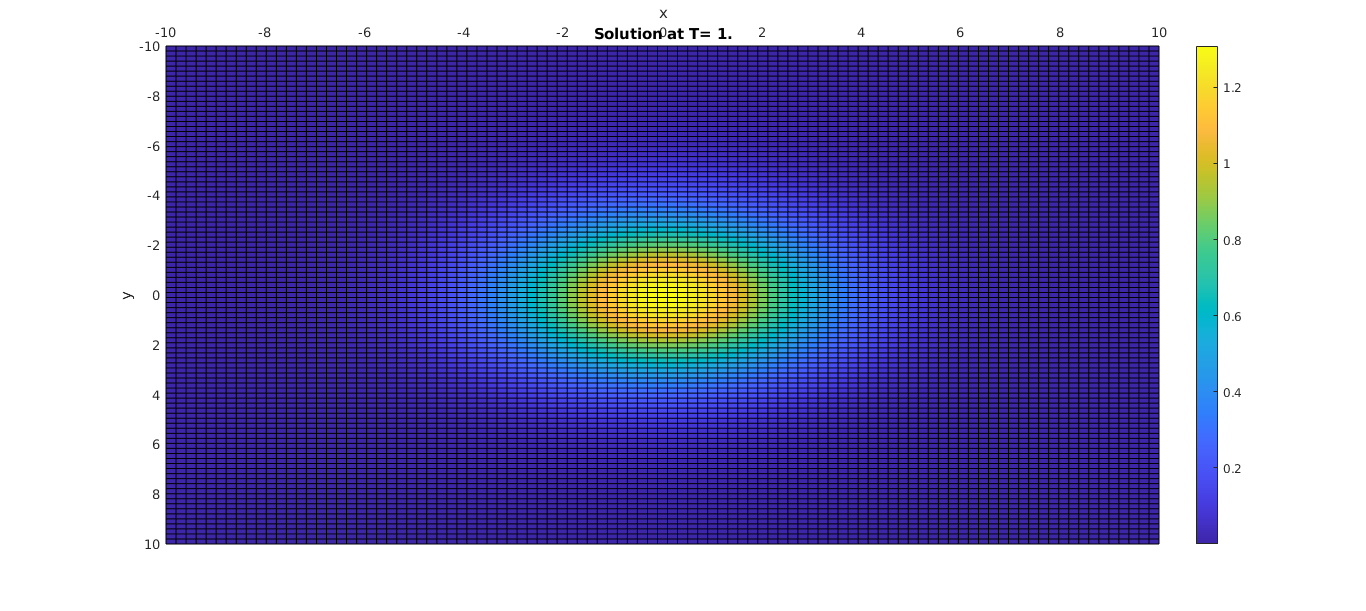
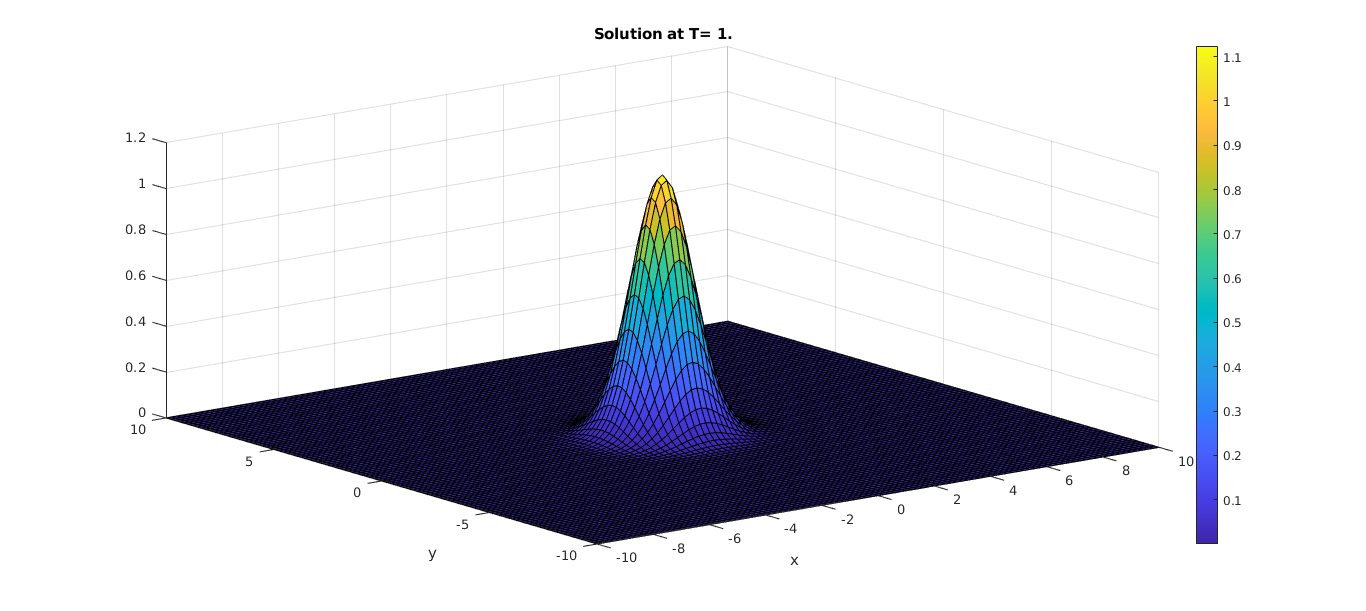
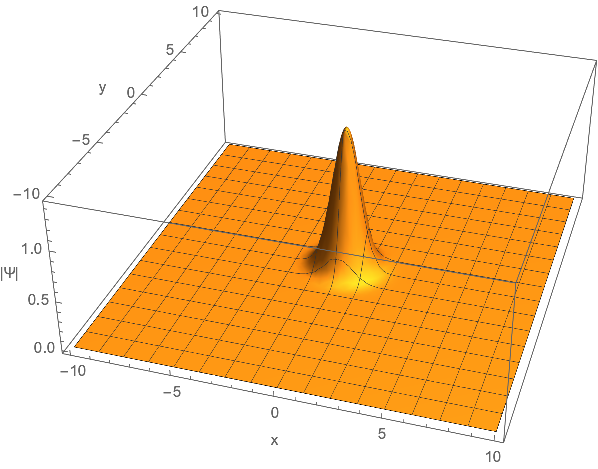
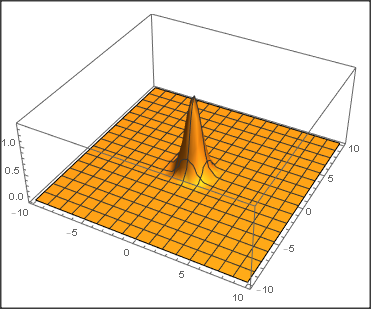
t=1numerical solution with several pikes and message from the system. In this case method of lines is preferable. $\endgroup$