How to numerically verify that principal value?

Question

Mathematica finds

Integrate[Exp[I*s]/(1 + s/(s^2 - 1)^2), {s, -Infinity, Infinity}, PrincipalValue -> True] // ToRadicals
(*A huge closed-form expression which is omitted here.*)
N[%]
(*-1.414 + 0.192275 I*)

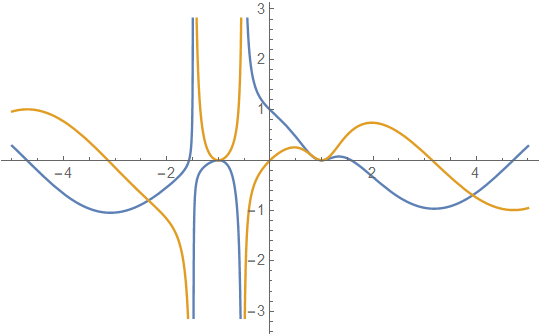
The use of the principal value is grounded by the plots

Plot[{Cos[s]/(1 + s/(s^2 - 1)^2),Sin[s]/(1 + s/(s^2 - 1)^2)},{s,-5,5},WorkingPrecision->30,PlotPoints -> 50]

It's clear that the integrand has its real singularities at the real roots of the denominator, so

sol = Reduce[1 + s/(s^2 - 1)^2 == 0, s, Reals] // ToRadicals;
 sol[[1]][[2]]
(*-(1/(2 Sqrt[3/(4 + (155/2 - (3 Sqrt[849])/2)^(1/3) + (1/2 (155 + 3 Sqrt[849]))^(1/3))]))
 -  1/2 Sqrt[8/3 - 1/3 (155/2 - (3 Sqrt[849])/2)^(1/3) -  1/3 (1/2 (155 + 3 Sqrt[849]))^(1/3) + 
   2 Sqrt[3/( 4 + (155/2 - (3 Sqrt[849])/2)^(1/3) + (1/2 (155 + 3 Sqrt[849]))^(  1/3))]]*)
 N[%]
(*-1.49022*) 
sol[[2]][[2]]
(*-(1/(2 Sqrt[3/(4 + (155/2 - (3 Sqrt[849])/2)^(1/3) + (1/2 (155 + 3 Sqrt[849]))^(1/3))])) +
 1/2 Sqrt[8/3 - 1/3 (155/2 - (3 Sqrt[849])/2)^(1/3) - 
 1/3 (1/2 (155 + 3 Sqrt[849]))^(1/3) + 2 Sqrt[3/( 4 + (155/2 - (3 Sqrt[849])/2)^(1/3)+(1/2 (155 + 3 Sqrt[849]))^( 1/3))]]*)

However, I have doubts concerning the obtained principal value because the integrand asymptotically equals $\exp(is)$ as $s\to \infty$ and $s\to -\infty$ and $$PV\int_{-\infty}^\infty \exp(is)\,ds $$ does not exist.

In view of it I try to verify it numerically through

NIntegrate[Exp[I*s]/(1+s/(s^2-1)^2),{s,-Infinity,
-(1/(2 Sqrt[3/(4+(155/2-(3 Sqrt[849])/2)^(1/3)+(1/2 (155+3 Sqrt[849]))^(1/3))]))-
1/2 Sqrt[8/3-1/3 (155/2-(3 Sqrt[849])/2)^(1/3)-1/3 (1/2 (155+3 Sqrt[849]))^(1/3)+
2 Sqrt[3/(4+(155/2-(3 Sqrt[849])/2)^(1/3)+(1/2 (155+3 Sqrt[849]))^(1/3))]],
-(1/(2 Sqrt[3/(4+(155/2-(3 Sqrt[849])/2)^(1/3)+(1/2 (155+3 Sqrt[849]))^(1/3))]))+
1/2 Sqrt[8/3-1/3 (155/2-(3 Sqrt[849])/2)^(1/3)-1/3 (1/2 (155+3 Sqrt[849]))^(1/3)+
2 Sqrt[3/(4+(155/2-(3 Sqrt[849])/2)^(1/3)+(1/2 (155+3 Sqrt[849]))^(1/3))]],Infinity},
Method->"PrincipalValue",AccuracyGoal->3,PrecisionGoal->3,WorkingPrecision->50]

which results in the error message

NIntegrate::ncvb: NIntegrate failed to converge to prescribed accuracy after 9 recursive bisections in s near {s} = {3.7749613270651398879039428756113970426387939277790*10^28}. NIntegrate obtained 8.8211977939280824575415993952100374290963331174834*10^47 I and 9.1940327832901306869987159913883594088789773626283`50.*^47 for the integral and error estimates.

and

 (*-2.6098684408162971553635553440779848277629513026488*10^49 + 
 8.8211977939280824575415993952100374290963331174789*10^47 I*)

Constructive suggestions are welcome.

Addition. @b.gates.you.know.what in her/his answer suggests to use FourierTransform to this end. This is not it. Here is an example:

Limit[Integrate[Exp[I*t], {t, -a, a}], a -> Infinity]
(*Indeterminate*)

but

FourierTransform[1, y, t] /. t -> 1

(*0*)

-

Answer 1

As noted by the user Mariusz Iwaniuk one could use:

den[s_] = 1 + s/(s^2 - 1)^2;
f[s_] = Exp[I s] / den[s];
integ = Sqrt[2 Pi] Limit[FourierTransform[1/den[s], s, k], k -> 1];
integ // N
(* -0.998307 - 0.313707 I *)

As a check one could try using residues:

zeros = Solve[den[s] == 0 + I 0, s];
residues = Residue[f[s], {s, #}] & /@ zeros[[All, 1, 2]];

2 Pi I (1/2 residues[[1]] + 1/2 residues[[2]] + residues[[4]]) // N
(* -0.998307 - 0.313707 I *)

Answer 2

Following the advice by @b3m2a1, I present my answer. Let us substract Exp[I*s] from the integrand. Then

a = Integrate[Exp[I*s]/(1 + s/(s^2 - 1)^2) - Exp[I*s], {s, -Infinity, Infinity},
PrincipalValue -> True] // ToRadicals;
N[a,30]
(*-1.41400018802696985500835186883 + 0.19227531358741820427339218093 I*)

is in discordance with

NIntegrate[Exp[I*s]/(1 + s/(s^2 - 1)^2) - Exp[I*s],{s, -Infinity,
-(1/(2 Sqrt[3/( 4 + (155/2 - (3 Sqrt[849])/2)^(1/3) + (1/2 (155 + 3 Sqrt[849]))^(1/3))])) - 
 1/2 \[Sqrt](8/3 - 1/3 (155/2 - (3 Sqrt[849])/2)^(1/3) - 
 1/3 (1/2 (155 + 3 Sqrt[849]))^(1/3) + 
 2 Sqrt[3/(      4 + (155/2 - (3 Sqrt[849])/2)^(
   1/3) + (1/2 (155 + 3 Sqrt[849]))^(1/3))]),
-(1/(2 Sqrt[3/(   4 + (155/2 - (3 Sqrt[849])/2)^(1/3) + (1/2 (155 + 3 Sqrt[849]))^(
1/3))])) + 1/2 \[Sqrt](8/3 - 1/3 (155/2 - (3 Sqrt[849])/2)^(1/3) - 
 1/3 (1/2 (155 + 3 Sqrt[849]))^(1/3) + 
 2 Sqrt[3/(4 + (155/2 - (3 Sqrt[849])/2)^(
   1/3) + (1/2 (155 + 3 Sqrt[849]))^(1/3))]),Infinity},
Method -> "PrincipalValue", WorkingPrecision -> 40, AccuracyGoal -> 7, PrecisionGoal -> 7]
(*-0.9983073697207267431817361195580732660872 - .313706787296245329692113892146598026371 I*)

Therefore, at least one of the above results in not correct. However, the latter result is in accordance with the modified approach by @b.gates.you.know.what :

den[s_] = 1 + s/(s^2 - 1)^2;
f[s_] = Exp[I s]/den[s] - Exp[I*s];
integ = Sqrt[2 Pi] Limit[FourierTransform[1/den[s] - 1, s, k], k -> 1];
integ // N
(*-0.998307 - 0.313707 I*)

The above result can be grounded by the calculation with the residues (Here the Jordan's lemma is applicable: pay your attention to -Exp[I*s] in the definition of f[s_].).

Conclusion: (i) Because $$PV\int_{-\infty}^\infty \exp(is)\,ds $$ does not exist, the principal value of the integral under consideration in the question does not exist too. (ii) The symbolic result produced by the Integrate command with the PrincipalValue->True option is not correct.