I want to fit derived distribution on real data and I need MLE parameters, standard errors of the estimated parameters, AIC, Loglikelihood, Goodness of fit test (Chi-square, Anderson etc), PDF, CDF, Plots and QQplots. The data,CDF and PDF are
data = {3.70, 2.74, 2.73, 2.50, 3.60, 3.11, 3.27, 2.87, 1.47, 3.11,
4.42, 2.41, 3.19, 3.22, 1.69, 3.28, 3.09, 1.87, 3.15, 4.90, 3.75,
2.43, 2.95, 2.97, 3.39, 2.96, 2.53, 2.67, 2.93, 3.22, 3.39, 2.81,
4.20, 3.33, 2.55, 3.31, 3.31, 2.85, 2.56, 3.56, 3.15, 2.35, 2.55,
2.59, 2.38, 2.81, 2.77, 2.17, 2.83, 1.92, 1.41, 3.68, 2.97, 1.36,
0.98, 2.76, 4.91, 3.68, 1.84, 1.59, 3.19, 1.57, 0.81, 5.56, 1.73,
1.59, 2.00, 1.22, 1.12, 1.71, 2.17, 1.17, 5.08, 2.48, 1.18, 3.51,
2.17, 1.69, 1.25, 4.38, 1.84, 0.39, 3.68, 2.48, 0.85, 1.61, 2.79,
4.70, 2.03, 1.80, 1.57, 1.08, 2.03, 1.61, 2.12, 1.89, 2.88, 2.82,
2.05, 3.65};
cdf = (1 - (1 + ((1 - (1 + x^ξ)^-ψ)^λ/(1 - (1 - (1 + x^ξ)^-ψ)^λ))^γ)^-α)^(β)
pdf = D[cdf, x];
\[ScriptCapitalD] = ProbabilityDistribution[pdf, {x, 0, Infinity},
Assumptions -> α > 0 && β > 0 && γ > 0 && λ > 0 && ψ > 0 && ξ > 0];


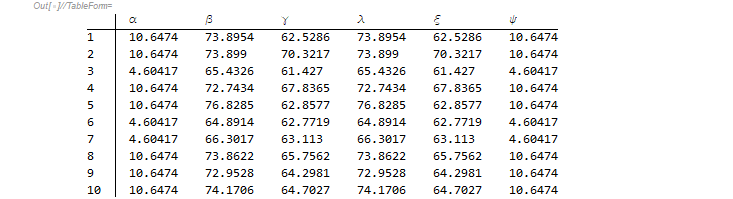
FullSimplifyyour cdf can be simplified to $\left(1-\left(\left(\frac{1}{\left(1-\left(x^{\xi }+1\right)^{-\psi }\right)^{-\lambda }-1}\right)^{\gamma }+1\right)^{-\alpha }\right)^{\beta }$. $\endgroup$