I am trying to plot a function over a tirangular region
F[x_, y_] := -(0.1/x)*(Log[(x - y)/0.1])
Plot3D[F[x, y] , {x, 0.1, 3}, {y, 0, 5},
RegionFunction -> Function[{x, y}, x > y],
PlotRange -> {-2, 1}, AxesLabel -> Automatic, Mesh -> 60]
and I get some a jagged surface on one boundary.
I appreciate the surface is very steep on the $x=y$ line, and it could pose numerical difficulties.
Yet, is there a workaround to obtain a more aesthetically pleasing plot?
Thanks

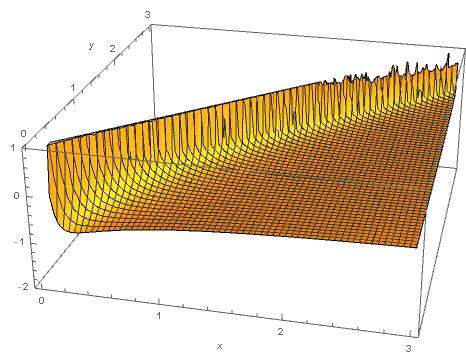
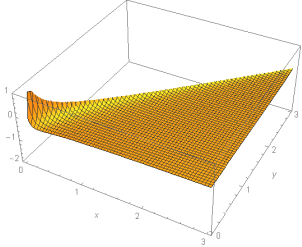
PlotPoints -> 100might help. $\endgroup$