8
$\begingroup$

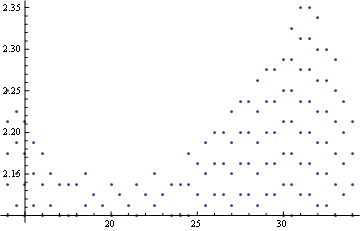
I want to create ListContourPlots for sets of Data (from a physics simulation) given as Triplets {x, y, f(x, y)}. Certain parameter regions in the x-y-plane are excluded by experiments. The list of triplets simply does not contain any Triplets with excluded x-y-parameters. ListPointPlot3D shows that this is not the problem.

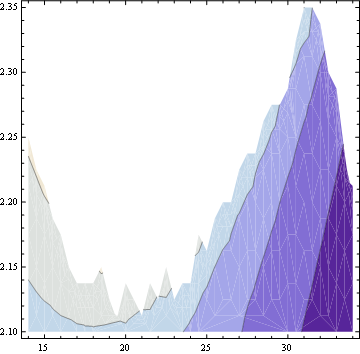
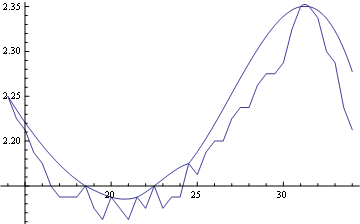
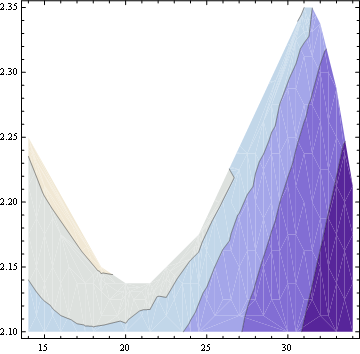
The problem is that ListContourPlot (which I prefer to use, since I have to correlate exclusions from different quantities) interpolates values into regions where no data points are given, as soon as the shape of the data-filled region becomes concave.

I already tried creating a list that is only defined in the excluded regions and painting it over the original plot, however, my dataset is not entirely concave, so that overpainting will cut off some of the interesting parts of the plot...

I figure it has something to do with the "Method" option of ListContourPlot, but those things are really badly documented.

Here is a list of 140 data triplets that show the problem.

l= {{14., 2.1, 125.433}, {14., 2.1375, 125.957}, {14., 2.175, 
  126.58}, {14., 2.2125, 127.354}, {14., 2.25, 128.415}, {14.5, 
  2.1125, 125.698}, {14.5, 2.15, 126.315}, {14.5, 2.1875, 
  127.087}, {14.5, 2.225, 128.152}, {15., 2.1, 125.593}, {15., 2.1375,
   126.238}, {15., 2.175, 127.061}, {15., 2.2125, 128.256}, {15.5, 
  2.1125, 125.886}, {15.5, 2.15, 126.669}, {15.5, 2.1875, 
  127.783}, {16., 2.1, 125.73}, {16., 2.1375, 126.534}, {16., 2.175, 
  127.708}, {16.5, 2.1125, 126.054}, {16.5, 2.15, 127.082}, {17., 2.1,
   125.829}, {17., 2.1375, 126.85}, {17.5, 2.1, 125.858}, {17.5, 
  2.1375, 127.016}, {18., 2.1, 125.868}, {18., 2.1375, 
  127.185}, {18.5, 2.1125, 126.243}, {18.5, 2.15, 128.277}, {19., 
  2.125, 126.76}, {19.5, 2.1125, 126.183}, {20., 2.1, 125.633}, {20., 
  2.1375, 127.686}, {20.5, 2.125, 126.573}, {21., 2.1125, 
  125.763}, {21.5, 2.1, 125.093}, {21.5, 2.1375, 127.062}, {22., 
  2.125, 125.806}, {22.5, 2.1125, 124.979}, {22.5, 2.15, 
  127.707}, {23., 2.125, 125.111}, {23.5, 2.1, 124.03}, {23.5, 2.1375,
   125.248}, {24., 2.1, 123.738}, {24., 2.1375, 124.82}, {24.5, 2.1, 
  123.445}, {24.5, 2.1375, 124.411}, {24.5, 2.175, 126.896}, {25., 
  2.125, 123.689}, {25., 2.1625, 124.924}, {25.5, 2.1125, 
  123.092}, {25.5, 2.15, 123.987}, {25.5, 2.1875, 125.801}, {26., 
  2.125, 123.022}, {26., 2.1625, 123.937}, {26., 2.2, 125.892}, {26.5,
   2.125, 122.703}, {26.5, 2.1625, 123.512}, {26.5, 2.2, 
  124.901}, {27., 2.1125, 122.198}, {27., 2.15, 122.848}, {27., 
  2.1875, 123.782}, {27., 2.225, 125.874}, {27.5, 2.125, 
  122.095}, {27.5, 2.1625, 122.745}, {27.5, 2.2, 123.676}, {27.5, 
  2.2375, 125.734}, {28., 2.125, 121.808}, {28., 2.1625, 
  122.394}, {28., 2.2, 123.198}, {28., 2.2375, 124.563}, {28.5, 
  2.1125, 121.39}, {28.5, 2.15, 121.87}, {28.5, 2.1875, 
  122.506}, {28.5, 2.225, 123.412}, {28.5, 2.2625, 125.253}, {29., 
  2.125, 121.29}, {29., 2.1625, 121.747}, {29., 2.2, 122.372}, {29., 
  2.2375, 123.256}, {29., 2.275, 124.961}, {29.5, 2.125, 
  121.036}, {29.5, 2.1625, 121.454}, {29.5, 2.2, 122.006}, {29.5, 
  2.2375, 122.762}, {29.5, 2.275, 123.966}, {30., 2.1, 120.465}, {30.,
   2.1375, 120.896}, {30., 2.175, 121.334}, {30., 2.2125, 
  121.862}, {30., 2.25, 122.595}, {30., 2.2875, 123.735}, {30.5, 2.1, 
  120.187}, {30.5, 2.1375, 120.599}, {30.5, 2.175, 121.065}, {30.5, 
  2.2125, 121.525}, {30.5, 2.25, 122.157}, {30.5, 2.2875, 
  123.07}, {30.5, 2.325, 124.9}, {31., 2.125, 120.167}, {31., 2.1625, 
  120.593}, {31., 2.2, 121.078}, {31., 2.2375, 121.561}, {31., 2.275, 
  122.237}, {31., 2.3125, 123.247}, {31., 2.35, 126.014}, {31.5, 
  2.125, 119.881}, {31.5, 2.1625, 120.285}, {31.5, 2.2, 
  120.747}, {31.5, 2.2375, 121.268}, {31.5, 2.275, 121.808}, {31.5, 
  2.3125, 122.628}, {31.5, 2.35, 124.034}, {32., 2.1125, 
  119.484}, {32., 2.15, 119.849}, {32., 2.1875, 120.264}, {32., 2.225,
   120.742}, {32., 2.2625, 121.268}, {32., 2.3, 121.85}, {32., 2.3375,
   122.72}, {32.5, 2.1125, 119.213}, {32.5, 2.15, 119.558}, {32.5, 
  2.1875, 119.949}, {32.5, 2.225, 120.396}, {32.5, 2.2625, 
  120.911}, {32.5, 2.3, 121.431}, {33., 2.1, 118.846}, {33., 2.1375, 
  119.16}, {33., 2.175, 119.512}, {33., 2.2125, 119.91}, {33., 2.25, 
  120.365}, {33., 2.2875, 120.871}, {33.5, 2.125, 118.783}, {33.5, 
  2.1625, 119.101}, {33.5, 2.2, 119.458}, {33.5, 2.2375, 
  119.856}, {34., 2.1, 118.333}, {34., 2.1375, 118.614}, {34., 2.175, 
  118.923}, {34., 2.2125, 119.261}};

ListPlot@l[[All, 1 ;; 2]]

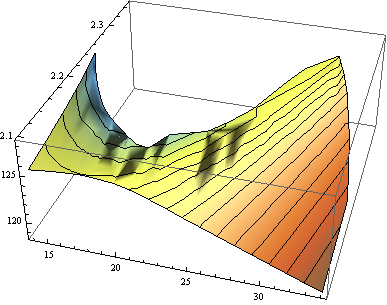
Mathematica graphics

Improve this question
$\endgroup$
2
  • 1
    $\begingroup$ "interpolates values into regions where no data points are given". I think you need to thing a bit about what the mathematical difference is between the regions you want to interpolate and those you do not. If interpolate should not interpolate any regions enclosed by points, then it shouldn't do any interpolation at all. $\endgroup$
    – jVincent
    Commented Feb 21, 2013 at 8:40
  • 1
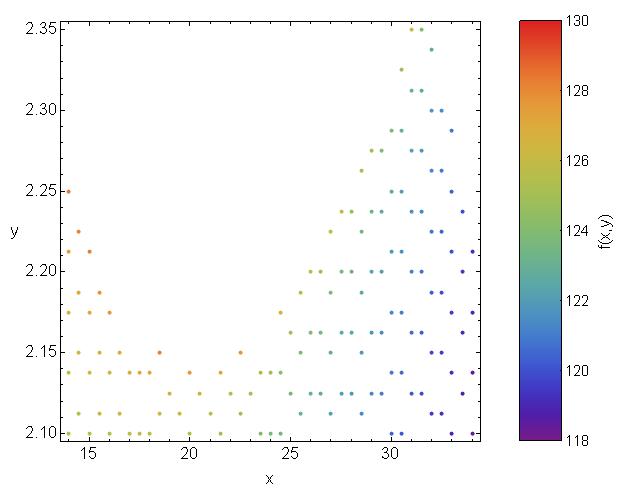
    $\begingroup$ @jVincent Exactly. I think InterpolationOrder->0 is the way to go. @Neuneck If you provide some code for the plot and an image ilustrating the problem (instead of a "spoiler"), you are bound to get better answers. $\endgroup$
    – Ajasja
    Commented Feb 21, 2013 at 8:46

2 Answers 2

Reset to default
12
$\begingroup$ 
f = Interpolation[l3= (Last /@ Sort /@ GatherBy[l[[All, 1 ;; 2]], #[[1]] &])]
ListContourPlot[l, RegionFunction -> (#2 < f[#1] &)]

Mathematica graphics

Edit

If you want a smoother curve, you could use for example whuber's method here for getting something similar to an "envolvent" curve:

l4 = {(#[[1]] - 14) 2 + 1, #[[2]]} & /@ l3;
nrow = 32;
i = Image[SparseArray[Flatten[Table[{i, IntegerPart@#1} -> #2, {i, 1, nrow}] & @@@ l4]]] 
                                                                                   // ImageAdjust

Mathematica graphics

{minima, maxima} = Flatten[Position[First[ImageData[#[i]]], 1]] & /@ {MinDetect, MaxDetect};

f1 = Interpolation[N@{(#[[1]] - 1)/2 + 14, #[[2]]} & /@ l4[[maxima]], InterpolationOrder -> 1]

f2[x_] = Max[f[x], f1[x]]

The "envolvent":

Quiet@Show[ListLinePlot@l3, Plot[f2[x], {x, 14, 34}]]

Mathematica graphics

Quiet@ListContourPlot[l, RegionFunction -> (#2 < f2[#1] &)]

Mathematica graphics

Quiet@ListPlot3D[l, RegionFunction -> (#2 < f2[#1] &), 
                 ColorFunction -> "SouthwestColors", MeshFunctions -> {#3 &}]

Mathematica graphics

Improve this answer
$\endgroup$
1
  • $\begingroup$ Thank you, awesome answer and solved my problem! $\endgroup$
    – Neuneck
    Commented Feb 21, 2013 at 11:55
3
$\begingroup$

First create a list data data= and put inside the list containing the points. Then, use the following code in order to produce what you want

Clear[colorbar]
colorbar[{min_, max_}, colorFunction_: Automatic, divs_: 150] := 
DensityPlot[y, {x, 0, 0.1}, {y, min, max}, AspectRatio -> 10, 
PlotRangePadding -> 0, PlotPoints -> {2, divs}, MaxRecursion -> 0, 
Frame -> True, FrameLabel -> {{None, "f(x,y)"}, {None, None}}, 
LabelStyle -> Directive[FontFamily -> "Helvetica", 17], 
FrameTicks -> {{None, All}, {None, None}}, 
FrameTicksStyle -> Directive[FontFamily -> "Helvetica", 15, Plain], 
ColorFunction -> colorFunction]

With[{opts = {ImageSize -> {Automatic, 500}}, cf = "Rainbow"}, 
Row[{Show[
ListPlot[List /@ data[[All, {1, 2}]], 
 PlotStyle -> ({PointSize[0.01], ColorData[cf][#1]} & /@ 
    Rescale[data[[All, 3]], {118, 130}]), PlotRange -> All, 
 AspectRatio -> 1, Frame -> True, RotateLabel -> False, 
 Axes -> None, FrameTicks -> True, FrameLabel -> {"x", "y"}, 
 LabelStyle -> Directive[FontFamily -> "Helvetica", 17], 
 ImagePadding -> {{60, 20}, {60, 20}}, opts]], 
 Show[colorbar[{118, 130}, cf], 
ImagePadding -> {{20, 60}, {60, 20}}, opts]}]]

Here is my output using Mathematica 9.0 under WinXP.

enter image description here

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.