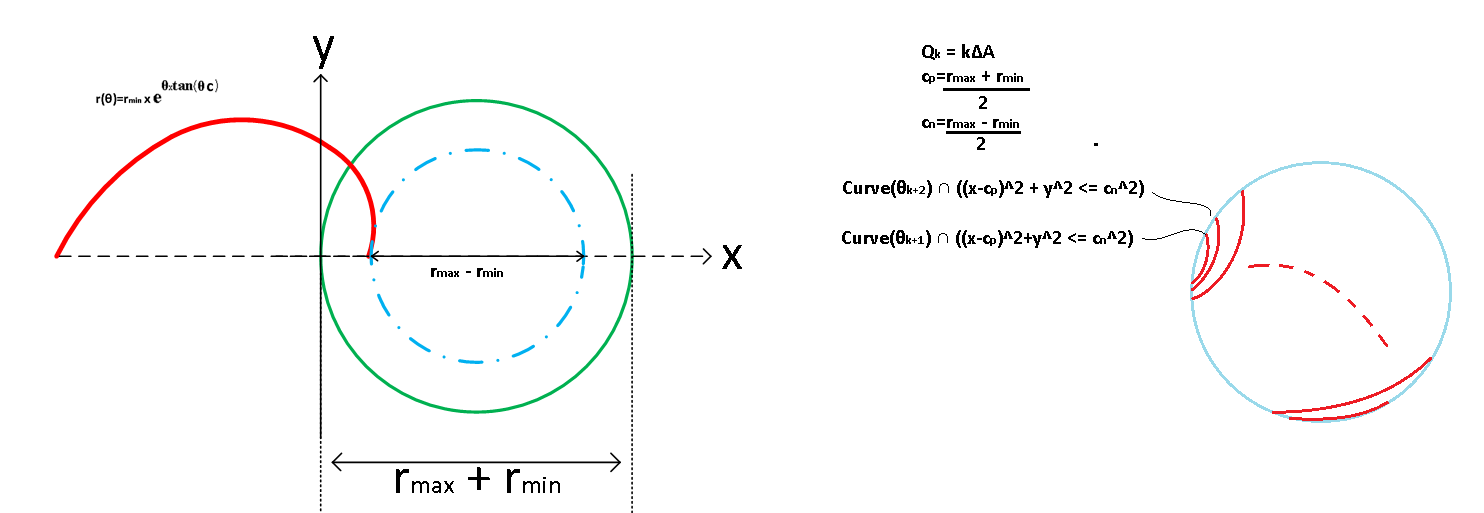
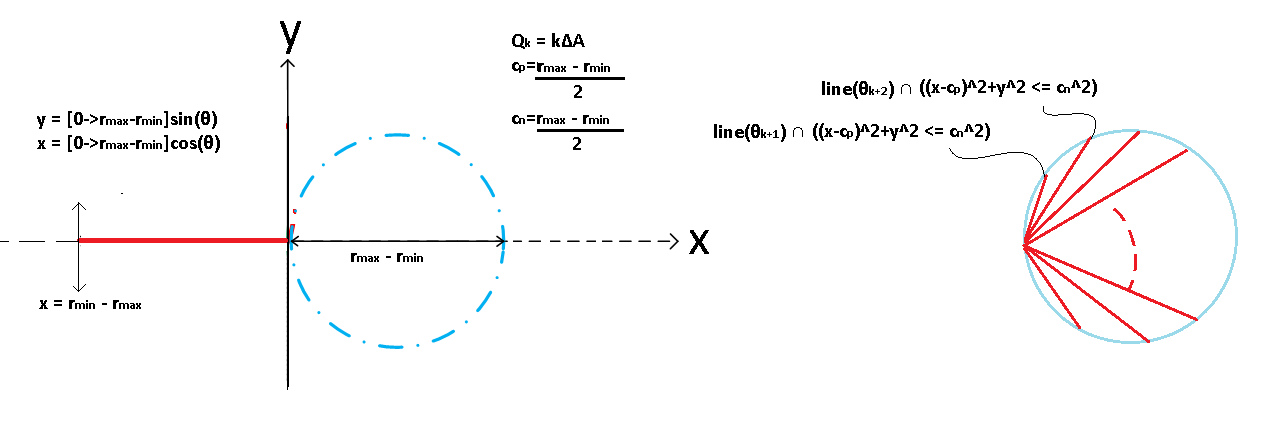
I need to prove that for a full turn of a logarithmic spiral (independent from the constant tangent of it) and a straight line on the z axis, the logarithmic spiral always covers more space-time in the circle than the straight line.
I need to prove that;
$$ \frac{ \int_{0}^{2\pi} curve(\Theta ) \bigcap ((x-\frac{r_{max}+r_{min}}{2})^{2}+y^{2}\leq (\frac{r_{max}-r_{min}}{2})^{2}) d\theta}{length of the spiral} > \frac{\int_{0}^{2\pi} line(\Theta ) \bigcap ((x-\frac{r_{max}-r_{min}}{2})^{2}+y^{2}\leq (\frac{r_{max}-r_{min}}{2})^{2}) d\theta}{length of the line}$$
I don't know where to start here. When the spiral rotates around the z axis in clockwise you will see that it will intersect with the circle as on the right. For example, the first intersection is demonstrated when θk+1 and the second is θk+2 vs. as shown in the right figure. If we assume θk = k x ∆A I am asking for the sum of those pieces of the spiral that is in the circle when ∆A→0. That means -I guess- some king of integral but the limits of are changing with the angle. I hope I made myself clear.
How can I get this on wolfram?