I'm attempting to add gaussian white noise into a single equation of a 2 state variable dynamical system $$\frac{dx(t)}{dt}=1-x(t)\left(1+e^{-y(t)}\right)$$ $$\frac{dy(t)}{dt}=1-y(t)\left(1+e^{\frac{x(t)}{y(t)}}\right)$$
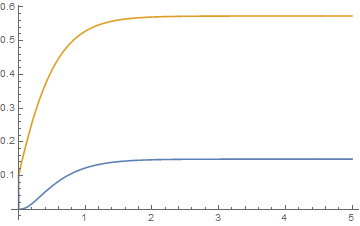
Here is the solution for initial conditions of $(x_0,y_0)=(0.1,0.1)$
q[x_, y_] := 1 - x (1 + Exp[1/y ]);
p[x_, y_] := 1 - y (1 + y Exp[x/ y]);
sol = NDSolveValue[{x'[t] == q[x[t], y[t]], y'[t] == p[x[t], y[t]],
x[0] == 0.1, y[0] == 0.1}, {x[t], y[t]}, {t, 0, 5}];
Plot[{sol[[1]], sol[[2]]}, {t, 0, 5}]
I now add gaussian white noise to the first equation, and wish to solve $$dx = \left(1-x(t)\left(1+e^{-y(t)}\right)\right)dt+\sigma dW(t)$$ $$dy=\left(1-y(t)\left(1+e^{\frac{x(t)}{y(t)}}\right)\right)dt$$ $$dW(t) = \eta(t)\sqrt{dt}$$
where $\eta(t)$ is an uncorrelated white noise.
\[Sigma] = 0.1;
sol2 = RandomFunction[ItoProcess[{
\[DifferentialD]x[t] ==
1 - x[t] (1 + Exp[1/y [t]]) + \[Sigma] \[DifferentialD]w[t],
\[DifferentialD]y[t] == 1 - y[t] (1 - y[t] Exp[x[t]/ y[t]])}, {x[
t], y[t]}, {{x, y}, {0.1, 0.1}}, t,
w \[Distributed] WienerProcess[]], {0, 5, 0.01}];
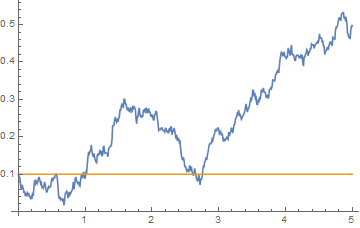
ListLinePlot[sol2]
but I get results that seems independent of the deterministic solution itself
I would expect noise fluctuating around the deterministic solution of both state variables. In fact when I take \[Sigma]=0 I get a flat line of the initial condition, while I expect the deterministic solution itself.
Obviously I'm doing something wrong here. Since this is an over simplification of a larger problem I'm working on, I will appreciate if you could point along your answer what is the best way in your opinion to treat SDE's in mathematica.

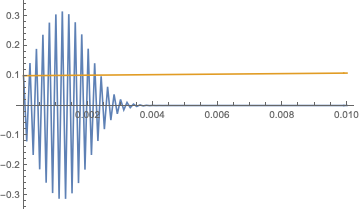
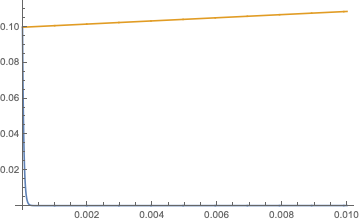
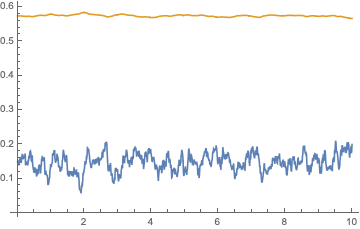
sol2seem to be different than the expressions above. $\endgroup$