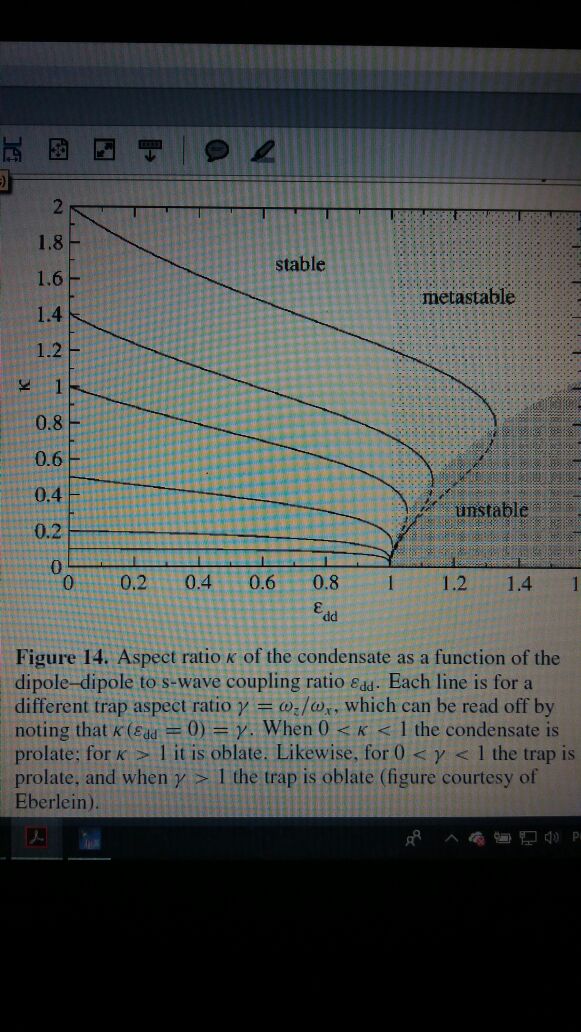
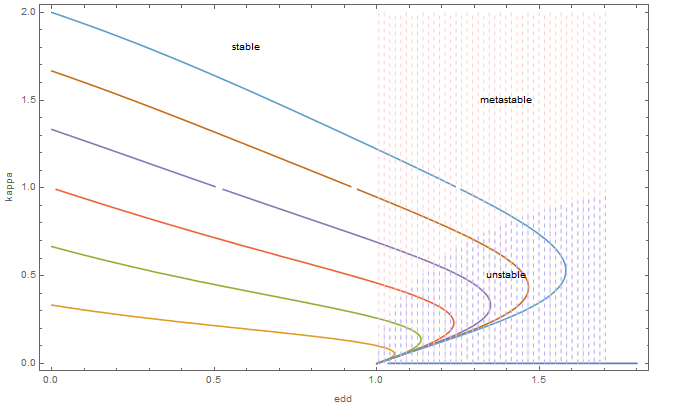
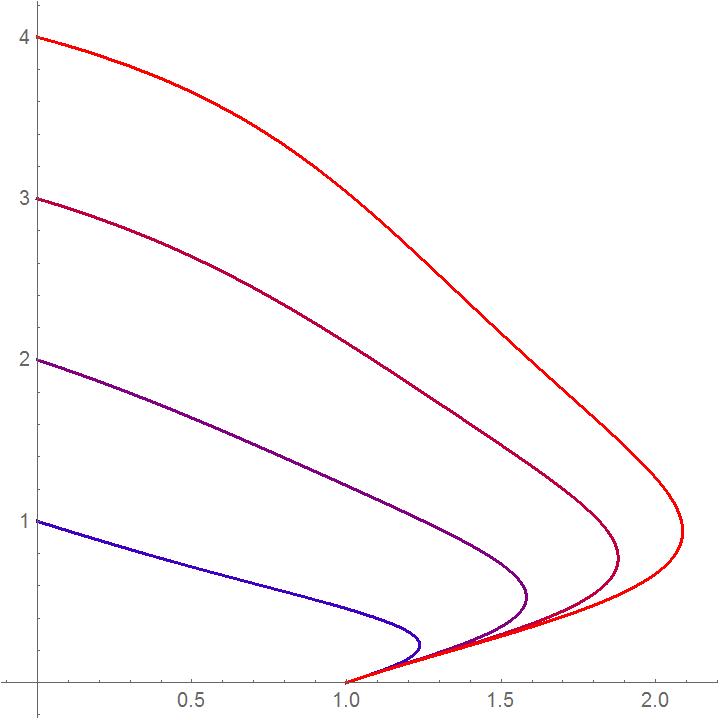
How can I plot this transcedental equation?

Question

How can I plot $\kappa(\epsilon_{dd},\lambda)$ this transcendental equation? $$3\kappa^2 \epsilon_{dd}\left[\left(\frac{\lambda^2}{2}+1\right)\frac{f_s(\kappa)}{1-\kappa^2}-1\right]+(\epsilon_{dd}-1)(\kappa^2-\lambda^2)=0 $$ where $\lambda=1,2,3,4$ and $$f_s(\kappa)=\frac{1+2\kappa^2}{1-\kappa^2}-\frac{3\kappa^2 artanh \sqrt{1-\kappa^2} }{(1-\kappa^2)^{3/2}}. $$

My original problem is not that, but it's similar. If you help me with this, maybe I can solve mine.

Here are the codes of equations:

fs[kappa_] := (1 +2 kappa^2)/(1 - kappa^2) - (3 kappa^2 ArcTanh[
  Sqrt[1 - kappa^2]])/(1 - kappa^2)^(3/2)

3 kappa^2 edd (((lambda^2/2) -1 ) fs[kappa]/(1 - kappa^2) - 
 1) + (edd - 1) (kappa^2 - lambda^2) == 0

Answer 1

Use ContourPlot.

fs[kappa_] := (1 + 2 kappa^2)/(1 - 
 kappa^2) - (3 kappa^2 ArcTanh[Sqrt[1 - kappa^2]])/(1 - 
  kappa^2)^(3/2)

zero[kappa_, edd_, lambda_] = 
3 kappa edd (((lambda^2/2) + 1) fs[kappa]/(1 - kappa^2) - 
  1) + (edd - 1) (kappa^2 - lambda^2);

Show[{ContourPlot[
Evaluate@
Table[zero[kappa, edd, lambda] == 0, {lambda, 0, 2, 1/3}], {edd, 
0, 1.8}, {kappa, 0, 2}, FrameLabel -> Automatic, 
AspectRatio -> 6/10], 
ContourPlot[edd + 1, {edd, 0, 1.8}, {kappa, 0, 2}, 
FrameLabel -> Automatic, AspectRatio -> 6/10, 
RegionFunction -> Function[{x, y, z}, 1 < x < 2], 
ContourStyle -> {Directive[Lighter[Red, 0.8], Dashed]}, 
Contours -> 100, ContourShading -> None], 
ContourPlot[edd + 1, {edd, 0, 1.8}, {kappa, 0, 2}, 
FrameLabel -> Automatic, AspectRatio -> 6/10, 
RegionFunction -> Function[{x, y, z}, (x - 2)^2 + (y)^2 < 1], 
ContourStyle -> {Directive[Lighter[Blue, 0.7], Dashed]}, 
Contours -> 100, ContourShading -> None]}, 
Epilog -> {Text[unstable, {1.4, 0.5}], Text[metastable, {1.4, 1.5}], 
Text[stable, {0.6, 1.8}]}]

Answer 2

You consider four equations[lamda=1,2,3,4] in epsdd and kappa. It is very easy to solve for epsdd=f[kappa;lamda]. The four solutions can be plotted for different lamda with

ParametricPlot[{f[kappa;lamda],kappa},{kappa,...}]

if you know the kappa-range!

solution(with MMA-code offered) and the corrected formulas:

fs[kappa_] := (1 + 2 kappa^2)/(1 -kappa^2) - (3 kappa^2 ArcTanh[Sqrt[1 - kappa^2]])/(1 -kappa^2)^(3/2)
gl = 3 kappa edd (((lambda^2/2) + 1) fs[kappa]/(1 - kappa^2) -1) + (edd - 1) (kappa^2 - lambda^2) == 0

ergedd =  Solve[gl, edd][[1]] (* implicit soulution *)
(* {edd -> (kappa^2 - lambda^2)/(kappa^2 - lambda^2 + 
3 kappa (-1 + ((1 + lambda^2/2) ((1 + 2 kappa^2)/(1 - kappa^2) - 
(3 kappa^2 ArcTanh[Sqrt[1 - kappa^2]])/(1 - kappa^2)^(3/2)))/(1-kappa^2)))} *)


Show[Table[
ParametricPlot[  {edd /. ergedd, kappa} , {kappa, 0, lambda}, 
PlotStyle -> RGBColor[lambda/4, 0, 1 - lambda/4]]  , {lambda, 1, 
4}]
, AspectRatio -> 1 ,PlotRange->{0,4}]

the result is as expected: